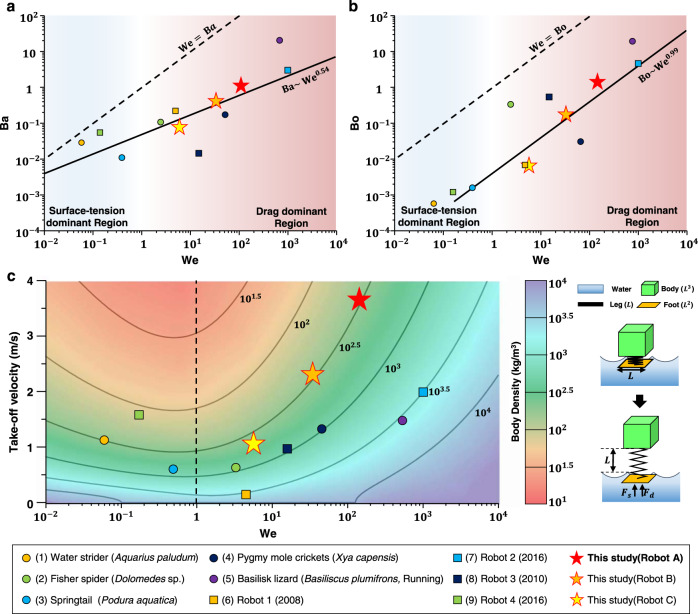
Fig. 1. The trend of the Baudoin (Ba), Bond (Bo), and take-off velocity of jumping animals and robots depending on Weber (We) number.

a, b Baudoin (Ba), Weber (We), and Bond (Bo) number for insects and robots jumping on water. Bold lines indicate the dependence of the best-fit line (Ba = 0.0496 We^0.54, r² = 0.597, Bo = 0.0038 We^0.99, r² = 0.7221) and dashed line indicate the Ba=We and Bo=We, respectively. The blue region indicates that the jumping locomotion of systems is dominated by surface tension rather than drag (We«1), and the systems locomotion in the red region is dominated by drag rather than surface tension. c The take-off velocity of the insects and robots depends on the Weber number. Color contour indicates the body density (kg/m³) on the Weber number simulated by a simple model consisting of three components: body (L³), foot (L²), and leg (L). Black lines indicate that the take-off velocity of the model with various body densities varies with the Weber number. Data for characteristic leg speed U, area A, width w, and the take-off velocity collected from (1) Yang et al. (2016), Hu et al. (2010) and Hu et al. (2003) (2) Suter et al. (2000) and Shin et al. (2008) (3) Sudo et al. (2015), Hu et al. (2010) and Kim et al. (2017), (4) Burrows et al. (2012), (5) Bush et al. (2006), Hsieh et al. (2003) and Glasheen et al. (1996) (6) Shin et al. (2008), (7) Yang et al. (2016), (8) Hu et al. (2010), (9) Koh et al. (2015).