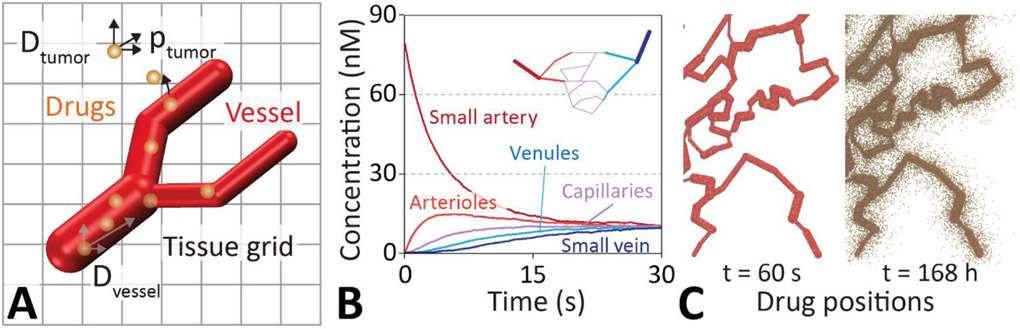
Figure 1 ∣. Drug diffusion model schematic and drug equilibration inside a vasculature.

A. Drug delivery is modeled by diffusive flow of particles through the vasculature and into the tissues using Brownian dynamics. Drugs incident on the walls of the vasculature are transferred back and forth between the vessel and the tissue with a given probability (${\mathrm{p}}_{{}_{{}^{\text{tumor}}}}$) via a kinetic Monte Carlo scheme. The vasculature is modeled by an interconnected network of cylinders. These are connected via spheres to allow branching as well as changing of the direction and radius of blood vessels. Interstitial space and tissues are represented as a micrometer resolution cubic grid. B. Rapid diffusive equilibration of an injected drug dosage throughout the small arteries (red), arterioles (light red), capillaries (pink), venules (light blue), and veins (blue) of a simple vascular network (inset). C. Three dimensional distribution of drugs in a vasculature and surrounding tissue at t = 60s and 168h. The model captures the position of individual drug molecules (brown spheres) as they transfer out of the vessel and disperse into the tissue.