FIGURE 1.

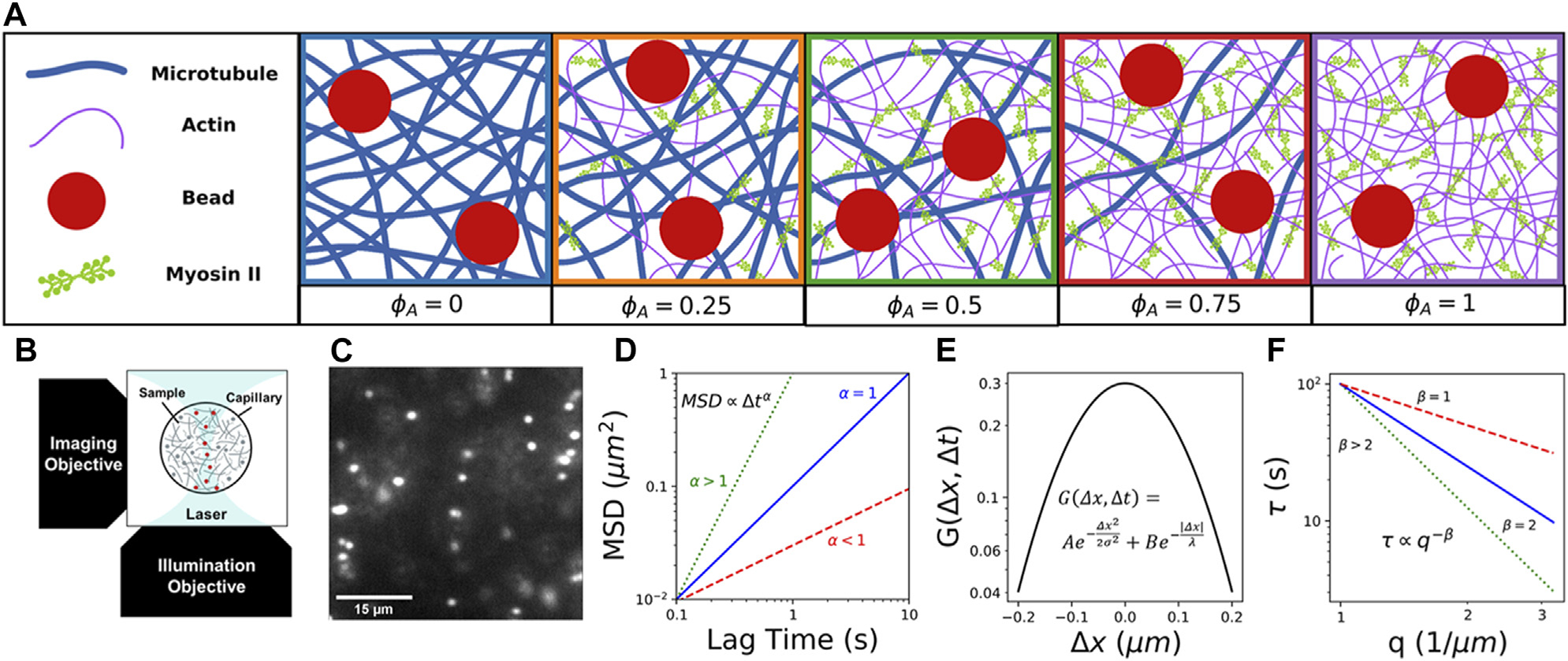
Combining light sheet microscopy with real-space single-particle tracking (SPT) and reciprocal-space differential dynamic microscopy (DDM) to characterize particle transport in active cytoskeletal composites. (A) We create composites of co-entangled microtubules (blue) and actin filaments (purple) driven out-of-equilibrium by myosin II minifilaments (green). We track the motion of embedded 1 μm beads (red) in composites with varying molar fractions of actomyosin, which we denote by the fraction of actin comprising the combined molar concentration of actin and tubulin $\left(5.8\mu \mathrm{M}\right):{\varphi }_A=0.0.25,0.5,0.75,1$. In all cases, the molar ratio of myosin to actin is fixed at 0.08. (B) Schematic of the light-sheet microscope we use for data collection, which provides the necessary optical sectioning to capture dynamics in dense three-dimensional samples. (C) Example frame from time-series of $1\mu \mathrm{m}$ beads embedded in a cytoskeleton composite, used to characterize particle transport in active crowded systems. (D) Cartoon of expected mean-squared displacements $(MSD)$ of embedded particles versus lag time $\mathrm{\Delta }t$, which we compute via single-particle tracking (SPT) and fit to a power law $MSD\tilde{\mathrm{\Delta }}{t}^{\alpha }$ to determine the extent to which particles exhibit nomal Brownian diffusion ($\alpha =1$, blue). subdiffusion ($\alpha <1$, red), or superdiffusion ($\alpha >1$, green). (E) Cartoon van Hove distribution $G$ of $x$- and $y$-direction particle displacements $\Delta d=\Delta x\cup \Delta y$ for a given lag time $\mathrm{\Delta }t$ computed from SPT trajectories. The distribution shown is described by a sum of a Gaussian and exponential function $G(\Delta d,\Delta t)=A{e}^{-\Delta d^2/2{\sigma }^2}+B{e}^{-\left|\Delta d\right|/\lambda }$, as is often seen in crowded and confined systems and those that display heterogeneous transport. (F) Cartoon of expected characteristic decorrelation times $\tau (q)$ as a function of wave number $q$, which we compute by fitting the image structure function computed from DDM analysis. We determine the scaling exponent $\beta$ from the power-law $\tau \left(q\right)~{\alpha }^{-\beta }$ to determine if transport is diffusive ($\beta =2$, blue). subdiffusive ($\beta >2$, green), or ballistic ($\beta =1$, red).