TABLE 3.
Main steps in population model buildinga
| Step | Model description | OBFVb | Comments |
|---|---|---|---|
| 1 | One-compartment model, CL = θ1CLCR | 1,476 | ςɛ2 = 16.8 |
| 2 | Two-compartment model, CL = θ1CLCR | 1,325 | ςɛ2 = 0.441; much better than step 1 |
| 3 | Infusion duration implemented as a random variable | 1,325 | Not better than step 2 |
| 4 |
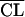 = θ1CLCR + θ2 = θ1CLCR + θ2
|
1,290 | Better than step 2 |
| 5 |
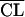 = θ1 × 6 × [θ3 − (age/100)]/(bw/SCR) + θ4, with i = 1 for males and 2 for females = θ1 × 6 × [θ3 − (age/100)]/(bw/SCR) + θ4, with i = 1 for males and 2 for females |
1,281 | Better than step 4c |
| 6 | Similar to step 5, but θ1 = θ2 | 1,285 | Sex is a significant covariatec |
| 7 to 10 | Typical values of either CL, V1, CLD, or Vt are allowed to differ according to o.d. or b.i.d. regimen | 1,282 to 1,287 | No influence of dosing regimen |
| 11 to 14 | Similar to steps 7 to 10 but with separated η’s according to o.d. or b.i.d. regimen | 1,282 to 1,289 | No influence of dosing regimen |
| 15 |
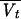 = θ7 + [(θ8 − θ7) × time]/(θ9 + time) = θ7 + [(θ8 − θ7) × time]/(θ9 + time) |
1,281 | θ8 tends to θ7; no influence of time on Vt |
| 16 | Similar to step 15, but for V1 | 1,281 | No influence of length of therapy |
| 17 |
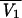 = θ5 × (bw/65) + θ6 = θ5 × (bw/65) + θ6
|
1,280 | Not significantc |
| 18 |
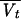 = θ7 (bw/65) + θ8 = θ7 (bw/65) + θ8
|
1,281 | Not significantc |
| 19 | Similar to step 5, but with FOCE η-ɛ interaction method | 1,248 | ςɛ2 = 0.189 |
| 20 | Similar to step 19, but C = Ĉ + ɛ1Ĉb + ɛ2 with var (ɛ2) fixed to 0.25 | 1,258 | Not better than step 19 |
After step 2 the two-compartment model was always used. After step 6 the clearance model described in step 5 was always used.
OBFV, objective function value.
Additional criteria (see Materials and Methods section) were also considered for the decision.
