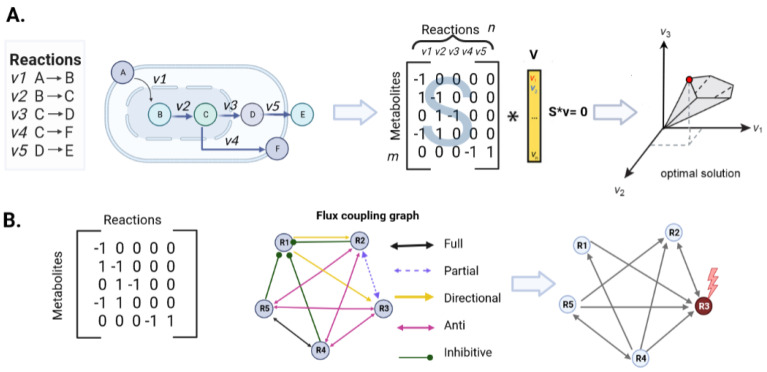
Figure 2.

Flow balance analysis (FBA) scheme and representation of the flow coupling graph. (A) The metabolic network consists of a list of stoichiometrically balanced biochemical reactions (R1, R2, R3, Rn). This type of reconstruction can be represented mathematically by a stoichiometric matrix (S) of size m × n composed of its reactions and metabolites. The steady-state flow distribution is defined by the equation S*v = 0, where v is a flow vector. According to the objective function of interest, maximization or minimization is performed; optimization allows for the finding of the flux distribution that enables the optimal solution for this objective function while observing the restrictions given the principle of mass balance and the limits of reaction [21]. (B) The application of control theory starts from the use of matrix S. The vertices represent reactions, and the labeled edges represent the five coupling relationships (represented by different colors; see legend). The steady-state principle implies that some reactions operate in a concentrated manner, leading to reaction coupling relationships. Reaction i is directionally coupled with j if σj v = 0 implies σi v = 0. Partial coupling is a particular case of directional and full coupling: two reactions, i and j, are partially coupled if they have the same state. If one of the two reactions is inactive, then a steady-state flux is only possible if the other reaction has a non-zero flux, which would define two anti-coupled reactions. Finally, reaction i couples inhibition with reaction j if the maximum flux of reaction i implies that j is inactive. The above can be summarized as follows: (1) the directional and total (or active) flow of R1 leads to the activation of R2 and R3 and the inactivation of R4 and R5; (2) the inactive flow of R1 leads to the deactivation of R2 and the activation of R4 and R5; (3) the inactive flow of R1 leads to the activation of R2 and the deactivation of R4. Taken and modified from [29].