Fig. 2.

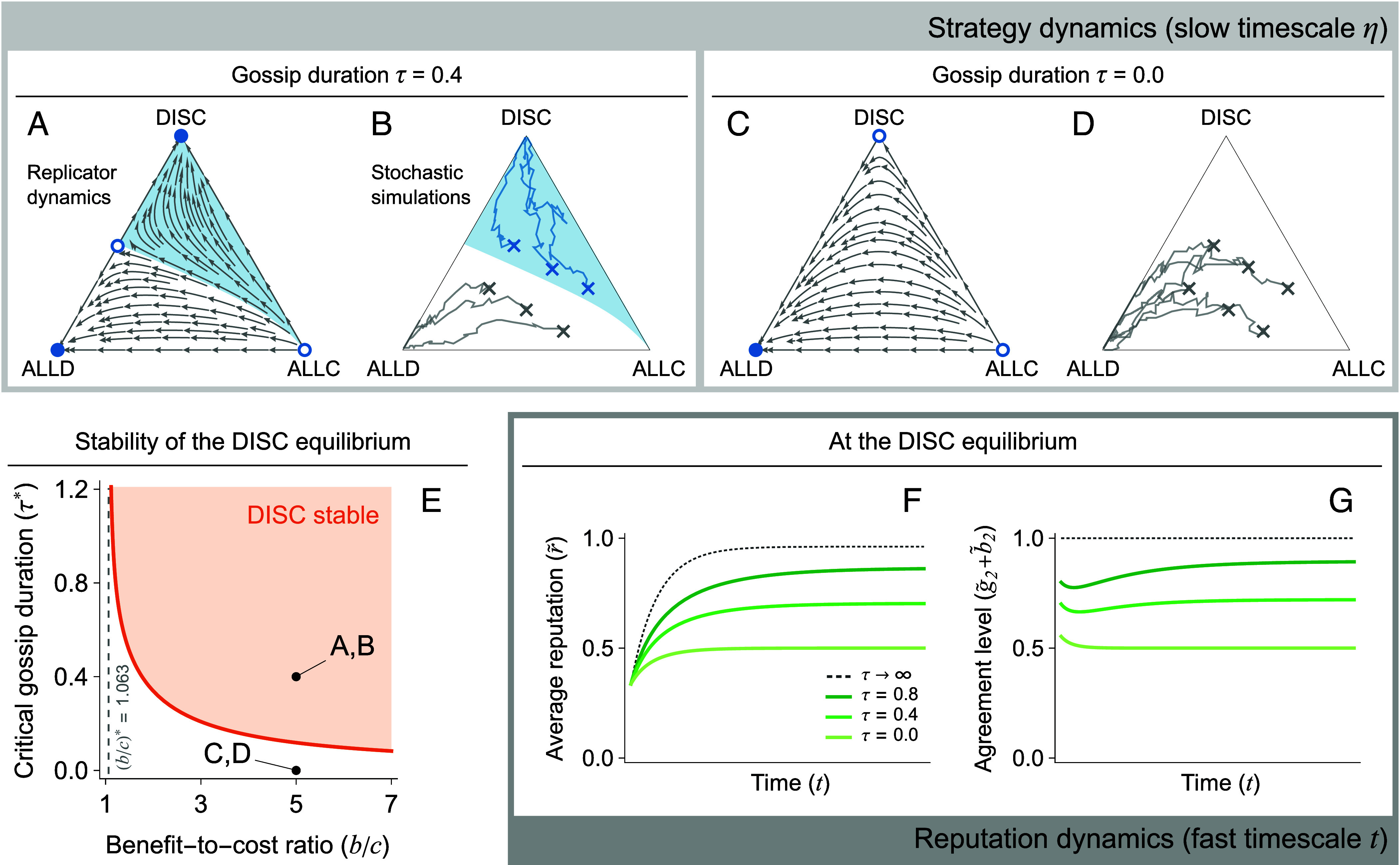
Sufficiently long gossip stabilizes cooperation. (A–D) Dynamics of competition among strategies ALLC, ALLD, and DISC under the Stern Judging norm, with a fixed benefit-to-cost ratio ($b/c=5$). (A and C) Gradients of selection (arrows) in the replicator dynamics (Eq. 6)). There is a basin of attraction toward the DISC vertex (shaded region) when $\tau =0.4>{\tau }^{\ast }$ (A) but not when $\tau =0.0<{\tau }^{\ast }$ (C). (B and D) Trajectories of stochastic simulations in a finite population ($N=100$), with x’s indicating different initial conditions (Materials and Methods). The long-term behavior of the stochastic simulations is consistent with the analytical predictions of the replicator-dynamic ODEs (B vs. A; D vs. C): When $\tau =0.4>{\tau }^{\ast }$ (B), trajectories starting from initial conditions above the separatrix tend to converge to the DISC vertex (the shaded region denotes the basin of attraction in A). When $\tau =0.0<{\tau }^{\ast }$ (D), all six trajectories converge to the ALLD vertex. (E) The discriminator-only equilibrium ($f_{\text{ALLD}}=1$) is locally stable only if the scaled gossip duration $\tau$ exceeds a critical value ${\tau }^{\ast }$ (solid orange curve defined by condition ii) and $b/c$ exceeds a critical value ${(b/c)}^{\ast }$ (dashed gray line defined by condition i). The orange region indicates parameter values where both these conditions are satisfied. The critical gossip duration ${\tau }^{\ast }$ decreases with the benefit-to-cost ratio, $b/c$. (F and G) The average reputation and agreement level as a function of time $t$ during the process of reputation dynamics by independent observations and gossip (Eq. 2). These quantities are evaluated at the DISC vertex ($f_{\text{DISC}}=1$) with a fixed benefit-to-cost ratio ($b/c=5$). Colors correspond to different values for the duration of gossip periods, $\tau$. The lightest green corresponds to $\tau =0.0$ (no gossip), which is equivalent to private reputations. The gray dashed curves correspond to $\tau \to \infty$ (infinitely long gossip), which is equivalent to public reputations. Other parameters: $u_a=u_e=0.02$. Analogous results for the Simple Standing and Shunning norms are shown in SI Appendix, Fig. S2.