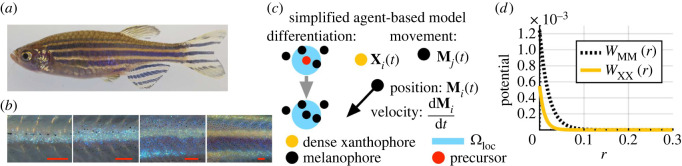
Figure 1.

Motivating biological example and model. (a) Wild-type zebrafish feature stripe patterns in their skin. These patterns consist of several types of brightly coloured pigment cells. (b) Over the course of a few months, these cells organize sequentially into stripes and interstripes from the centre of the fish body outward [10]. (c) For the purposes of this paper, we focus on a single population of black melanophores or gold dense xanthophores, using a simplified version of the model from [14]. The agent-based model (ABM) [14] that motivates our work describes how patterns arise through cell differentiation, competition and movement. In our simplified version of the ABM [14], we assume new cells appear at randomly selected locations based on short-range activation; this models cell differentiation from uniformly distributed precursors (red position) [14], and we also refer to this as ‘proliferation’ or ‘birth’ in this paper. (We describe the cell differentiation rules in the full model [14] in more detail in electronic supplementary material, figure S1.) In both our work and the ABM from [14], cell movement is deterministic and governed by ordinary differential equations (ODEs). (d) These ODEs account for cell–cell repulsion through potential functions, which describe melanophore–melanophore ($W_{\mathrm{MM}}$) and xanthophore–xanthophore ($W_{\mathrm{XX}}$) interactions as a function of their pairwise distance r. Red scale bar is 250 μm in (b). Image (a) adapted from Fadeev et al. [15] and licensed under CC-BY 4.0 (https://creativecommons.org/licenses/by/4.0/). Image (b) adapted from Frohnhöfer et al. [10] and licensed under CC-BY 3.0 (https://creativecommons.org/licenses/by/3.0/); published by The Company of Biologists Ltd.