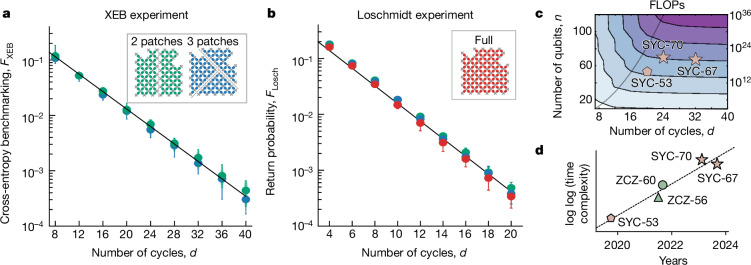
Fig. 4. Demonstration of a classically intractable computation.

a, Verification of RCS fidelity with logarithmic XEB. The full device is divided into two (green) or three (blue) patches to estimate the XEB fidelity for a modest computational cost. We used the discrete gate set of single-qubit gates chosen randomly from Z^pX^1/2Z^−p with p ∈ {−1, −3/4, −1/2, …, 3/4}. For each number of cycles, 20 circuit instances were sampled with 100,000 shots each. The solid lines indicate the XEB estimated from the digital error model. b, Verification of RCS fidelity with Loschmidt echo. The inversion was done by reversing the circuit and inserting single-qubit gates. In this case, the Loschmidt echo number of cycles doubled. c, Time complexity estimated as a function of the number of qubits and the number of cycles for a set of circuits. As a working definition of time complexity, we used the number of FLOPs needed to compute the probability of a single bit string under no memory constraints. The solid line indicates the depth at which correlations spread to the full device and the FLOPs with depth go from exponential to polynomial. d, Evolution of the time complexity of the RCS experiments. The dashed line represents doubly exponential growth as a guide for the eye.