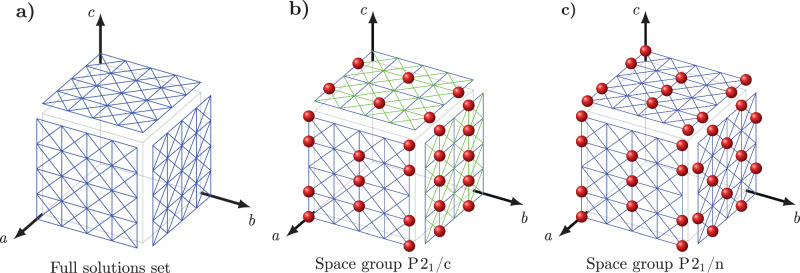
Fig. 2. Illustrations of the solutions of equation (10) for the Zernike order parameters.

a The full set of solutions of equation for select space groups. The figure shows the intersections between the set of planes forming the solutions to Eq. (10) for the ZOPs and the faces of the unit cell. In general, the solutions are either planes parallel to the faces of the unit cell or parallel to the diagonals of the unit cell. b, c Projections of the high density regions (red spheres) on the faces of the unit cell in fractional coordinates as generated from the analysis of the molecular positions of the organic molecular crystal structures in the P2₁/c and P2₁/n space groups with one molecule in the asymmetric unit ($Z^{\prime }=1$). The green lines correspond to solutions missing from the specific space group. For all space groups, the high-density regions are found on a zero Zernike position (ZZP) or on the intersection between two or three ZZPs. There are clear differences in the positions of the high-density regions among the different space groups, indicating that the geometry of the unit cell and, more specifically, the symmetry in each space group is correlated with the arrangements of the molecules.