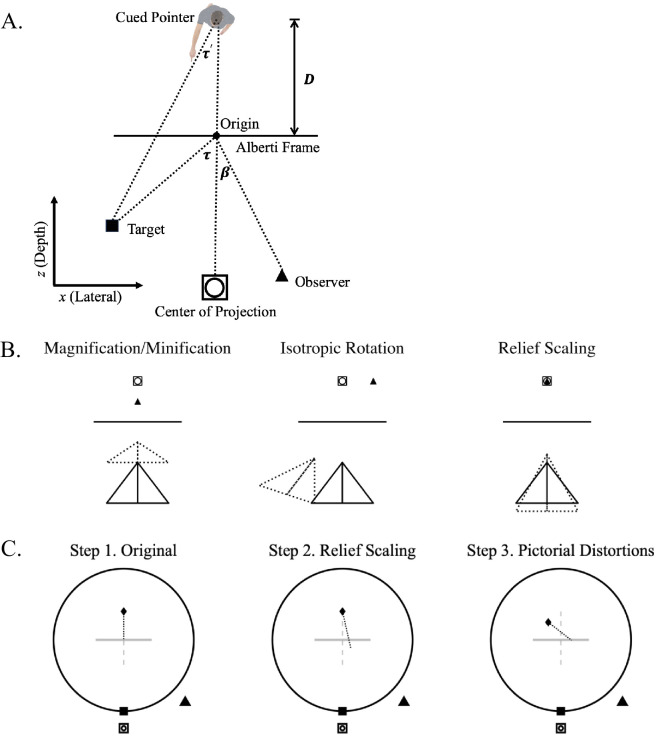
Figure 4.

(A) An illustration of the model environment as a 2D xz-plane, where the x-axis is parallel and the z-axis is perpendicular to the plane of the Alberti Frame, and the origin is centered at the Alberti Frame. See text for details. (B) Illustrations of the effects of magnification/minification (perpendicular displacement; Equation 3 with ω = 1), isotropic rotation (lateral displacement; Equation 4 with ω = 1), and relief scaling on the perceived object (dashed triangles; Equation 5 with C = 1.4). The solid triangles represent the observer's vantage point, whereas open squares and circles represent the image's center of projection. (C) A step-by-step illustration of the linear transformations. Step 1: In the original visual environment, the pointer (filled diamond) is cued behind the Alberti Frame (gray horizontal line) and pointing at the 0° target (filled square). Step 2: Because of relief scaling, the pointer's perceived dimensions are expanded along the observer's (filled triangle) line of sight, which slightly perturbs the pointer's perceived pointing direction. Step 3: The discrepancy between the observer's vantage point (filled triangle) and the image's center of projection (open square and circle) leads to pictorial distortions, which rotate the entire perceptual space of the pointer around the center of the frame by an amount proportional to the observer's viewing angle. Note: (1) because the pointer is not perceived to be at the center of the frame, its perceived location is also rotated around the frame, and (2) the transformed pointing direction does not exactly coincide with the observer's location due to the relief scaling in Step 2.