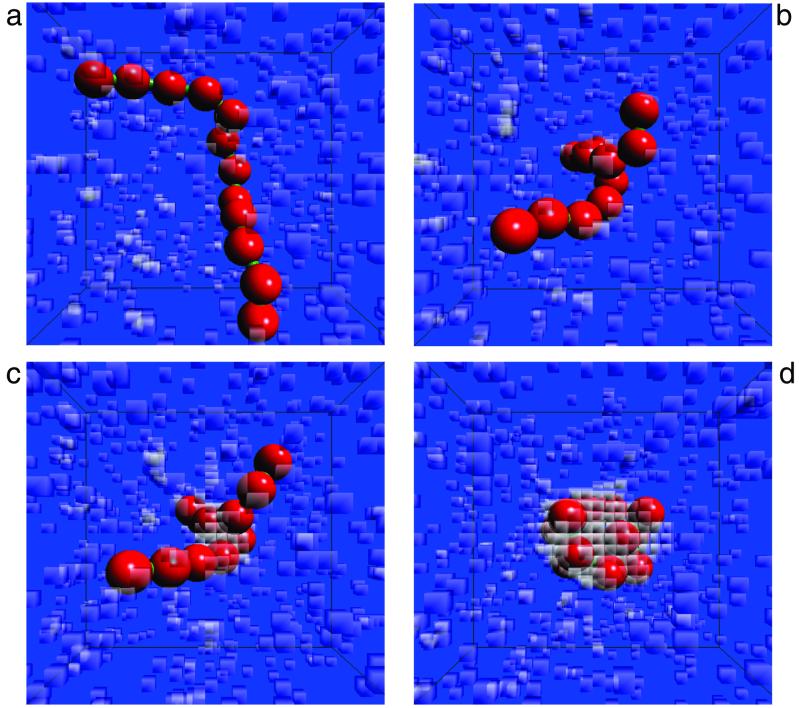
Figure 2.

Four configurations from a trajectory where a 12-unit hydrophobic chain in water goes from the coil to the globule state. (a) A configuration from the equilibrated coil. The chain remained in configurations like that throughout a 10-ns run at room temperature (T = 0.663ɛ). On a much longer time scale, about 10⁻⁵ s, the chain typically does exhibit a transition from the coil to globule. Such events are found with transition path sampling, equilibrating from an initial high-temperature (T = 0.74ɛ), 10-ns trajectory that exhibited the transition spontaneously. Three configurations exhibiting that transition, covering a stretch of 1.5 ns, are shown in b, c, and d, with that in c being a configuration from the transition state surface. The transparent cubes denote the vapor cells. Those seen far from the chain are typical spontaneous density fluctuations in bulk liquid water. The size of the simulation box is 397 nm³, corresponding to 42,875 cells. The parameters characterizing the energy of the solvent are given in the caption to Fig. 1. The intrachain potential, V(r₁, r₂, . . . , r₁₂), is a function of the positions of the centers of each of the 12 hydrophobic spheres (the red particles in the figure). It contains three parts: (i) steep (essentially hard sphere) repulsions between solute particles such that their interparticle separations are larger than σ = 0.72 nm; (ii) stiff harmonic potentials bonding adjacent particles in the hydrophobic chain, ½k_s(σ − |r_α+1 − r_α|)², with k_s = 14.1 J/m²; (iii) a bending potential favoring an extended chain, ½k_θθ_α², where θ_α is the angle between (r_α+2 − r_α+1) and (r_α+1 − r_α), and k_θ = 1.85 × 10⁻²⁰ J/rad². The volumes v_i excluded from water by the chain are dynamic because they change with changing chain configuration—i.e., v_i = v_i({r_α}). Specifically, these volumes are computed by assuming water molecules have van der Waals radii equal to 0.14 nm, and that the diameter of each hydrophobic unit is σ = 0.72 nm. That is, points in the excluded volume, r, are those in the union of all volumes inscribed by |r − r_α| < 0.5 nm, α = 1, 2, . . . , N_s.