Skip to main content
PMC home page
Journal of the Experimental Analysis of Behavior logoLink to Journal of the Experimental Analysis of Behavior
. 1989 Jan;51(1):145–162. doi: 10.1901/jeab.1989.51-145

Response-reinforcer contingency and spatially defined operants: testing an invariance property of phi.

G Galbicka 1, J R Platt 1
PMCID: PMC1338897  PMID: 2921584

Abstract

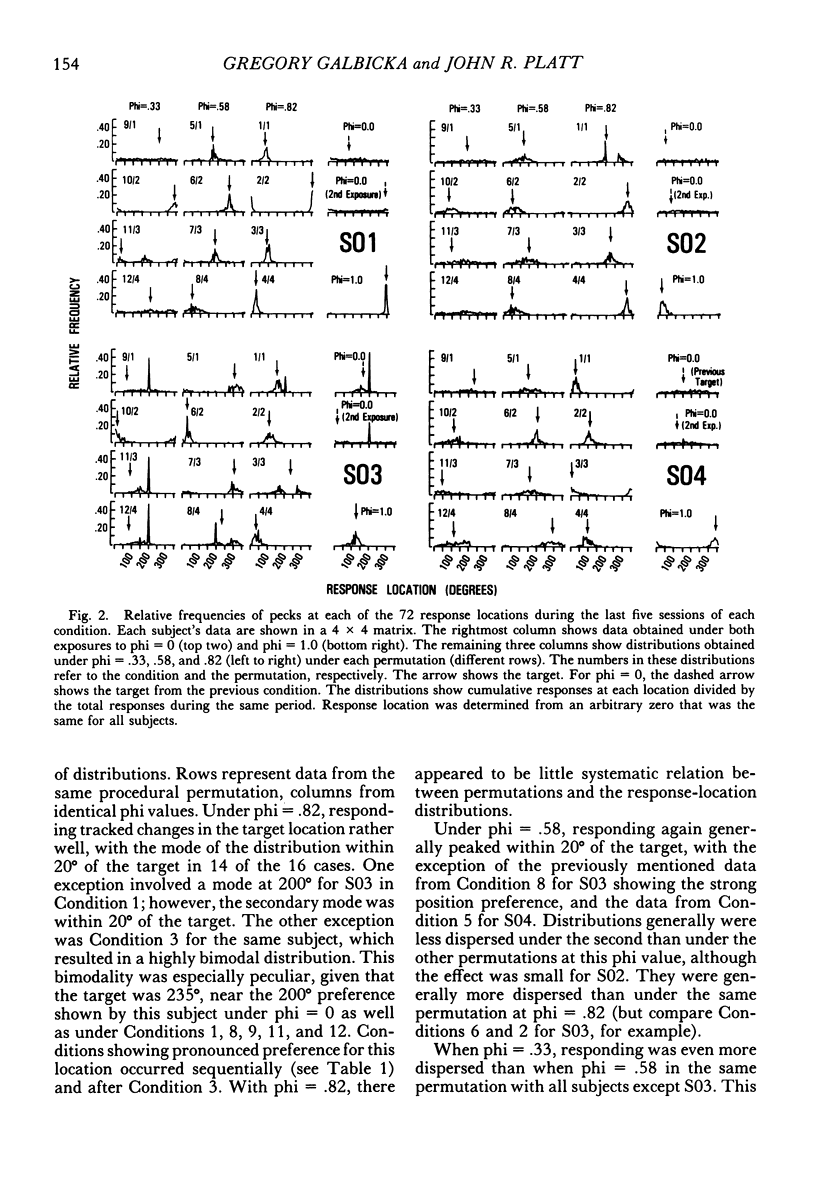
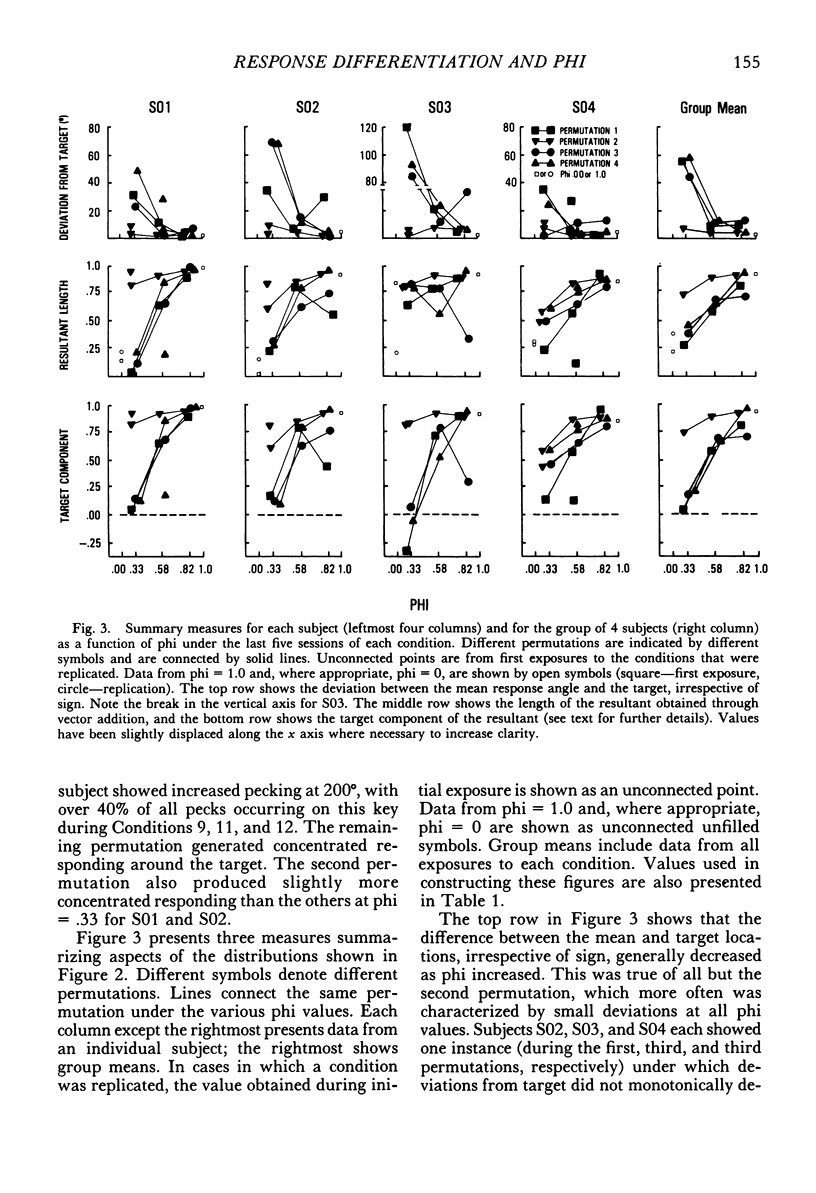
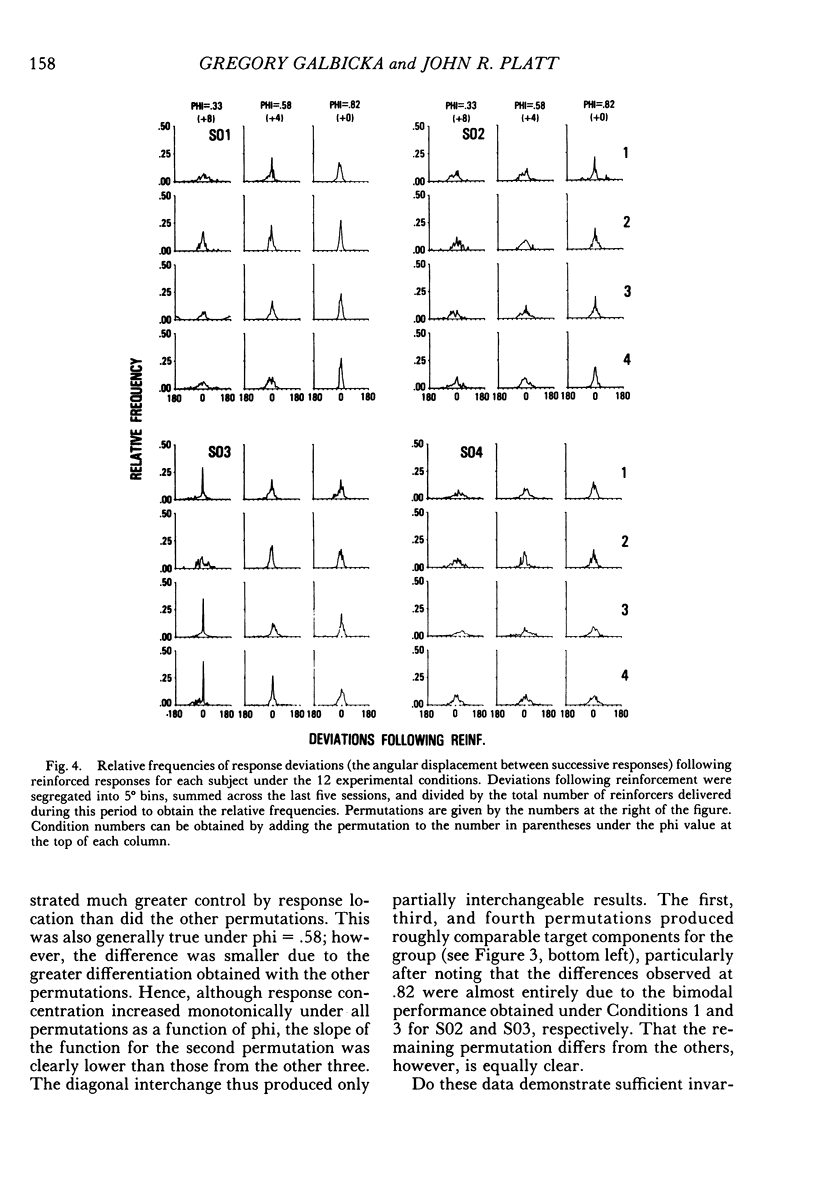
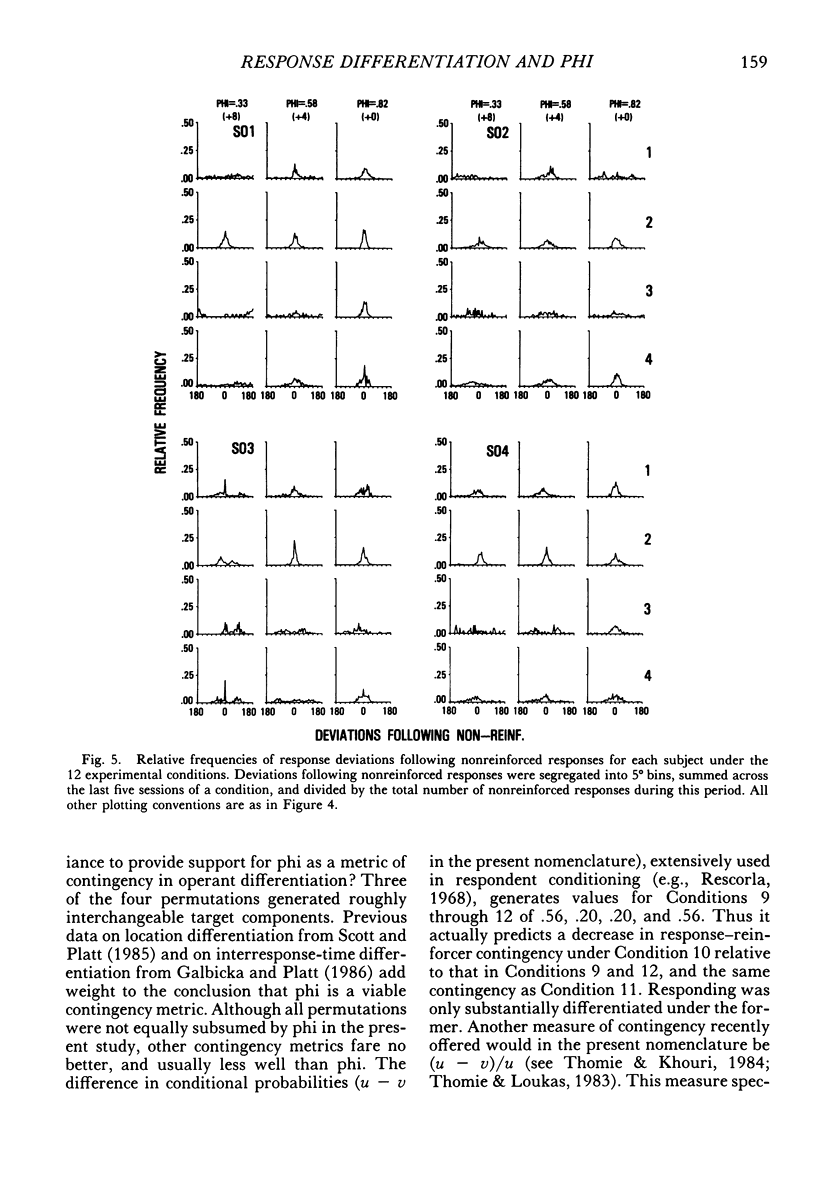
A chamber containing 72 response keys defining the circumference of a circle 1 m in diameter was used to examine the relation between differentiation of response location and a measure of response-reinforcer contingency known as the phi coefficient. A different target key was specified in each successive phase, and response location was differentiated with respect to the target. Criterional and noncriterional responses (i.e., responses "near" and "far" from the target) were defined using targeted percentile schedules to control the overall probability of each response class. By manipulating criterional (and, hence, noncriterional) response probability and the reinforcement probabilities conditional on each, a mathematical invariance property peculiar to phi in contingency analysis was examined. Specifically, diagonally interchanging cell frequencies in a 2 x 2 table relating criterional/noncriterional responses to reinforcement/nonreinforcement leaves phi unchanged. Hence, the degree of response differentiation predicted by phi remains unchanged under the four permutations implied by the various diagonal interchanges. This predicted invariance was examined under values of phi equal to .33, .58, and .82. Increasing phi generally increased the stereotypy of response location. Three of the permutations generated almost interchangeable performance at different phi values. The remaining permutation, however, generated functions relating response concentration to phi with slopes shallower than those obtained under the other permutations. This resulted from relatively higher levels of differentiation, compared to the other permutations, at low phi values. These data strongly suggest boundary conditions on the ability of phi to reflect completely the local processes that are indexed by phi at a molar level.

Full text

PDF
148

Selected References

These references are in PubMed. This may not be the complete list of references from this article.

  1. Baum W. M. On two types of deviation from the matching law: bias and undermatching. J Exp Anal Behav. 1974 Jul;22(1):231–242. doi: 10.1901/jeab.1974.22-231. [DOI] [PMC free article] [PubMed] [Google Scholar]
  2. Davison M. C., Tustin R. D. The relation between the generalized matching law and signal-detection theory. J Exp Anal Behav. 1978 Mar;29(2):331–336. doi: 10.1901/jeab.1978.29-331. [DOI] [PMC free article] [PubMed] [Google Scholar]
  3. Eckerman D. A., Lanson R. N. Variability of response location for pigeons responding under continuous reinforcement, intermittent reinforcement, and extinction. J Exp Anal Behav. 1969 Jan;12(1):73–80. doi: 10.1901/jeab.1969.12-73. [DOI] [PMC free article] [PubMed] [Google Scholar]
  4. Galbicka G. Differentiating the behavior of organisms. J Exp Anal Behav. 1988 Sep;50(2):343–354. doi: 10.1901/jeab.1988.50-343. [DOI] [PMC free article] [PubMed] [Google Scholar]
  5. Galbicka G., Platt J. R. Parametric manipulation of interresponse-time contingency independent of reinforcement rate. J Exp Psychol Anim Behav Process. 1986 Oct;12(4):371–380. [PubMed] [Google Scholar]
  6. Gibbon J., Berryman R., Thompson R. L. Contingency spaces and measures in classical and instrumental conditioning. J Exp Anal Behav. 1974 May;21(3):585–605. doi: 10.1901/jeab.1974.21-585. [DOI] [PMC free article] [PubMed] [Google Scholar]
  7. Hammond L. J. The effect of contingency upon the appetitive conditioning of free-operant behavior. J Exp Anal Behav. 1980 Nov;34(3):297–304. doi: 10.1901/jeab.1980.34-297. [DOI] [PMC free article] [PubMed] [Google Scholar]
  8. Herrnstein R. J. On the law of effect. J Exp Anal Behav. 1970 Mar;13(2):243–266. doi: 10.1901/jeab.1970.13-243. [DOI] [PMC free article] [PubMed] [Google Scholar]
  9. Kuch D. O., Platt J. R. Reinforcement rate and interresponse time differentiation. J Exp Anal Behav. 1976 Nov;26(3):471–486. doi: 10.1901/jeab.1976.26-471. [DOI] [PMC free article] [PubMed] [Google Scholar]
  10. McCarthy D., Davison M. Independence of stimulus discriminability from absolute rate of reinforcement in a signal-detection procedure. J Exp Anal Behav. 1982 May;37(3):371–382. doi: 10.1901/jeab.1982.37-371. [DOI] [PMC free article] [PubMed] [Google Scholar]
  11. Nevin J. A., Jenkins P., Whittaker S., Yarensky P. Reinforcement contingencies and signal detection. J Exp Anal Behav. 1982 Jan;37(1):65–79. doi: 10.1901/jeab.1982.37-65. [DOI] [PMC free article] [PubMed] [Google Scholar]
  12. Rescorla R. A. Probability of shock in the presence and absence of CS in fear conditioning. J Comp Physiol Psychol. 1968 Aug;66(1):1–5. doi: 10.1037/h0025984. [DOI] [PubMed] [Google Scholar]
  13. Shimp C. P. Sequential dependencies in free-responding. J Exp Anal Behav. 1973 May;19(3):491–497. doi: 10.1901/jeab.1973.19-491. [DOI] [PMC free article] [PubMed] [Google Scholar]
  14. Weiss B., Laties V. G., Siegel L., Goldstein D. A computer analysis of serial interactions in spaced responding. J Exp Anal Behav. 1966 Nov;9(6):619–626. doi: 10.1901/jeab.1966.9-619. [DOI] [PMC free article] [PubMed] [Google Scholar]

Articles from Journal of the Experimental Analysis of Behavior are provided here courtesy of Society for the Experimental Analysis of Behavior

RESOURCES