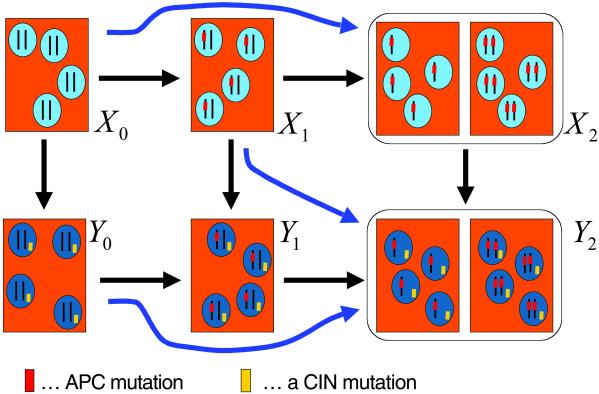
Fig 2.
The early steps of colon cancer occur in small crypts that contain a few thousand cells. The whole crypt is replenished from a small number of stem cells. The effective population size of the crypt, N, with respect to the somatic evolution of cancer might be of the order of 10 cells. As long as N2 ≪ 1/u (where u ≈ 10−7–10−6), then there is a high probability that at any one time crypts contain cells of only one type. Hence, we can investigate a stochastic process describing transitions among six different states, X0, X1, X2, Y0, Y1, and Y2, referring to homogeneous crypts of cell type x0, x1, x2, y0, y1, and y2, respectively. The transitions reflect the mutational network of Fig. 1. In addition, there are three stochastic tunnels. For certain parameter values, the system can tunnel from X0 to X2 without reaching X1. Similarly, there are tunnels from Y0 to Y2 and from X1 to Y2. Tunnels occur if the second step in a consecutive transition is much faster than the first one and if the final cell has a strong selective advantage.