Skip to main content
PMC home page
Biophysical Journal logoLink to Biophysical Journal
. 1972 Sep;12(9):1205–1217. doi: 10.1016/S0006-3495(72)86156-3

Viscoelasticity of Human Blood

G B Thurston
PMCID: PMC1484135  PMID: 5056964

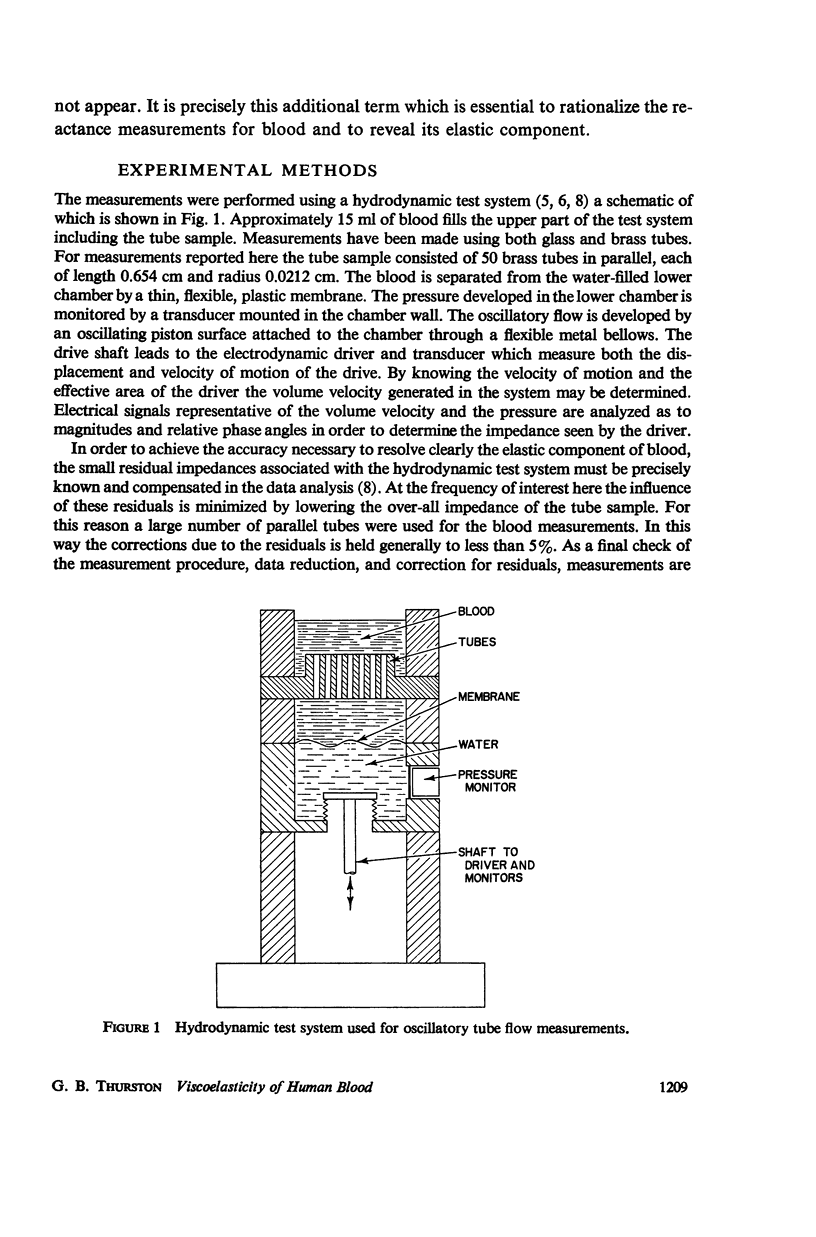
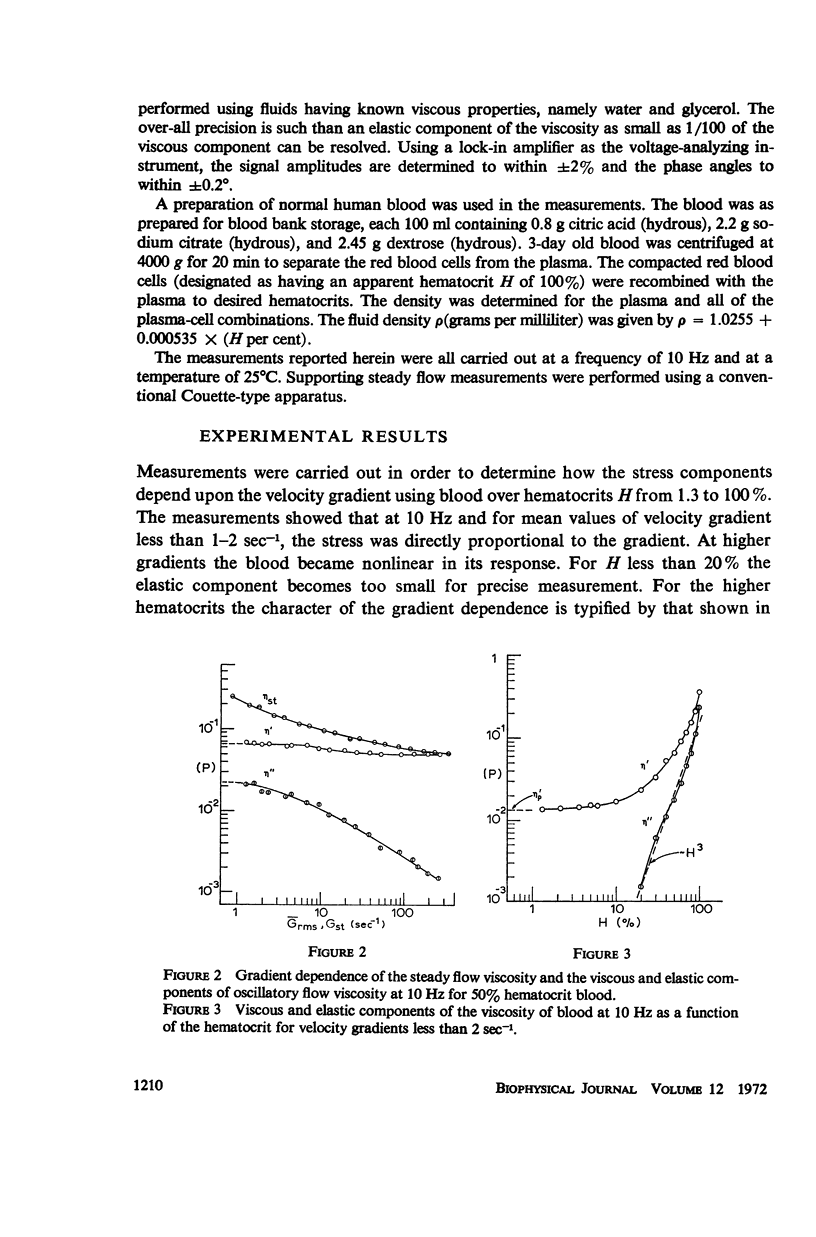
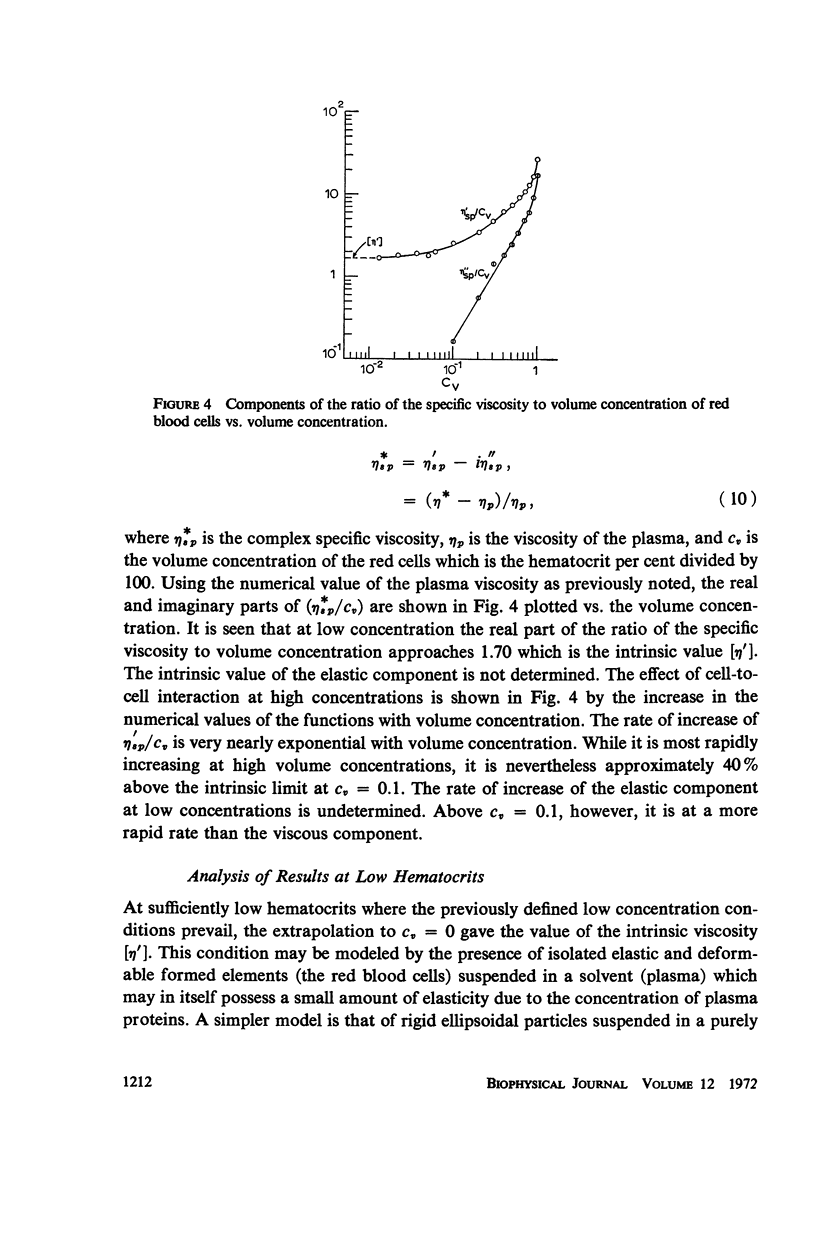
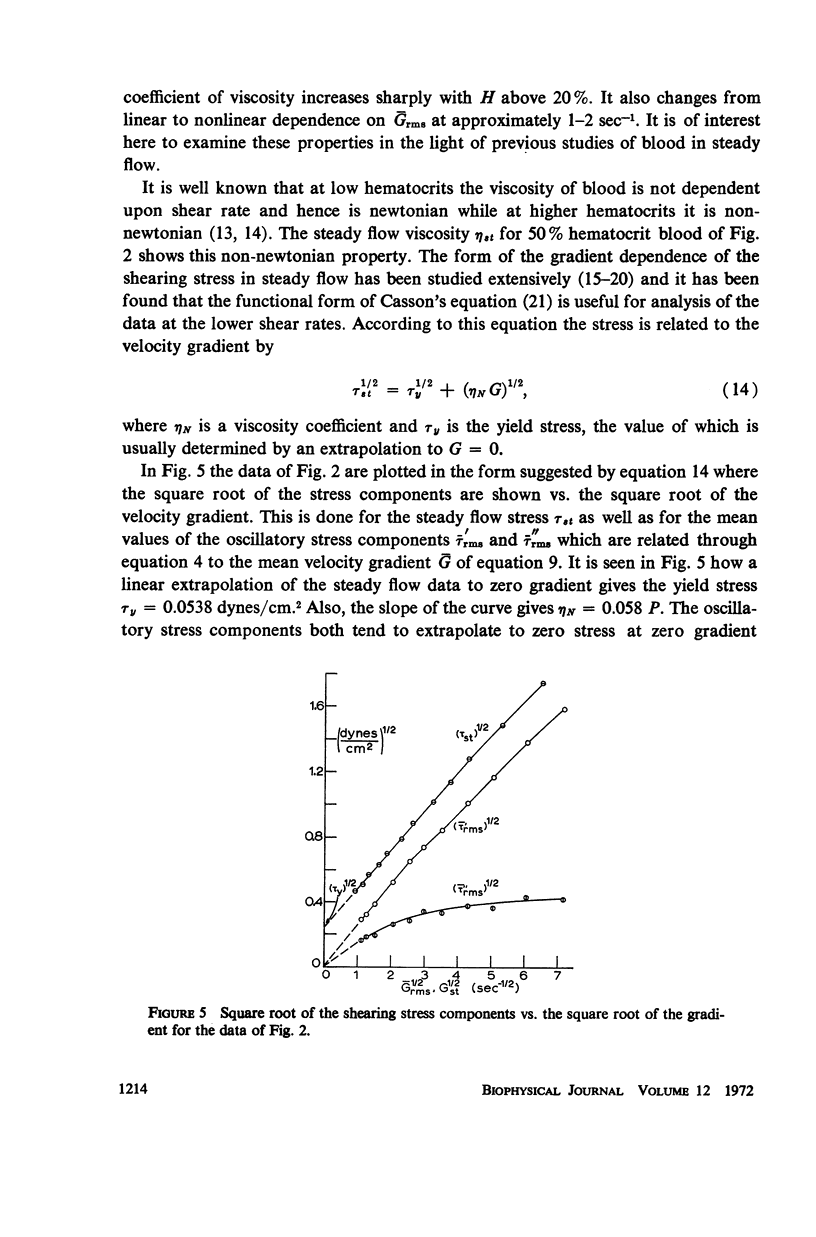
Abstract

Measurements made for oscillatory flow of blood in circular tubes show that blood possesses elastic properties which make consideration of its viscous properties alone inadequate. Results are for a frequency of 10 Hz while varying the amplitude of the velocity gradient for red blood cells in plasma at concentrations ranging from 0 to 100% apparent hematocrit. For velocity gradients less than 1-2 sec-1 both the viscous and elastic components of the shearing stress are linearly related to the gradient. For hematocrits above 20% the elastic component of the complex coefficient of viscosity increases with hematocrit approximately to the third power while the viscous component increases exponentially. Oscillatory flow measurements at very low hematocrits, when extrapolated to zero cell concentration, give the intrinsic viscosity of the average individual isolated red cell. The viscous part of this is found to be 1.7 which is compared with theoretical values from the rigid ellipsoid model for which the minimum possible value is 2.5. This difference is attributed to cell deformability. With increasing velocity gradient nonlinear properties develop. The viscous component of the complex viscosity becomes of the order of the steady flow viscosity at high gradients while the elastic component tends to decrease in inverse proportion to the gradient. Thus, the elastic component of the oscillatory stress tends to saturate, this tendency appearing at the approximate level of the yield stress.

Full text

PDF

Selected References

These references are in PubMed. This may not be the complete list of references from this article.

  1. BLAIR G. W. An equation for the flow of blood, plasma and serum through glass capillaries. Nature. 1959 Feb 28;183(4661):613–614. doi: 10.1038/183613a0. [DOI] [PubMed] [Google Scholar]
  2. Brooks D. E., Goodwin J. W., Seaman G. V. Interactions among erythrocytes under shear. J Appl Physiol. 1970 Feb;28(2):172–177. doi: 10.1152/jappl.1970.28.2.172. [DOI] [PubMed] [Google Scholar]
  3. Charm S. E., Kurland G. S. Static method for determining blood yield stress. Nature. 1967 Dec 16;216(5120):1121–1123. doi: 10.1038/2161121a0. [DOI] [PubMed] [Google Scholar]
  4. Chien S., Usami S., Dellenback R. J., Gregersen M. I. Blood viscosity: influence of erythrocyte deformation. Science. 1967 Aug 18;157(3790):827–829. doi: 10.1126/science.157.3790.827. [DOI] [PubMed] [Google Scholar]
  5. Chien S., Usami S., Dellenback R. J., Gregersen M. I., Nanninga L. B., Guest M. M. Blood viscosity: influence of erythrocyte aggregation. Science. 1967 Aug 18;157(3790):829–831. doi: 10.1126/science.157.3790.829. [DOI] [PubMed] [Google Scholar]
  6. Errill E. W. Rheology of blood. Physiol Rev. 1969 Oct;49(4):863–888. doi: 10.1152/physrev.1969.49.4.863. [DOI] [PubMed] [Google Scholar]
  7. Goldstone J., Schmid-Schönbein H., Wells R. The rheology of red blood cell aggregates. Microvasc Res. 1970 Jul;2(3):273–286. doi: 10.1016/0026-2862(70)90018-x. [DOI] [PubMed] [Google Scholar]
  8. Kunz A. L., Coulter N. A., Jr Non-Newtonian behavior of blood in oscillatory flow. Biophys J. 2008 Dec 31;7(1):25–36. doi: 10.1016/S0006-3495(67)86573-1. [DOI] [PMC free article] [PubMed] [Google Scholar]
  9. MERRILL E. W., GILLILAND E. R., COKELET G., SHIN H., BRITTEN A., WELLS R. E., Jr Rheology of human blood, near and at zero flow. Effects of temperature and hematocrit level. Biophys J. 1963 May;3:199–213. doi: 10.1016/s0006-3495(63)86816-2. [DOI] [PMC free article] [PubMed] [Google Scholar]
  10. Meiselman H. J., Merrill E. W., Salzman E. W., Gilliland E. R., Pelletier G. A. Effect of dextran on rheology of human blood: low shear viscometery. J Appl Physiol. 1967 Mar;22(3):480–486. doi: 10.1152/jappl.1967.22.3.480. [DOI] [PubMed] [Google Scholar]
  11. Merrill E. W., Benis A. M., Gilliland E. R., Sherwood T. K., Salzman E. W. Pressure-flow relations of human blood in hollow fibers at low flow rates. J Appl Physiol. 1965 Sep;20(5):954–967. doi: 10.1152/jappl.1965.20.5.954. [DOI] [PubMed] [Google Scholar]
  12. Merrill E. W., Cheng C. S., Pelletier G. A. Yield stress of normal human blood as a function of endogenous fibrinogen. J Appl Physiol. 1969 Jan;26(1):1–3. doi: 10.1152/jappl.1969.26.1.1. [DOI] [PubMed] [Google Scholar]
  13. Schmid-Schoenbein H., Wells R., Schildkraut R. Microscopy and viscometry of blood flowing under uniform shear rate (rheoscopy). J Appl Physiol. 1969 May;26(5):674–678. doi: 10.1152/jappl.1969.26.5.674. [DOI] [PubMed] [Google Scholar]
  14. Schmid-Schöenbein H., Wells R. Fluid drop-like transition of erythrocytes under shear. Science. 1969 Jul 18;165(3890):288–291. doi: 10.1126/science.165.3890.288. [DOI] [PubMed] [Google Scholar]
  15. Schmid-Schönbein H., Gaehtgens P., Hirsch H. On the shear rate dependence of red cell aggregation in vitro. J Clin Invest. 1968 Jun;47(6):1447–1454. doi: 10.1172/JCI105836. [DOI] [PMC free article] [PubMed] [Google Scholar]
  16. TAYLOR M. G. The influence of the anomalous viscosity of blood upon its oscillatory flow. Phys Med Biol. 1959 Jan;3(3):273–290. doi: 10.1088/0031-9155/3/3/307. [DOI] [PubMed] [Google Scholar]
  17. Walder D. N., Weaver J. P., Evans A. An apparatus to detect a yield stress in blood. Biorheology. 1969 Apr;6(1):23–32. doi: 10.3233/bir-1969-6103. [DOI] [PubMed] [Google Scholar]

Articles from Biophysical Journal are provided here courtesy of The Biophysical Society

RESOURCES