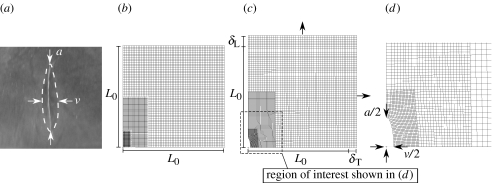
Figure 2.
A longitudinal incision in the dura mater of a 2-day-old rat and the finite-element model used to calculate the strains present in the dura mater. (a) Following incision, the length (a) and maximum width (v) of each incision was measured. The dashed line denotes the edge of the incision after being allowed to relax for 5 min. (b) The finite-element model used to calculate retraction of the edges of an incision made in rat dura mater under varying levels of tensile strain. The unstrained model had an initial side length of L0. (c) Longitudinal (δL) and transverse (δT) displacements were applied to the edges of the model to simulate straining of the dura mater in both the longitudinal and transverse directions. (d) For each combination of δL and δT applied to the model, we recorded the half-length (a/2) and the maximum half-width (v/2) of the incision.