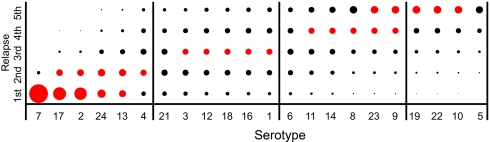
Fig. 5.

Model of relapses of B. hermsii infection when there is a hierarchy of switch rates. The bottom row of circles shows the frequencies, p_i, at which each serotype is detectable in the first-relapse bacteremia from the antisera-typing data (frequencies by serotype among the total of mice in Table 1), where the subscript i is the serotype number. Moving upwards, each row of circles shows the predicted frequency of detection in subsequent-relapse bacteremias. The total area of the circles across a row sums to a constant, so the circle areas represent the relative frequency at which a serotype is detectable in a particular bacteremia. In each row, the five red circles show the serotypes with the highest frequency of detection. We assumed that the current variant did not influence the switch rate (see Results) and that the abundance level that stimulated immunity, y, was 10-fold lower than the level at which it was detectable by antisera typing, x (see Supporting Methods, which is published as supporting information on the PNAS web site). In the absence of immunity, let the probability that the abundance of a type stimulates protective immunity be q_i = p_i^y/x. Then f_i = p_i(1 − q_i)ⁿ⁻¹, where the value (1 − q_i)ⁿ⁻¹ is the probability that, in the nth relapse, a serotype has not previously generated an immune response by appearing during one of the first n − 1 relapses. We used y/x = 0.1 to generate this figure; lower values of y/x, such as 0.01, cause only small qualitative changes in the patterns of dominance in successive relapses.