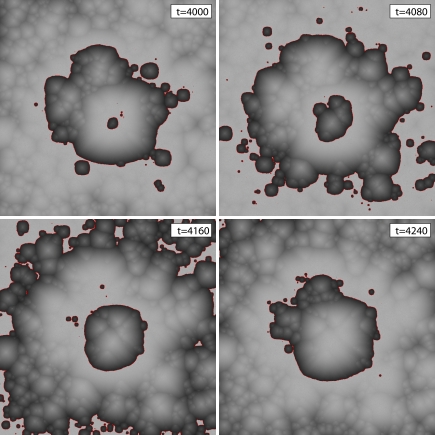
Fig. 1.

An autonomous self-organized periodic wave source generated by noise. Results of the numerical solution of Eq. 1 with α = 0.01, ε = 0.05, A = 0.7, B = 6.4 × 10⁻⁵, and h = 3.16 are shown. The system is discretized by finite difference on a 800 × 800 grid with reflecting boundary conditions. Red pixels denote the regions where u > 5, and the gray scale shows v (white is v = 1, black is v = 0, with various shades of gray showing v in between). The system is initially seeded with u = 0.5, v = 0.05 + 0.65 exp(−((x − 400 h)² + (y − 400 h)²)/(200 h)²), a bell-shaped distribution of the recovery variable. Note that the initial values of v are all below the deterministic threshold for firing. After a number of cycles a steadily oscillating pattern in which waves are initiated periodically in the center of the system is established. Once initiated, these waves propagate radially outward, generating a characteristic target pattern. The pattern persisted for as long as the simulation could be run. The snapshots are taken after ≈100 periods [see supporting information (SI) Movies 1–10]. Notice the characteristic formation of the nuclei ahead of the wave, which are then absorbed by the oncoming wave front; this is strongly reminiscent of the observations of cAMP signaling in Dictyostelium populations (19). We emphasize that this pattern is impossible in the same model in the absence of the noise.