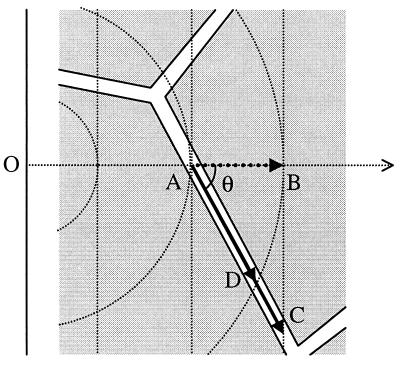
Figure 4.

Difference between tortuosity for unidirectional and radial flux. A particle at A, flowing down its concentration gradient (horizontal dotted arrow), is confined to an intercellular pore. In the case of unidirectional flux, it reaches the next iso-density contour (vertical dotted line) at C. In the absence of obstacles, it would have reached the next contour at B. The ratio of path length AC/AB, equivalent to 1/cos θ, averaged over space, is thus equivalent to λ_x, the tortuosity for unidirectional flux. If, however, the diffusion flux is radial, originating from a point source at O, the particle only needs to travel as far as D to reach the next isodensity contour (circular dotted lines). The tortuosity for radial flux λ_r, is thus given by averaging the ratio AD/AB over space. Because AC > AD, it follows that λ_x > λ_r.