Figure 4.
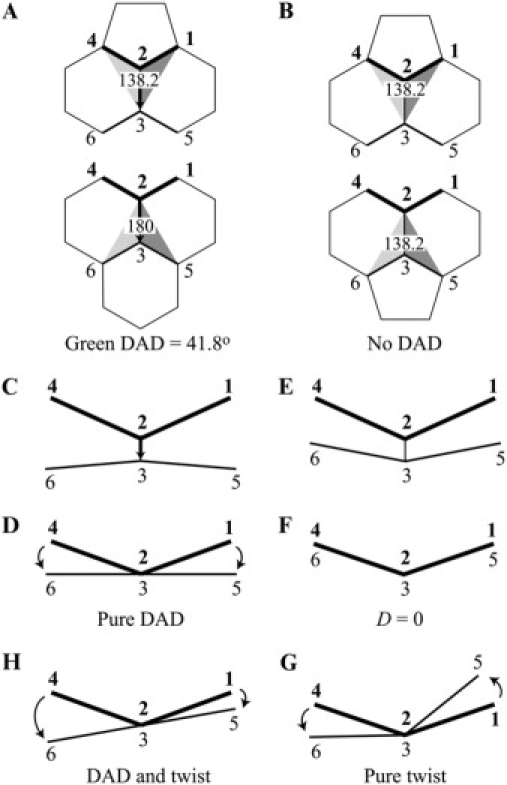
A physical description of DAD and twist. (A) The green DAD edge has two hexagonal side faces, a pentagon at the top, and a hexagon at the bottom. Focusing on the two end vertices one at a time, the dihedral angle about edge 23 at its top is 138.2° and at its bottom is 180°. The DAD is thus 41.8°, pointing from top to bottom. Bolded vertex numbers are in front; unbolded vertex numbers are in back. (B) This edge has the same end faces, both pentagons, so it is without DAD. Focusing on the two end vertices one at a time, the dihedral angle about edge 23 at its top is 138.2° and at its bottom is 138.2° as well. The DAD is thus 0°. (C) If the top of the figure in A is tilted forward and the bottom backward, then edge 23 is foreshortened. (D) If the top of the figure in C is tilted even farther forward, edge 23 appears as a point. The 138.2° angle between the near (thick) edges 24 and 21 is the dihedral angle about edge 23 at its top (vertex 2) end. The 180° angle between the far (thin) edges 36 and 35 is the dihedral angle about edge 23 at its bottom (vertex 3) end. The difference, a DAD of 41.8°, conveys broadening of the dihedral angle from the pentagon (near) end to the hexagon (far) end. The curved arrow on the left marks counterclockwise (negative) rotation of the far edge 36 from the near edge 24 about edge 23. The curved arrow on the right marks clockwise (positive) rotation of the far edge 35 from the near edge 21 about edge 23. The presence of equal and opposite rotations is a hallmark of an edge with pure DAD, that is, without any twist. (E) If the top of the figure in B is tilted forward and the bottom backward, then edge 23 is foreshortened. (F) If the top of the figure in E is tilted even farther forward, edge 23 appears as a point. The 138.2° angle between the near (thick) edges 24 and 21 is the dihedral angle about edge 23 at its top (vertex 2) end. The same 138.2° angle between the far (thin) edges 36 and 35 is the dihedral angle about edge 23 at its bottom (vertex 3) end. The difference is 0°, so this edge has no DAD. (G) Starting with the configuration in F, far (thin) edges 36 and 35 are both rotated counterclockwise, and edge 23 is said to acquire counterclockwise (negative) twist. The DAD is still zero. The left curved arrow marks the counterclockwise rotation of the left edges 36 from 24 about edge 23, and the right curved arrow marks the identical counterclockwise rotation of the right edges 35 from 21 about edge 23. The presence of identical rotations is the hallmark of an edge with pure twist. The dihedral angles between the front (thick) edges (138.2°) and between the back (thin) edges (138.2°) are unchanged, so DAD remains zero. (H) Starting with the configuration in D, if far (thin) edges 36 and 35 are both rotated counterclockwise, edge 23 is said to acquire counterclockwise (negative) twist. The DAD is still 41.8°. The left curved arrow marks a large counterclockwise rotation of the left edges 36 from 24 about edge 23, and the right curved arrow marks a small clockwise rotation of the right edges 35 from 21 about edge 23. The difference between the left and right rotations is the same as the difference between the left and right rotations in D because the difference represents the DAD, which is unchanged. The average of the two rotations is the twist. This edge has both DAD and twist.
