Skip to main content
PMC home page
The Journal of Physiology logoLink to The Journal of Physiology
. 2003 Sep 5;553(Pt 1):147–154. doi: 10.1113/jphysiol.2003.049759

Titin isoform variance and length dependence of activation in skinned bovine cardiac muscle

Norio Fukuda *, Yiming Wu *, Gerrie Farman †, Thomas C Irving †, Henk Granzier *
PMCID: PMC2343481  PMID: 12963792

Abstract

We have explored the role of the giant elastic protein titin in the Frank-Starling mechanism of the heart by measuring the sarcomere length (SL) dependence of activation in skinned cardiac muscles with different titin-based passive stiffness characteristics. We studied muscle from the bovine left ventricle (BLV), which expresses a high level of a stiff titin isoform, and muscle from the bovine left atrium (BLA), which expresses more compliant titin isoforms. Passive tension was also varied in each muscle type by manipulating the pre-history of stretch prior to activation. We found that the SL-dependent increases in Ca2+ sensitivity and maximal Ca2+-activated tension were markedly more pronounced when titin-based passive tension was high. Small-angle X-ray diffraction experiments revealed that the SL dependence of reduction of interfilament lattice spacing is greater in BLV than in BLA and that the lattice spacing is coupled with titin-based passive tension. These results support the notion that titin-based passive tension promotes actomyosin interaction by reducing the lattice spacing. This work indicates that titin may be a factor involved in the Frank-Starling mechanism of the heart by promoting actomyosin interaction in response to stretch.

When stretched within the physiological sarcomere length (SL) range, active tension of cardiac muscle increases several fold, and this intrinsic ability of cardiac muscle forms, at least in part, the basis for Frank-Starling's law of the heart (e.g. Allen & Kentish, 1985; Kentish et al. 1986; Lakatta, 1987). The cellular mechanism of the SL dependence of activation involves an increased sensitivity of tension to Ca2+ at increased SL (e.g. Hibberd & Jewell, 1982; Kentish et al. 1986), the molecular mechanism(s) of which remains elusive.

Recently it was proposed that passive tension generated by the giant muscle protein titin (also known as connectin) plays a role in SL-dependent activation (Cazorla et al. 1999, 2001; Fukuda et al. 2001). Here we critically tested this intriguing proposition with experiments that take advantage of differential expression of cardiac titin isoforms to provide an independent mechanism to vary titin-based passive tension. In the heart, small (N2B) and large (N2BA) titin isoforms are expressed in a species-specific and location-specific manner (Cazorla et al. 2000). N2B titin has a shorter extensible region than N2BA titin and, consequently, produces higher passive tension at a given SL, when compared to N2BA titin. In the bovine heart, the expression ratio of N2B titin to N2BA titin is approximately equal in the ventricle, whereas the atrium expresses predominantly N2BA titin, resulting in higher passive tension in the ventricle (Wu et al. 2000). Therefore, one may expect that SL-dependent activation will be more pronounced in ventricular than in atrial muscle.

In the current study, we compared SL-dependent activation of skinned bovine ventricular (BLV) and atrial (BLA) muscles. Results show that the SL dependence is markedly more pronounced in BLV, consistent with the higher passive tension in BLV. To explore the underling mechanism(s), we performed small-angle X-ray diffraction studies and investigated changes in interfilament lattice spacing (i.e. lateral separation of the thick and thin filaments) in BLV and BLA. We found that the lattice spacing decreases with increasing SL and that the decrease is more dramatic in BLV. These results provide support for the notion that titin-based passive tension is a factor in the Frank-Starling mechanism of the heart and that the mechanism may involve titin-based modulation of the lattice spacing.

A preliminary report has appeared in abstract form (Fukuda et al. 2002).

METHODS

Preparation of muscle

Bovine hearts (animals, ≈1.5 year) were obtained from local slaughterhouses. Muscle strips (diameter, 1-2 mm; length ≈10 mm) were dissected from the left atrium and ventricle, and were skinned in relaxing solution (5 mm MgATP, 40 mm Bes, 1 mm Mg2+, 10 mm EGTA, 1 mm dithiothreitol, 15 mm phosphocreatine, 15 u ml−1 creatine phosphokinase, 180 mm ionic strength (adjusted by K-propionate), pH 7.0) containing 1 % (w/v) Triton X-100 overnight at ≈3 °C (Wu et al. 2000). Muscles were then washed thoroughly with relaxing solution and stored for one month or less at -20 °C in relaxing solution containing 50 % (v/v) glycerol. Passive and active tensions were not changed during this period in either BLV or BLA (statistical tests failed to reveal a correlation between passive or active tension and storage duration (data not shown)). To prevent protein degradation, all solutions used in this study contained protease inhibitors (phenylmethylsulfonyl fluoride (PMSF), 0.5 mm; leupeptin, 0.04 mm; E64, 0.01 mm) (Wu et al. 2000).

Measurement of isometric tension

Small preparations (100-200 µm in diameter, ≈2 mm in length) were dissected from the strips described above for measurement of isometric passive and active tension (Wu et al. 2000). Tension was measured as described previously (Granzier & Irving, 1995; Wu et al. 2000). SL was measured by laser diffraction during relaxation (Wu et al. 2000; Granzier & Irving, 1995).

The muscle preparation was first immersed in relaxing solution (SL 1.9 µm). Just prior to contraction, the preparation was bathed in a low-EGTA (0.5 mm) relaxing solution (Fukuda et al. 2001). The preparation was then activated at pCa 4.5 to obtain maximal Ca2+-activated tension, followed by relaxation. The preparation was then activated at various submaximal pCa values (high to low pCa) and lastly at pCa 4.5 to construct the pCa-tension relationship (pCa adjusted by Ca/EGTA). Then, the preparation was stretched to SL 2.4 µm in relaxing solution and held at this length. The time-dependent decline in passive tension was barely noticeable 20 min after stretch (Fig. 1), at which time the preparation was activated at pCa 4.5, followed by determination of the pCa-tension relationship. Active tension was normalized by the maximal active tension at pCa 4.5 obtained at the end of the force-pCa protocol at each SL. pCa-tension relationships were fitted to the Hill equation (Fukuda et al. 2000). We employed the difference between the values of the mid-point (pCa50) of the pCa-tension relationship measured at SL 1.9 and 2.4 µm (i.e. ▵pCa50) as an index of the SL dependence of Ca2+ sensitivity of tension. The Hill coefficient, nH, was used as a measure of cooperativity. Experiments were all conducted at a relatively low temperature (12 °C), which reduced rundown of active tension during the time required to measure a full pCa-tension relationship (i.e. < 5 % and < 15 % at SL 1.9 and 2.4 µm, respectively). In another series of experiments, active tension was measured ≈1 min after stretching the muscle to SL 2.4 µm, i.e. at higher passive tension, compared to the lower passive tension that existed >20 min after stretch in the former experiment (see Fig. 1). In this protocol, a single activation-relaxation cycle was performed after which the muscle was released back to the slack length (SL 1.9 µm) and 30 min later the same protocol was repeated except that activation was at a different pCa. In this way we constructed the pCa-tension relationship, with each activation taking place at a similar level of high passive tension. The rundown of active tension in this type of experiment was < 20 % at SL 2.4 µm.

Figure 1. Changes in passive tension with time following stretch.

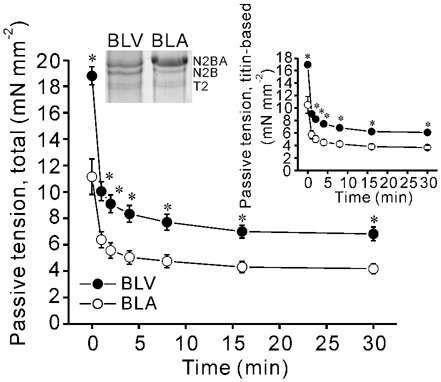
Figure 1

Open in a new tab

SL was increased from 1.9 to 2.4 µm and then held constant. Titin-based passive tension is shown in upper-right inset. * P < 0.05 compared with corresponding values for BLA. n = 5 for BLV and BLA. Expression profile of N2B and N2BA titins is also shown in upper left inset (T2, degradation product of titin).

For passive tension measurement, SL was increased from 1.9 to 2.4 µm at 0.1 muscle length s−1 and was held constant for 30 min (Granzier & Irving, 1995; Wu et al. 2000). The thick and thin filaments were then extracted by immersing the preparation in relaxing solution containing 0.6 m KCl and then in relaxing solution containing 1.0 m KI (Wu et al. 2000). Previous control experiments have demonstrated that KCl/KI treatment does not affect collagen-based passive tension (Wu et al. 2000), and thus we assumed that the remaining passive tension after KCl/KI treatment was collagen-based, whereas titin-based passive tension was determined as total passive tension minus collagen-based (KCl/KI-insensitive) tension (for additional details see Granzier & Irving, 1995; Wu et al. 2000).

Gel electrophoresis

To determine the titin isoform composition in BLV and BLA, specimens were solubilized and electrophoresed on 2-7 % acrylamide gradient gels. To study regulatory proteins as well as myosin light chains (MLCs), we used Laemmli gels (acrylamide: 3.5 % in stack and 15 % resolving gel). To study isoforms of myosin heavy chains (MHCs), we used gels with a 3.5 % acrylamide stack and 5 % resolving gel (run for 17 h at constant voltage of 60 V). Gels were scanned and digitized (Epson 800), and densitometry was performed using One-D-Scan (v. 2.03, Scanalytics Corporation). Proteins were identified by comigration with known standards.

Lattice spacing measurement

Small-angle X-ray diffraction experiments were performed on the BioCAT beamline 18ID at the Advanced Photon Source, Argonne National Laboratory (Irving et al. 2000). Samples were mounted between a force transducer and a servo motor in a small trough that allowed simultaneous collection of the X-ray patterns and viewing of the striation pattern using a 40 × objective on an inverted microscope equipped with a CCD video camera. The lattice spacing as well as SL was measured in relaxed preparations as described previously (Irving et al. 2000).

The BLV and BLA preparations were stretched at a constant velocity (0.1 muscle length s−1) from their slack length (SL 1.9-2.0 µm) to a pre-determined amplitude (resulting in SL ≈2.1, 2.2, 2.3 or 2.4 µm), held for various durations, followed by a release to the slack length. We measured the lattice spacing at the slack length and again ≈30 s after stretching muscles to each SL.

Low angle X-ray diffraction patterns were collected on a CCD-based X-ray detector. Diffraction spots are observed in the equatorial part of the diffraction pattern corresponding to Bragg reflections from planes through the unit cell of the myofilament lattice (Fig. 6A inset). The distance of the 1,0 reflection from the centre of the X-ray pattern (S) enables the perpendicular distance between the 1,0 lattice planes (d1,0) to be calculated using the Bragg relationship, which reduces to:

graphic file with name tjp0553-0147-mu1.jpg

where L is the distance from the muscle to the detector and λ is the wavelength of the X-radiation (1.03 Å). The d1,0 lattice spacing can, in turn, be converted to the inter-thick filament spacing by multiplying d1,0 by 2/√3.

Figure 6. Interfilament lattice spacing in BLV and BLA.

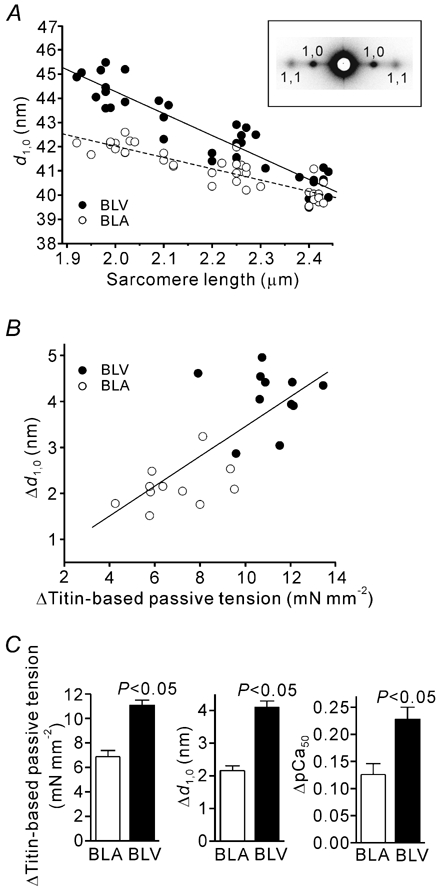
Figure 6

Open in a new tab

A, changes in d1,0 with SL in BLV and BLA. Lines are linear regression fits for BLV (coninuous line) (y = −9.06x + 62.36 (r2 = 0.865, P < 0.0001)) and BLA (dashed line) (y = −4.66x + 51.35 (r2 = 0.783, P < 0.0001)). The slope of the linear regression line was significantly (P < 0.001) greater in BLV than in BLA. Eleven preparations were used (BLV and BLA). Inset, X-ray patterns from BLA at SL 2.4 µm showing sharp equatorial reflections. B, linear regression analysis of the relation between titin-based passive tension and d1,0 when SL was increased from the slack length (1.9-2.0 µm) to ∼2.4 µm. ▵d1,0, difference in d1,0 at the slack length and SL ∼2.4 µm. A significant correlation is present (y = 0.33x + 0.19 (r2 = 0.572, P < 0.0001)). C, ▵Titin-based passive tension, ▵d1,0 and ▵pCa50 (same as in Fig. 2A inset) in BLV and BLA. All measurements were made at quasi steady-state passive tension.

Statistics

Significant differences were assigned using the paired or unpaired Student's t test as appropriate. Regression analysis was performed using the least-square method. All data are expressed as mean ± s.e.m., with n representing the number of muscles. Statistical significance was assumed to be P < 0.05.

RESULTS

SL dependence of activation

BLV expresses similar amounts of both N2B and N2BA titins, while BLA predominantly expresses N2BA titin (Fig. 1 inset). Both total passive tension and titin-based passive tension are significantly higher in BLV than in BLA at the peak as well as quasi steady-state during stress-relaxation (Fig. 1). The differences in quasi steady-state passive tension were maintained throughout the force-pCa protocol (Fig. 2A bottom). Because of stress-relaxation, the quasi steady-state tension is less than peak tension in both BLV and BLA. The values of peak passive tension were similar in both types of muscle to those reported in our previous study (see Wu et al. 2000). Because passive tensions of BLV and BLA are very different, these preparations are well suited to study the effect of titin-based passive tension on active tension. At SL 1.9 µm, Ca2+ sensitivity was slightly higher in BLV than in BLA, but this difference was not significant (see also Table 1). At SL 2.4 µm, BLV was significantly more sensitive to Ca2+, and consequently, ▵pCa50 was markedly more pronounced in BLV (Fig. 2A inset). No significant differences were observed in nH between BLV and BLA at both SLs (Table 1). Maximal tension was similar in BLV and BLA at SL 1.9 µm (Table 1). Maximal tension increased upon increase in SL in both BLV and BLA, with the magnitude being significantly (P < 0.05) greater in BLV (increase was 18.21 ± 1.85 vs. 9.48 ± 2.18 mN mm−2).

Figure 2. SL dependence of Ca2+ sensitivity and passive tension.

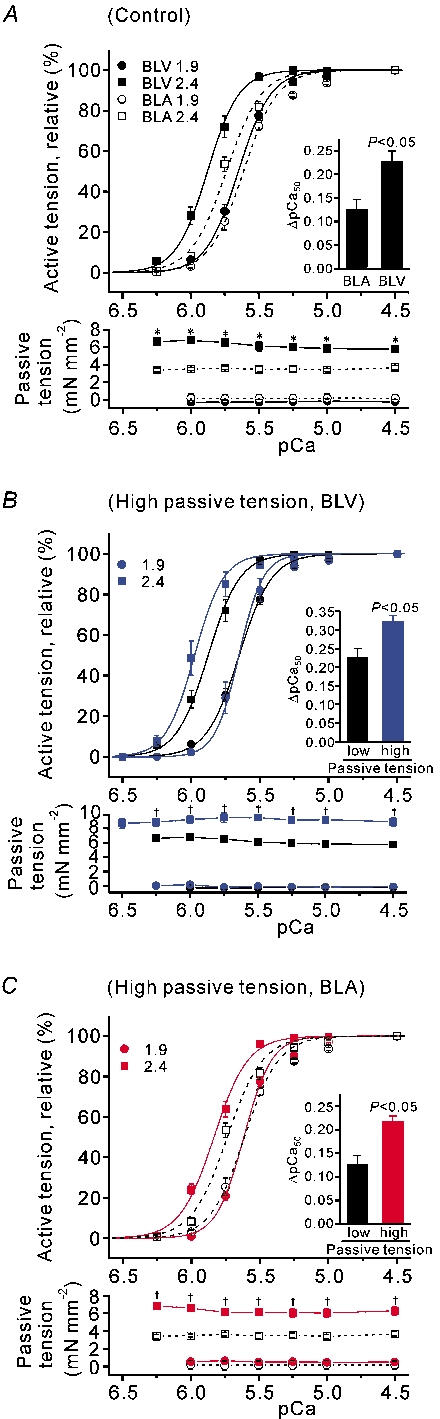
Figure 2

Open in a new tab

A, coninuous and dashed lines indicate BLV and BLA, respectively. Top, pCa-tension relationships in BLV and BLA at SL 1.9 and 2.4 µm. Inset, ▵pCa50. Bottom, passive tension (total) just prior to activating muscle at each pCa. Note that changes in passive tension throughout the protocol are small. * P < 0.05 compared with corresponding values for BLA at SL 2.4 µm. B, top, SL dependence of Ca2+ sensitivity at high passive tension in BLV (shown in blue; black symbols and lines are taken from A (BLV) for comparison). Inset, ▵pCa50 (low passive tension, taken from A (BLV)). Bottom, passive tension (total) just prior to activating muscle at each pCa. ‡ P < 0.05 compared with corresponding values obtained in A for BLV at SL 2.4 µm. C, same as in B for BLA. pCa-tension relationships and passive tension changes are shown in red (black symbols and lines are taken from A (BLA) for comparison). ‡ P < 0.05 compared with corresponding values obtained in A for BLA at SL 2.4 µm.

Table 1.

Summary of passive tension, maximal Ca2+-activated tension, pCa50 and nH under various conditions

SL (μm) Passive tension (mN mm−2) Maximal tension (mN mm−2) PCa50 nH
Fig. 2A (control) BLV(n = 8) 1.9 ˜0 21.36 ± 2.18 5.65 ± 0.01 3.66 ± 0.34
2.4 6.71 ± 0.42* 39.57 ± 3.10* 5.88 ± 0.03* 3.77 ± 0.13
BLA (n = 8) 1.9 ˜0 17.61 ± 1.17 5.61 ± 0.02 3.50 ± 0.36
2.4 3.50 ± 0.24 27.08 ± 2.69 5.74 ± 0.02 3.36 ± 0.26
Fig. 2B (high PT) BLV(n = 6) 1.9 ˜0 24.75 ± 2.05 5.65 ± 0.03 4.98 ± 0.53
2.4 9.22 ± 0.62† 48.94 ± 3.20† 5.98 ± 0.04† 4.13 ± 0.38
Fig. 2C (high PT) BLA (n = 5) 1.9 ˜0 21.05 ± 1.24 5.62 ± 0.01 4.30 ± 0.30
2.4 6.80 ± 0.41† 35.84 ± 2.45† 5.84 ± 0.02† 3.49 ± 0.12
Open in a new tab

Maximal tension was obtained by activating muscle at pCa 4.5 prior to construction of the pCa–tension relationship at each SL (passive tension, just prior to pCa 4.5 activation). High PT, high passive tension.

*

P < 0.05 compared with corresponding values for BLA.

†

P < 0.05 compared with corresponding values obtained in experiments under the control condition (Fig. 2A).

We then performed experiments ≈1 min after the stretch was completed and in which passive stress was substantially higher than the quasi steady-state passive tension (Fig. 2B and C for BLV and BLA, respectively). At SL 2.4 µm, the pCa-tension relationship was shifted to the left in each tissue, compared with that at low passive tension (Fig. 2A), showing increased Ca2+ sensitivity with passive tension. This resulted in a significant increase in ▵pCa50 (see insets of Fig. 2B and C). Increasing passive tension did not significantly affect nH in either BLV or BLA (Table 1). Maximal tension was significantly enhanced upon increase in passive tension (Table 1). This results in a more pronounced SL dependence of maximal tension where the values for the difference in maximal tension at SL 1.9 and 2.4 µm were 24.18 ± 2.44 and 14.80 ± 2.20 mN mm−2 in BLV and BLA, respectively, with each significantly (P < 0.05) greater than the corresponding value obtained at low passive tension (see above).

We found a positive correlation between titin-based passive tension and active tension for both Ca2+ sensitivity of active tension (Fig. 3A) and maximal active tension (Fig. 3B). When the SL dependence of Ca2+ sensitivity was indexed by ▵[Ca2+]50 (i.e. difference in [Ca2+] required for half-maximal activation at SL 1.9 and 2.4 µm), a significant correlation with titin-based passive tension was obtained as well (data not shown). These results support the notion that SL-dependent activation is a function of passive tension generated by titin.

Figure 3. Effect of titin-based passive tension on SL-dependent activation.

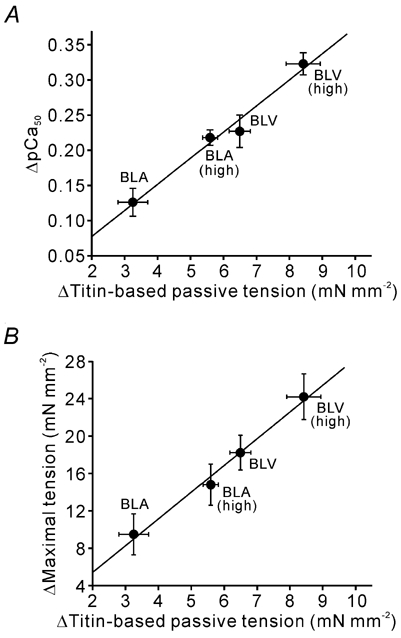
Figure 3

Open in a new tab

Data taken from Fig. 2 and Table 1. Lines are linear regression lines. Titin-based passive tension was obtained as described in Methods. ▵Titin-based passive tension, difference in titin-based passive tension at SL 1.9 and 2.4 µm. Passive tension data in Table 1 were used for analysis. Passive tension measured immediately after stretch is indicated as ‘high’. A, effect of titin-based passive tension on Ca2+ sensitivity (y = 0.037x + 0.003 (r2 = 0.979, P < 0.01)). B, effect of titin-based passive tension on maximal Ca2+-activated tension (y = 2.857x − 0.294 (r2 = 0.989, P < 0.005)).

Isoforms of thick and thin filament-based proteins

Isoform differences of thick and/or thin filament-based proteins could conceivably confer different SL-dependent activation properties in BLV and BLA. To investigate the expression profiles of thick and thin filament-based proteins, we conducted SDS-PAGE analyses.

MHC from BLV migrated faster than that from BLA (Fig. 4A), suggesting that β-MHC predominates in BLV, and α-MHC predominates in BLA (consistent with findings of others; see Cummins & Lambert, 1986; Reiser & Kline, 1998). Troponin T (TnT), troponin I (TnI), troponin C (TnC) and tropomyosin (Tm) were similarly expressed in BLV and BLA (Fig. 4B), consistent with previous findings (Humphreys & Cummins, 1984; Wilkinson & Taylor 1986). Myosin-binding protein C was also similarly expressed in BLV and BLA (data not shown). As reported previously (Cummins & Lambert, 1986), ventricular and atrial MLCs (ventricular, VLC-1 and VLC-2; atrial, ALC-1 and ALC-2) were predominantly expressed in BLV and BLA, respectively. However, it should be stressed that the expression profile of MLCs is promiscuous in that small amounts of ALC-1 and ALC-2 (VLC-1 and VLC-2) are co-expressed in BLV (BLA).

Figure 4. SDS-PAGE analysis showing isoforms of thick and thin filament-based proteins in BLV and BLA.

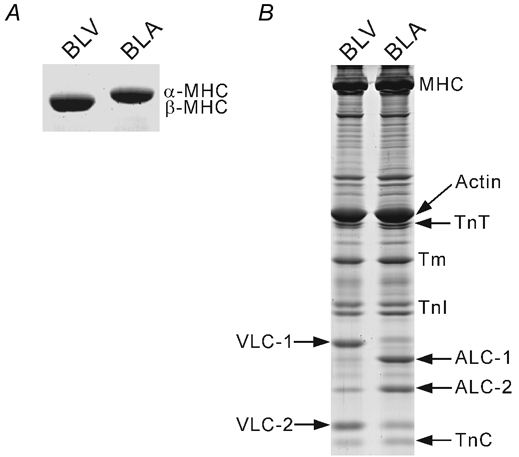
Figure 4

Open in a new tab

A, 5 % acrylamide gel showing separation of MHC in BLV and BLA. B, 15 % acrylamide gel showing regulatory proteins as well as MLCs in BLV and BLA.

By using the natural variation of the expression of MLCs, we examined whether the isoform variance of MLCs underlies the differing levels of SL-dependent activation in BLV and BLA. As shown in Fig. 5, the expression ratio of atrial to ventricular MLCs in BLV varies from heart to heart, from ≈0 % to up to ≈20 % for MLC-1 and from ≈2 % to up to ≈30 % for MLC-2. No correlation was found for either MLC-1 or MLC-2 in both Ca2+ sensitivity and maximal tension (Fig. 5), suggesting that differences in expression of MLCs do not underlie different levels of SL-dependent activation in BLV and BLA.

Figure 5. Expression ratio of atrial MLC to ventricular MLC plotted against ▵pCa50 (A and B) and ▵maximal tension (C and D) in BLV preparations.

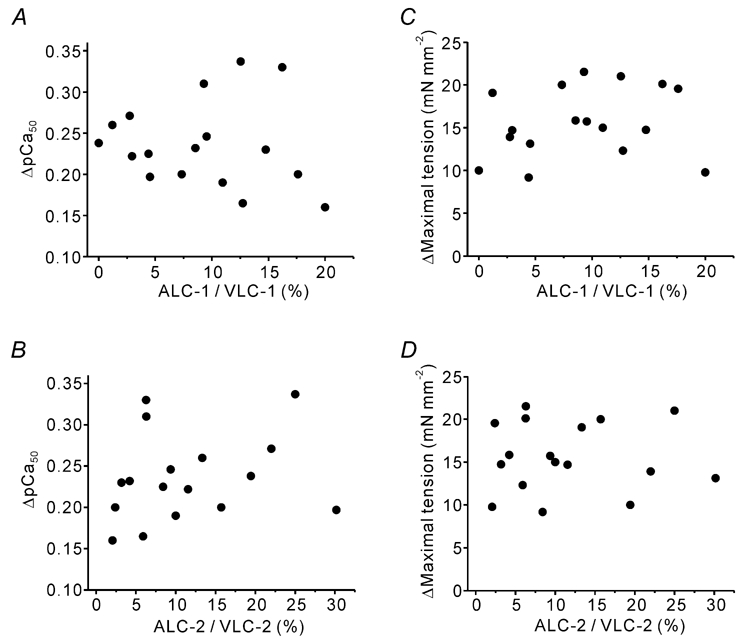
Figure 5

Open in a new tab

Preparations were obtained from seven different hearts. No correlation exists between parameters.

Lattice spacing

Earlier it was proposed that titin-based passive tension may reduce the lattice spacing and that this may explain the effect of titin on Ca2+ sensitivity (Cazorla et al. 2001). Here we tested whether the lattice spacing responds differently to stretch in BLV and BLA, tissues with high and low titin-based passive tension, respectively. We found that d1,0 decreased linearly with SL over the SL range from 1.9 to 2.4 µm in BLV and BLA, and that the slope of the SL-d1,0 relationship was significantly steeper in BLV than in BLA (Fig. 6A; see inset for an example of a diffraction pattern). As shown in Fig. 6B, a significant correlation exists between ▵titin-based passive tension and ▵d1,0 (when SL was varied from the slack length to 2.4 µm), suggesting that, as found previously in skeletal muscle (Higuchi & Umazume, 1986), the lattice spacing responds to titin-based passive tension. A summary of ▵titin-based passive tension, ▵d1,0, and ▵pCa50 for BLV and BLA (Fig. 6C) shows that each parameter is significantly greater in BLV than in BLA.

DISCUSSION

The Frank-Starling mechanism has as its cellular basis the phenomenon of SL-dependent activation of cardiac muscle. Here we have shown that titin-based passive tension has a profound effect on SL-dependent activation, implicating it as a factor in the Frank-Starling mechanism. Our experiments revealed that the SL dependence of Ca2+ sensitivity and maximal tension is more pronounced in BLV than in BLA, tissues that have high and low levels of passive tension, respectively. When passive tension is increased by modulating the pre-history of stretch, the SL dependence of activation responds accordingly. X-ray diffraction experiments demonstrated that titin-based passive tension affects interfilament lattice spacing, providing a possible mechanism by which titin may modulate active tension.

A striking difference between BLV and BLA is the different titin isoform composition and the ensuing difference in titin-based passive tension. However, differences also exist in the isoform expression profile of contractile proteins, any of which could potentially underlie differences in SL-dependent activation. First, β-MHC and α-MHC are predominantly expressed in the ventricle and atrium of adult cow, respectively (Fig. 4A; Cummins & Lambert, 1986; Reiser & Kline, 1998). However, considering the result of an earlier study (Akella et al. 1995) that conversion of α-MHC to β-MHC in the hypothyroid rat model does not significantly change the SL dependence of Ca2+ sensitivity, it seems unlikely that the difference in SL-dependent activation in BLV and BLA can be explained by MHC expression differences alone. In addition to MHC isoform differences, the MLC expression profile of the adult bovine ventricle and atrium varies (Fig. 4B) (Cummins & Lambert, 1986), and the role of MLCs in SL-dependent activation has yet to be reported. However, the present finding (Fig. 5) that there is no correlation between the ratio of atrial to ventricular MLCs and SL dependency (either ▵pCa50 or ▵maximal tension) suggests that the isoform variance of MLCs is unlikely to account for different SL-dependent activation in BLV and BLA. For the Tn complex and Tm we found no major differences in BLV and BLA (Fig. 4B; Humphreys & Cummins, 1984; Wilkinson & Taylor 1986). Therefore, it appears that isoform variance of thick and thin filament-based proteins does not underlie differences in SL-dependent activation in BLV and BLA.

The finding that the SL dependence of Ca2+ sensitivity and maximal tension is enhanced when measured immediately after passive stretch in both BLV and BLA (Fig. 2B and C) supports the notion that it is titin and not differences in thick and thin filament-based proteins that modulates the SL dependence of Ca2+ activation (see Fig. 3). This is because thick and thin filament-based proteins are the same regardless of the level of passive tension. Fukuda et al. (2001) reported that Ca2+ sensitivity is not significantly altered after titin degradation by trypsin in rat ventricular trabeculae while maximal tension was markedly reduced. The lack of an effect of passive tension on Ca2+ sensitivity in this previous work may be due to species differences (rat vs. cow), differences in the method used to vary passive tension (i.e. proteolysis was employed in Fukuda et al. (2001)) and/or other differences in experimental protocol. Under the present experimental conditions, however, Ca2+ sensitivity changes in response to a change in titin-based passive tension (Fig. 2 and Fig. 3A), supporting our conclusion that titin-based passive tension affects both Ca2+ sensitivity and maximal tension.

We previously proposed that titin modulates the lattice spacing and that this may be a mechanism by which titin influences SL-dependent activation (Cazorla et al. 2001). In the sarcomere, titin does not run parallel to the thin and thick filaments, but instead runs obliquely, because near the Z-line, titin binds to actin (Trombitas & Granzier, 1997) and in the A-band, it is intimately associated with myosin (Labeit et al. 1992; Trombitas et al. 1995). Thus, titin produces longitudinal as well as radial force, the latter of which reduces the lattice spacing (for details see Cazorla et al. 2001). With a stoichiometry of six titin molecules per half thick filament (Cazorla et al. 2000; Liversage et al. 2001) and experimentally measured titin-based passive tension and lattice spacing, we previously concluded that titin-based radial force is sufficiently large to compress the myofilament lattice in cardiac muscle (for details see Cazorla et al. 2001). In this earlier work we also showed that titin degradation by trypsin indeed results in lattice expansion. Currently, the relation between active tension and the lattice spacing is not well resolved, with Konhilas et al. (2002a,b; 2003) providing evidence in different experimental models that Ca2+ sensitivity and the lattice spacing are not well correlated. Preliminary studies, however, indicate that interpretation of the findings of Konhilas et al. (2002a,b; 2003) may be complicated by structural rearrangements of the myosin heads with changes in the lattice spacing so that the lattice spacing influences active tension only indirectly through the myosin head position relative to the thin filament (Farman et al. 2003). In any case, the present work clearly shows that the relaxed lattice spacing is under the influence of titin-based passive tension; i.e., SL-dependent changes in the lattice spacing were more pronounced in BLV than in BLA, consistent with differences in titin-based passive tension (Fig. 6). In accordance with this finding, during stress-relaxation (after SL increased from ≈1.9 to ≈2.4 µm; see Fig. 1), the lattice spacing was significantly expanded, albeit by a small amount, in both BLV and BLA (i.e., by 0.2-0.3 nm from ≈1 min to ≈20 min after stretch; data not shown). In each case, the SL dependence of active tension (both Ca2+ sensitivity and maximal tension) varied accordingly (see Fig. 2). These results support the idea that titin-based passive tension reduces the lattice spacing and thereby increases the likelihood of actomyosin interaction, resulting in increased active tension (e.g. note the similar difference in titin-based passive tension, the lattice spacing and Ca2+ sensitivity between BLV and BLA; Fig. 6C).

It is also worth considering that titin-based passive tension may influence actomyosin interaction by directly affecting cross bridge behaviour (Cazorla et al. 2001; Fukuda et al. 2001). Evidence for strain-dependent structural changes in the thick filament that led to an increase in the fraction of cross bridges in the disordered state in response to passive stretch has been reported for skeletal muscle (Wakabayashi et al. 1994). It seems reasonable, therefore, to assume that a passive tension-based mechanism that influences cross bridge interaction also exists in cardiac muscle and that it scales with passive stiffness, i.e. that the mechanism is more pronounced in BLV than in BLA. To test this proposal, careful two-dimensional X-ray diffraction studies are needed that assess cross bridge behaviour at low and high levels of passive tension.

In conclusion, our studies show that titin-based passive tension influences the SL dependence of Ca2+ sensitivity and maximal active tension in skinned bovine cardiac muscle. The underlying mechanism may involve titin-based modulation of interfilament lattice spacing, but other possibilities are not excluded. This work supports the view that titin is not only a passive mechanical spring but also a stretch sensor that promotes actomyosin interaction.

Acknowledgments

This work was supported by National Institutes of Health (NIH) Grant HL62881 (to H.G.) and American Heart Association (AHA) Grant 0120651Z (to N.F.). We would like to thank J. Henry, G. A. Kumar, and A. Joshi for help with X-ray data analysis and N. King for help with gel electrophoresis. Use of the Advanced Photon Source was supported by the US Department of Energy, Basic Energy Sciences, Office of Energy Research, under Contract No. W-31-109-ENG-38. BioCAT is a NIH-supported Research Center (RR08630).

REFERENCES

  1. Akella AB, Ding XL, Cheng R, Gulati J. Diminished Ca2+ sensitivity of skinned cardiac muscle contractility coincident with troponin T-band shifts in the diabetic rat. Circ Res. 1995;76:600–606. doi: 10.1161/01.res.76.4.600. [DOI] [PubMed] [Google Scholar]
  2. Allen DG, Kentish JC. The cellular basis for length-tension relation in cardiac muscle. J Mol Cell Cardiol. 1985;17:821–840. doi: 10.1016/s0022-2828(85)80097-3. [DOI] [PubMed] [Google Scholar]
  3. Cazorla O, Freiburg A, Helmes M, Centner T, McNabb M, Wu Y, Trombitas K, Labeit S, Granzier H. Differential expression of cardiac titin isoforms and modulation of cellular stiffness. Circ Res. 2000;86:59–67. doi: 10.1161/01.res.86.1.59. [DOI] [PubMed] [Google Scholar]
  4. Cazorla O, Vassort G, Garnier D, Le Guennec JY. Length modulation of active force in rat cardiac myocytes: is titin the sensor? J Mol Cell Cardiol. 1999;31:1215–1227. doi: 10.1006/jmcc.1999.0954. [DOI] [PubMed] [Google Scholar]
  5. Cazorla O, Wu Y, Irving TC, Granzier H. Titin-based modulation of calcium sensitivity of active tension in mouse skinned cardiac myocytes. Circ Res. 2001;88:1028–1035. doi: 10.1161/hh1001.090876. [DOI] [PubMed] [Google Scholar]
  6. Cummins P, Lambert SJ. Myosin transitions in the bovine and human heart. A developmental and anatomical study of heavy and light chain subunits in the atrium and ventricle. Circ Res. 1986;58:846–858. doi: 10.1161/01.res.58.6.846. [DOI] [PubMed] [Google Scholar]
  7. Farman GP, de Tombe PP, Irving TC. Radial head position in cardiac muscle affects calcium sensitivity. Biophys J. 2003;84:139A. [Google Scholar]
  8. Fukuda N, Kajiwara H, Ishiwata S, Kurihara S. Effects of MgADP on length dependence of tension generation in skinned rat cardiac muscle. Circ Res. 2000;86:e1–6. doi: 10.1161/01.res.86.1.e1. [DOI] [PubMed] [Google Scholar]
  9. Fukuda N, Sasaki D, Ishiwata S, Kurihara S. Length dependence of tension generation in rat skinned cardiac muscle: role of titin in the Frank-Starling mechanism of the heart. Circulation. 2001;104:1639–1645. doi: 10.1161/hc3901.095898. [DOI] [PubMed] [Google Scholar]
  10. Fukuda N, Wu Y, Farman G, Irving TC, Granzier H. Effect of isoform variance of titin on length-dependent activation in bovine cardiac muscle. Biophys J. 2002;82:398A. [Google Scholar]
  11. Granzier H, Irving TC. Passive tension in cardiac muscle: contribution of collagen, titin, microtubles, and intermediate filaments. Biophys J. 1995;68:1027–1044. doi: 10.1016/S0006-3495(95)80278-X. [DOI] [PMC free article] [PubMed] [Google Scholar]
  12. Hibberd MG, Jewell BR. Calcium- and length-dependent force production in rat ventricular muscle. J Physiol. 1982;329:527–540. doi: 10.1113/jphysiol.1982.sp014317. [DOI] [PMC free article] [PubMed] [Google Scholar]
  13. Higuchi H, Umazume Y. Lattice shrinkage with increasing resting tension in stretched, single skinned fibers of frog muscle. Biophys J. 1986;50:385–389. doi: 10.1016/S0006-3495(86)83474-9. [DOI] [PMC free article] [PubMed] [Google Scholar]
  14. Humphreys JE, Cummins P. Regulatory proteins of the myocardium: atrial and ventricular tropomyosin and troponin-I in the developing and adult bovine and human heart. J Mol Cell Cardiol. 1984;16:643–657. doi: 10.1016/s0022-2828(84)80628-8. [DOI] [PubMed] [Google Scholar]
  15. Irving TC, Konhilas J, Perry D, Fischetti R, de Tombe PP. Myofilament lattice spacing as a function of sarcomere length in isolated rat myocardium. Am J Physiol Heart Circ Physiol. 2000;279:H2568–2573. doi: 10.1152/ajpheart.2000.279.5.H2568. [DOI] [PubMed] [Google Scholar]
  16. Kentish JC, ter Keurs HEDJ, Ricciardi L, Bucx JJJ, Noble MIM. Comparison between the sarcomere length-force relations of intact and skinned trabeculae from right ventricle: influence of calcium concentrations on these relations. Circ Res. 1986;58:755–768. doi: 10.1161/01.res.58.6.755. [DOI] [PubMed] [Google Scholar]
  17. Konhilas JP, Irving TC, de Tombe PP. Myofilament calcium sensitivity in skinned rat cardiac trabeculae: role of interfilament spacing. Circ Res. 2002a;90:59–65. doi: 10.1161/hh0102.102269. [DOI] [PubMed] [Google Scholar]
  18. Konhilas JP, Irving TC, de Tombe PP. Length-dependent activation in three striated muscle types of the rat. J Physiol. 2002b;544:225–236. doi: 10.1113/jphysiol.2002.024505. [DOI] [PMC free article] [PubMed] [Google Scholar]
  19. Konhilas JP, Irving TC, Wolska BM, Jweied EE, Martin AF, Solaro RJ, de Tombe PP. Troponin I in the murine myocardium: influence on length-dependent activation and interfilament spacing. J Physiol. 2003;547:951–961. doi: 10.1113/jphysiol.2002.038117. [DOI] [PMC free article] [PubMed] [Google Scholar]
  20. Lakatta EG. Starling's law of the heart is explained by an intimate interaction of muscle length and myofilament calcium activation. J Am Coll Cardiol. 1987;10:1157–1164. doi: 10.1016/s0735-1097(87)80361-3. [DOI] [PubMed] [Google Scholar]
  21. Labeit S, Gautel M, Lakey A, Trinick J. Towards a molecular understanding of titin. EMBO J. 1992;11:1711–1716. doi: 10.1002/j.1460-2075.1992.tb05222.x. [DOI] [PMC free article] [PubMed] [Google Scholar]
  22. Liversage AD, Holmes D, Knight PJ, Tskhovrebova L, Trinick J. Titin and the sarcomere symmetry paradox. J Mol Biol. 2001;305:401–409. doi: 10.1006/jmbi.2000.4279. [DOI] [PubMed] [Google Scholar]
  23. Reiser PJ, Kline W. Electrophoretic separation and quantitation of cardiac myosin heavy chain isoforms in eight mammalian species. Am J Physiol. 1998;274:H1048–1053. doi: 10.1152/ajpheart.1998.274.3.H1048. [DOI] [PubMed] [Google Scholar]
  24. Trombitas K, Granzier H. Actin removal from cardiac myocytes shows that near Z-line titin attaches to actin while under tension. Am J Physiol. 1997;273:C662–670. doi: 10.1152/ajpcell.1997.273.2.C662. [DOI] [PubMed] [Google Scholar]
  25. Trombitas K, Jin JP, Granzier H. The mechanically active domain of titin in cardiac muscle. Circ Res. 1995;77:856–861. doi: 10.1161/01.res.77.4.856. [DOI] [PubMed] [Google Scholar]
  26. Wakabayashi K, Sugimoto Y, Tanaka H, Ueno Y, Takezawa Y, Amemiya Y. X-ray diffraction evidence for the extensibility of actin and myosin filaments during muscle contraction. Biophys J. 1994;67:2422–2435. doi: 10.1016/S0006-3495(94)80729-5. [DOI] [PMC free article] [PubMed] [Google Scholar]
  27. Wilkinson JM, Taylor RD. Expression of troponin T in atria and ventricles of avian and mammalian hearts. J Mol Cell Cardiol. 1986;18:291–297. doi: 10.1016/s0022-2828(86)80411-4. [DOI] [PubMed] [Google Scholar]
  28. Wu Y, Cazolra O, Labeit D, Labeit S, Granzier H. Changes in titin and collagen underlie diastolic stiffness diversity of cardiac muscle. J Mol Cell Cardiol. 2000;32:2151–2162. doi: 10.1006/jmcc.2000.1281. [DOI] [PubMed] [Google Scholar]

Articles from The Journal of Physiology are provided here courtesy of The Physiological Society

RESOURCES