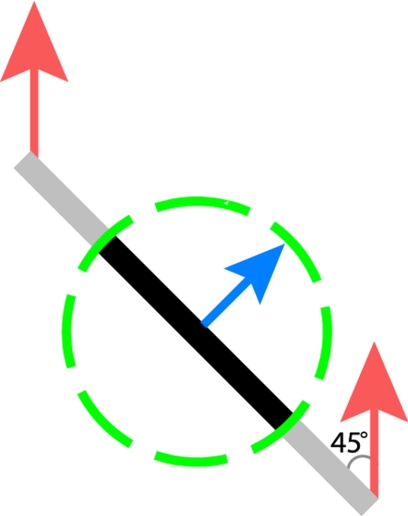
Fig. 1.

The geometry of the aperture problem. The red arrows show the actual motion of the bar; the blue arrow shows the motion of the bar as observed through the circular aperture (dashed circle). When an edge is observed through a circular aperture, the only available information about its direction of motion is along the axis perpendicular to its orientation. In other words, no time-varying information is conveyed along the parallel axis. In the example, a bar oriented at 45° and moving upwards at speed s seems to be moving up and to the right with speed s × sin(45°). Only the terminators (i.e., its corners) convey information about the bar's veridical direction of motion.