FIG. 4.

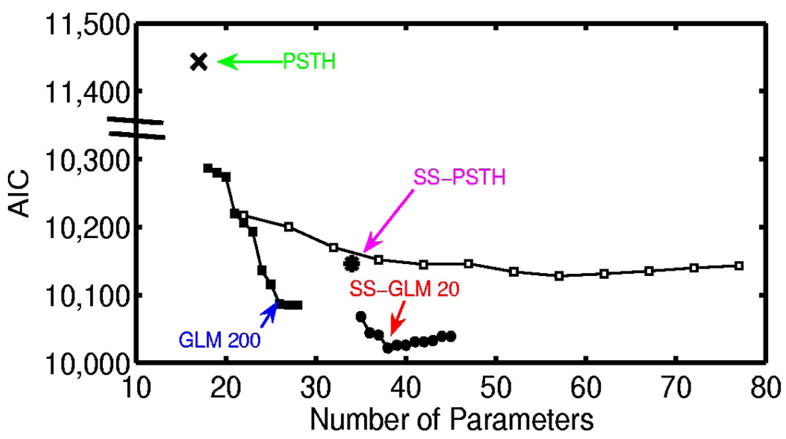
Akaike’s information criterion (AIC) (Eq. 13) for the analysis of the simulated neural spiking activity (Fig. 3) plotted as a function of the number of parameters for the candidate models: PSTH (×), generalized linear model (GLM) with coarse binning (black squares), state-space (SS)-PSTH (asterisk), GLM with fine binning (open squares), and SS-GLM with coarse binning (black circles). The model with the smallest AIC value provides the best approximating model in terms of minimum expected Kullbach–Leibler distance between the candidate model and the true model. The number of parameters is p, the dimension of ψ = (γ, θ₀, Σ) in Eq. 13. We construct the spike-history components of the GLM models (Eq. 4) by considering spike dependence going back 300 ms using coarse time binning and fine time binning. The coarse time bins were 1–5, 6 –10, 11–15, 16 –20, 21–30, 31–50, 51–100, 101–150, 151–200, 201–250, and 251–300 ms. The fine time bins consisted of sixty 5-ms bins. The best approximating model among the nonstate-space GLM models used coarse history dependence ≤ 200 ms defined as bins: 1–5, 6 –10, 11–15, 16 –20, 21–50, 51–100, 101–150, and 151–200 ms (GLM 200, blue). The best approximating model among all the models considered was the SS-GLM model with a 20-ms coarse history dependence defined as bins: 1–5, 6 –10, 11–15, and 16 –20 (SS-GLM 20, red). The model fits of the PSTH (green), GLM 200 (blue), SS-PSTH (pink), and SS-GLM 20 (red) are summarized in Table 1.