Figure 3.

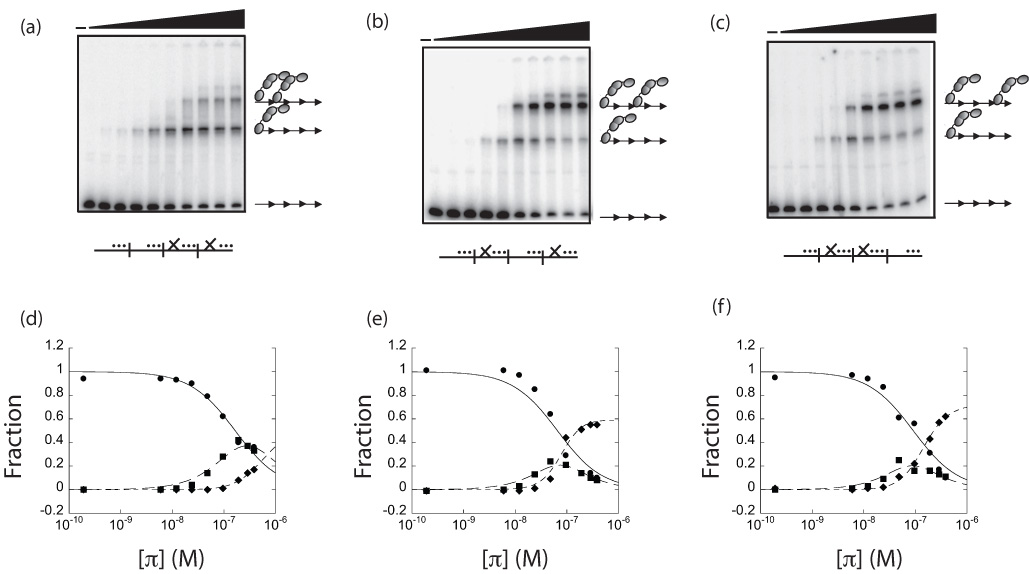
π binds with greater cooperativity to alternate iterons than adjacent iterons in vitro. (a–c) Gel shift titrations of purified π with the depicted probes. (_…) represents an iteron with mutations only in the 14^th, 17^th, and 18^th iteron, which is dimer proficient and monomer deficient. (X…) represents an iteron with mutation in the 7^th, and 9^th bp as well as the 14^th, 17^th, and 18^th, bp, which cannot bind monomers or dimers. The first lane is DNA only. Black triangles represent increasing levels of π•wt, starting with 3.12 ng (in a 15 □L reaction) and doubling for each lane. DNA probe preparation and gel shift titrations were carried out exactly as previously described³³ except that: 110 pg labeled iteron-containing probe was used in the binding reactions and Promega (Madison, WI) 6X loading dye was added prior to loading the gel. Arrows represent iterons. Gray double-ovals represent π dimers. (d–f) Quantification of gel shift titration data in panels (a–c), respectfully. The fraction of the total radioactivity as free DNA (circles), DNA containing a single π dimer (squares), and DNA containing two π dimers (diamonds) was quantified by fitting data from the gel shift titrations to the following equations using KaleidaGraph software (Reading, PA). The following equations were based on a modified statistical mechanical approach.³³,³⁵,⁴⁰

$${\mathrm{\theta }}_0={\mathrm{P}}_0+({\mathrm{P}}_{\text{max}}-{\mathrm{P}}_0)\mathrm{·}1/Z$$ (Eq. 1a)

$${\mathrm{\theta }}_1={\mathrm{P}}_0+({\mathrm{P}}_{\text{max}}-{\mathrm{P}}_0)·K_{1}L/Z$$ (Eq. 1b)

$${\mathrm{\theta }}_2={\mathrm{P}}_0+({\mathrm{P}}_{\text{max}}-{\mathrm{P}}_0)·K_2{L}^2/Z$$ (Eq. 1c)

θ_o,θ₁,and θ₂ are fractions of free DNA, single dimer complex, and two dimer complexes, respectively. P₀ and P_max are the baseline and maximum fraction for a given titration. Z is the binding polynomial and is equal to 1 + K₁L + K₂L². L is protein concentration, K₁=(k₁ + k₂) and K₂=(k₁k₂k₁₂). k₁ and k₂ are the binding affinity constants for the first iteron and the second iteron of the 2-iteron complex, and k₁₂ is the cooperativity coefficient describing the interaction of protein molecules occupying both sites. Broken, dotted and continuous lines correspond to the best fit of the data for equations (1a–1c), respectively. Once K₁ and K₂ were obtained from the least squares linear regression analysis, k₁₂ was derived by a few simple rearrangements, as described previously.³³

$$k_{12}=(\mathit{4}{K}_2)/{K_1}^2$$ (Eq. 2)