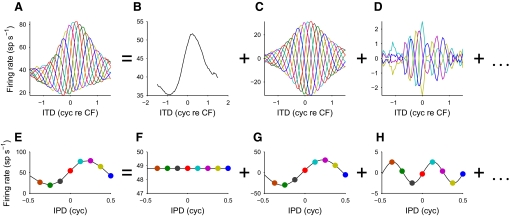
FIG. 3.

Fourier decomposition of a noise-delay function. The original noise-delay function (A) can be decomposed into several components (B–D) on the basis of the Fourier transform of the constituent IPD functions. For example, the responses to the 8 IPDs presented at zero ITD form a periodic function (E). Taking the Fourier decomposition of this yields several components: a DC offset (F), a fundamental (G), and a series of harmonics (of which only the second harmonic is shown, H). Each of these Fourier components is used to determine the IPD function at zero ITD in the corresponding noise-delay function component (B–D). Repeating this for the whole range of ITDs yields the complete components. Note that since the DC component is not modulated by IPD, the r₀(τ) component (B) will not show any IPD dependence. The data shown here are from a DNLL neuron with a characteristic frequency (CF) of 536 Hz.