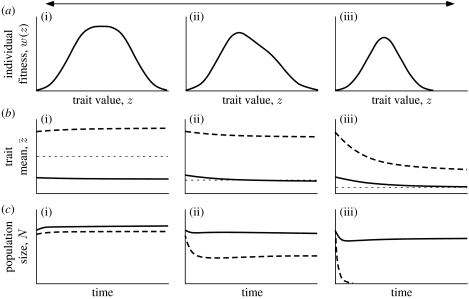
Figure 2.

Example of a stable range limit when the fitness function set by resources is unimodal. (a(i)–(iii)) Maximum individual fitness functions in the absence of inter- and intraspecific competitions (same as figure 1a,b). Trajectories of the population mean phenotypes (b(i)–(iii)) and population sizes (c(i)–(iii)) when environmental conditions are abruptly changed from those at the range centre to the alternatives assuming populations are initially close to the equilibrium expected at the range centre. The focal species, shown by the dashed curves, persists at the range centre (i) but goes extinct at the range edge (iii). The competitively dominant species, shown by the solid curves, persists everywhere. Dotted horizontal lines in (b) show the equilibrium for the trait mean if only one species is present. Computed using equations (2.1)–(2.5) in the text. Parameters are: a₁₁=a₂₂=1, a₁₂=0.7, a₂₁=1.1 (i.e. species 1 is the superior competitor that persists at the range limit of species 2), b₁₁=b₂₂=b₁₂=b₂₁=0.1, ${\sigma }_1^2$=${\sigma }_2^2$=4, $h_1^2$=$h_2^2$=0.5, D=0.02, θ=5, H₁=H₂=2 (i); H₁=2, H₂=1.2 (ii); H₁=2, H₂=0 (iii).