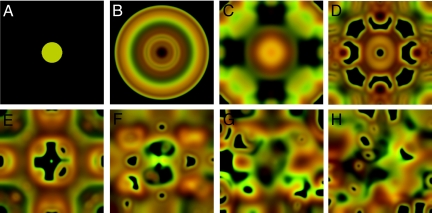
Fig. 1.

Chaotic pattern formation in spatial ecological public goods. A sequence of snapshots A–H demonstrates the spatial density distribution of cooperators (green) and defectors (red) over time (see Movie S1). The symmetry of the initial configuration A should be preserved in a deterministic system, but after some time it breaks down and disappears because of limitations of the numerical integration of Eq. 2. The exponential amplification of arbitrarily small disturbances characterizes chaotic systems. The initial configuration is a vacant L × L square (L = 400) with no flux boundaries and a homogeneous disk with radius L/10 in the center, where cooperators and defectors coexist at equal density (u_disk = v_disk = 0.1 and u = v = 0 elsewhere). The parameters of the ecological public goods are N = 8, d = 1.2, b = 1, r = 2.34, c = 1. The multiplication factor r lies slightly below the Hopf bifurcation r_Hopf = 2.3658, such that the fixed point Q is unstable, and in the absence of space, the population disappears. Diffusion of defectors is twice that of cooperators (D_C = 1, D_D = 2). The color brightness indicates the density of cooperators (green) and defectors (red). The snapshots are taken at times t = 0 (A), 1,200 (B), 1,800 (C), 2,000 (D), 2,200 (E), 2,600 (F), 2,800 (G), 4,000 (H). The numerical integration uses a spatial grid with dx = 0.8 and step size dt = 0.01.