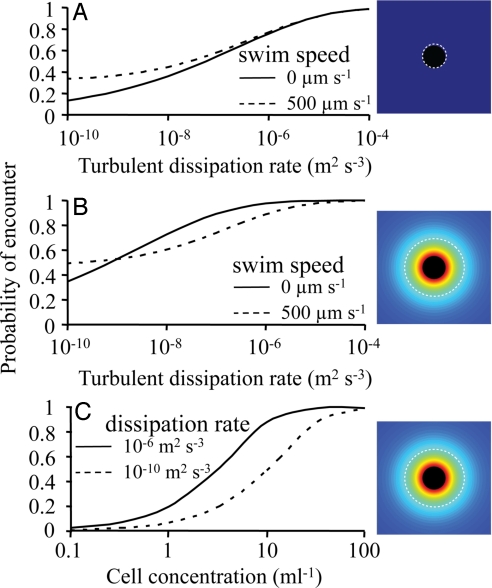
Fig. 2.

The probability of encounter between a susceptible cell and a population of cells producing an allelopathic compound (Eqs. 8–10). (A) Encounter radius is equal to the physical dimension of the cells. Probability of encounter is shown for swimming (500 μm s⁻¹, typical for protists) and nonmotile cells, and for a range of turbulent dissipation rates (from calm deep to well mixed surface waters). Concentration of allelopathic cells is 10 mL⁻¹. (B) Encounter probability when encounter is a function of the radius of the chemical envelope surrounding an allelopathic cell. The radius of the allelopathic envelope under flow conditions was determined by the concentration found three cell radii from the cell surface for nonflow conditions (Eq. 9). Concentration of allelopathic cells is 10 mL⁻¹. (C) Encounter probability as a function of the concentration of allelopathic cells for two turbulent dissipation rates. In all calculations the cell radius is 10 μm and the diffusion coefficient for the allelopathic compound is 5 × 10⁻¹⁰ m² s⁻¹. The broken, white line in the graphic Insets show the position of the encounter radius. Note that in B and C, the encounter radius represents the distance from the cell centre to where the concentration of a compound elicits an allelopathic effect in the absence of flow. This distance is then a function of cell motility and turbulent energy which act to erode the chemical envelope.