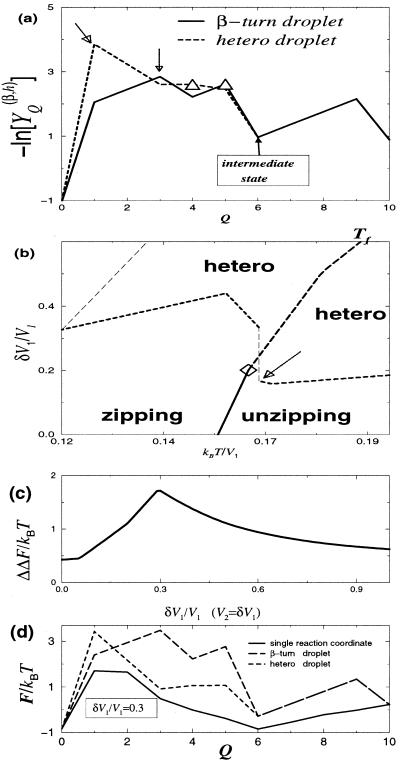
Figure 5.

(a) The typical free energy profiles for the hetero- and β-turn droplets at a T_f point indicated by the hollow arrow in b, in the double heterogeneity case. The barriers for the unfolding transition are indicated by Δ. Note that the folding barrier (the hollow arrow) is different from the unfolding one in the hetero-droplet. Here n = 10, γ = 3, ν = 10⁻⁴, and δV₁ = V₂ = 0.4V₁. (b) The typical crossover diagram. The solid curve, the ◊, and the long-dashed curve have the same meanings as in Fig. 4. Because the rate-limiting barriers are different for un/folding, there is a “discontinuity” (jump) in the crossover curve (indicated by the hollow arrow). (c) The difference ΔΔF = ΔF_d − ΔF_Q between the droplet analysis (ΔF_d) and the single reaction coordinate landscape (ΔF_Q) as a function of heterogeneity strength; this curve is based on data of the type shown in d for the specific value δV₁ = V₂ = 0.3V₁. Note that the droplet analysis, although dependent on a single coordinate Q, does not lump together all states with a given number of contacts.