Figure 2. The effects of stem cell parameters on the model predictions.
(A) We show the sensitivity of the model to the equilibrium frequency of cycling stem cells, 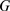 . Initially, the healthy cells are in equilibrium and there is one leukemic stem cell. As the leukemic cell population increases, the healthy cell population begins to decrease due to competition. Treatment is initiated once the leukemic cell burden has reached a threshold of
. Initially, the healthy cells are in equilibrium and there is one leukemic stem cell. As the leukemic cell population increases, the healthy cell population begins to decrease due to competition. Treatment is initiated once the leukemic cell burden has reached a threshold of  cells, at which point the leukemic cell burden begins to decline. Three scenarios are investigated: (i) the fraction of cycling stem cells is 10%,
cells, at which point the leukemic cell burden begins to decline. Three scenarios are investigated: (i) the fraction of cycling stem cells is 10%,  , using values of
, using values of 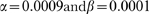 (blue lines), (ii) the fraction of cycling stem cells is 50%,
(blue lines), (ii) the fraction of cycling stem cells is 50%, 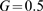 , with
, with  (red lines), and (iii) the fraction of cycling stem cells is 90%,
(red lines), and (iii) the fraction of cycling stem cells is 90%, 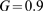 , with
, with 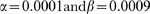 (green lines). Here
(green lines). Here  and
and 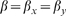 , with all other parameters as in Fig. 1. (B) We show the sensitivity of the model to
, with all other parameters as in Fig. 1. (B) We show the sensitivity of the model to  and
and  (i)
(i)  (blue lines), (ii)
(blue lines), (ii) 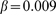 (red lines), and (iii)
(red lines), and (iii) 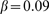 (green lines); in all cases
(green lines); in all cases  is chosen such that
is chosen such that 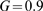 . Note the relative insensitivity of number of terminally differentiated leukemic cells to
. Note the relative insensitivity of number of terminally differentiated leukemic cells to  and
and 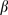 .
.

