Measurement of one and two bond N-C couplings in large proteins by TROSY-based J-modulation experiments

Abstract

Residual dipolar couplings (RDCs) between NC’ and NC^α atoms in polypeptide backbones of proteins contain information on the orientation of bond vectors that is complementary to that contained in NH RDCs. The ¹J_NCα and ²J_NCα scalar couplings between these atoms also display a Karplus relation with the backbone torsion angles and report on secondary structure. However, these N-C couplings tend to be small and they are frequently unresolvable in frequency domain spectra having the broad lines characteristic of large proteins. Here a TROSY-based J-modulated approach for the measurement of small ¹⁵N-¹³C couplings in large proteins is described. The cross-correlation interference effects inherent in TROSY methods improve resolution and signal to noise ratios for large proteins, and the use of J-modulation to encode couplings eliminates the need to remove frequency distortions from overlapping peaks during data analysis. The utility of the method is demonstrated by measurement of ¹J_NC’, ¹J_NCα, and ²J_NCα scalar couplings and ¹D_NC’ and ¹D_NCα residual dipolar couplings for the myristoylated yeast ARF1·GTPγs protein bound to small lipid bicelles, a system with an effective molecule weight of ~70 kDa.

1. Introduction

Couplings between pairs of spin ½ nuclei are a rich source of information for the structural characterization of proteins. The most common applications involve the use of three bond scalar couplings to restrict torsional angles about the central bond, such as the use of ³J between an amide proton and a Hα proton to restrict the ϕ angle between Cα and its directly bonded nitrogen [1,2,3,4], and the use of residual dipolar couplings (RDCs) to restrict orientations of bond vectors between pairs of spin ½ nuclei, such as that between an amide proton and an amide nitrogen of a ¹⁵N labeled protein [5,6,7,8]. Interestingly the ¹J_NCα and ²J_NCα scalar couplings are similar to three bond scalar couplings in their dependence on backbone dihedral angles, but depend on Ψ_i and Ψ_i−1 respectively, instead of ϕ [9,10,11]. Likewise, ¹⁵N-¹³C RDCs provide complementary information to ¹H-¹⁵N and other larger RDCs in restricting orientations of backbone structural elements [8,12,13]. The heteronuclear dipolar coupling Hamiltonian and the weak J-coupling Hamiltonian actually have the same spin part, so measurements of RDCs and J couplings usually utilize the same NMR experiments. While most experiments work well for measurement of the larger couplings, application becomes challenging when couplings are small compared to the spectral line widths. This is particularly an issue for ¹⁵N-¹³C couplings in larger proteins. Here we present a set of experiments that prove to be of particular value in these situations.

There has, of course, been considerable work devoted to the design of experiments for the measurement of scalar and dipolar couplings. In the most straightforward applications the couplings are measured from the frequency separations of lines in multiplet structures. The amide ¹⁵N-¹H J coupling of ~93Hz is usually larger than the ¹⁵N/¹H line-widths of proteins studied by solution NMR and can be determined at relatively high accuracy. The ¹⁵N-¹H RDCs are manifested as small changes in the doublet splitting and the slight increase in line-width in weakly orientated proteins do not severely degrade the measurement accuracy. The large ¹⁵N-¹H J coupling relative to the ¹⁵N transverse relaxation also makes possible the use of an IPAP type experiment, in which the doublet components are separated into different spectra in order to minimize spectral overlap without significant loss of sensitivity [14]. In contrast, the N-C couplings (¹J_NC’ ≈ 15Hz, ¹J_NCα ≈ 11Hz, ²J_NCα ≈ 7Hz) are small and measurement by frequency separation is usually limited to small proteins where sharp ¹⁵N lines are obtainable. The situation can be improved somewhat by allowing the J coupling to evolve longer than the chemical shift, thus scaling up the apparent splittings [15,16,17]. This does not solve the problem of doublet overlap because the line-width is often scaled up proportionally, however, the digitization error is reduced. IPAP type experiments can be used to eliminate overlap of peaks in ¹J_NC’ and ²J_NCα doublets [11] but IPAP is not suitable for the measurement of ¹J_NCα since there is no effective way of selectively creating anti-phase magnetization for N-C^α(i) without also creating anti-phase magnetization for N-C^α(i-1). The HNCO-based E.COSY type experiments can also be utilized to separate the ²J_NCα doublets through an extra C’ dimension subject to a much larger ¹J_C’Cα coupling[17]. However, due to the absence of a significant C’(i-1)-C^α(i) coupling, the E.COSY principle is inapplicable to the effective separation of the ¹J_NCα doublets and therefore its measurement is still limited by the ¹⁵N linewidth. Another complication associated with small J-couplings arises when the RDCs between pairs of atoms become comparable in size to their J couplings; this makes RDC sign and size determination ambiguous. In this case, E.COSY style experiments [18] can be employed if one of the weakly coupled spins is coupled to a third spin with a constant of known sign. The sign and size of an RDC can also be determined through variable angle sample spinning that scales anisotropic couplings but keeps scalar couplings unchanged [19].

As an alternative to frequency-based methods, J couplings can be obtained by intensity-based methods, including quantitative J-correlation [4,22] and J-modulation [10,20,21,24] experiments. The main difference between the two is that in the J-correlation method, the coupling is obtained from the ratio of two distinct functions correlated by J-coupling after a fixed through-coupling transfer period, while in the J-modulation method, only a single J-modulated function is recorded but at multiple transfer times. Because of its simplicity, the J-correlation method is widely used in 3-D based experiments. J-modulation is more often used in 2-D based experiments where a large number of modulation time points can be taken within a reasonable amount of spectrometer time. Because the coupling of interest is usually over-determined, the J-modulation experiment offers higher precision [21]. It also has the advantage that errors in derived couplings can be easily estimated based on the quality of multiple-point fitting. The intensity-based methods are applicable as long as chemical shift differences are larger than line-widths, regardless of the size of couplings. Thus they are widely used for the measurement of small homo- and heteronuclear couplings [10,20,22,23]. However, since longer modulation delays are necessary in order to measure small couplings, sensitivity loss from transverse relaxation degrades the performance. This is particularly a problem for large proteins and actually produces a similar problem to that encountered in frequency domain experiments when line widths approach the size of couplings to be measured.

The destructive interference between ¹H-¹⁵N dipolar interaction and ¹⁵N CSA can significantly improve spectral resolution and enhance sensitivity for experiments involving long ¹⁵N transverse relaxation periods [25,26]. However, it is difficult to exclusively utilize the ¹⁵N TROSY component during the measurement of NH couplings. For example, in frequency separated experiments, at least one of the lines in the multiplet structure is contaminated by the fast anti-TROSY relaxation [14,27,28], while in J-modulated experiments, it is hard to avoid a proton π pulse that exchanges TROSY and anti-TROSY magnetizations [21]. In contrast, for the measurement of N-C couplings, the slow ¹⁵N TROSY relaxation can be utilized during the entire J-modulation delay; this allows the use of the long delay times needed for accurate measurement.

The experimental scheme presented here is directed at the measurement of small ¹⁵N-¹³C couplings in large proteins. The sequence is basically a constant/semi-constant time ¹H-¹⁵N TROSY experiment [29] with an ¹⁵N evolution period modified to allow modulation by selected ¹³C couplings for various periods of time. The sensitivity is further enhanced by sharing ¹⁵N chemical shift evolution with the J-modulation periods. For the initial time points where the J-modulation periods are shorter than the time required for ¹⁵N chemical shift evolution, a semi-constant time evolution is automatically adapted. We also choose to collect a reference spectrum for every modulation delay, in which J couplings are refocused so that spectral intensity is only modulated by relaxation. The peak intensity ratio between the J-modulation spectrum and the reference spectrum eliminates the contribution from relaxation. The latter approach introduces characteristics of both J-correlated and J-modulated methods in that a reference function is employed to cancel the relaxation effects and multiple delays are used for non-linear fitting. In addition it eliminates complications due to line widths varying with J-modulation periods in the semi-constant time version, it improves fitting accuracy by removing the relaxation rate as a floating parameter, and it allows more flexibility in combining data from different experiments as may occur with unstable samples.

The procedure is illustrated with data on a GDP/GTP switch protein that is involved in vesicle trafficking, namely yARF1·GTP [29]. ARF1 is a 21 kDa member of the RAS super family. It is N-terminal myristoylated, and exposure of this myristoyl chain, in addition to an N-terminal amphipathic helix, is believed to modulate membrane association. We recently reported the solution structure for the GDP bound form of myristoylated yARF1 [31]. Here a bicelle associated GTPγs bound form of the myristoylated protein is used. The effective molecular weight of the complex is estimated to be 70 kDa, a large system that poses a good test for the pulse sequence.

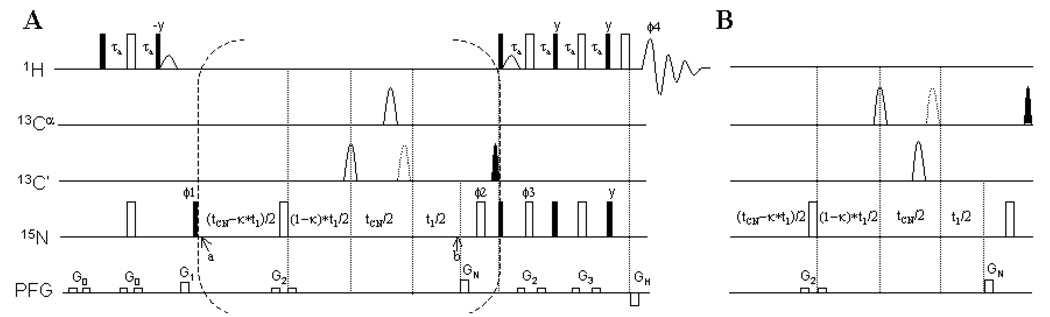
2. Pulse Sequence Design

Figure 1A shows the pulse sequence for measurement of ¹J_NC’ coupling. The first four pulses on protons and two on nitrogen encompass a period in which an INEPT transfer of proton magnetization to nitrogen occurs. Between points a and b, within the brackets, ¹⁵N chemical shift evolves for t_1, and ¹³C J coupling evolves for t_CN in the coupling modulation experiment. In the ¹³C decoupled (reference) experiment the central 180° pulse is moved to the end of the t_CN period which leads to complete refocusing of N-C couplings during the entire period when ¹⁵N magnetization is transverse, and a reference spectrum having only transverse relaxation but no J-modulation during t_CN results. The 90° ¹³C pulse after point b creates ¹HN-¹³C multiple quantum coherence from ¹HN-¹³C coherence formed during the J-modulated experiment to ensure removal of artifacts from these components. The ¹⁵N in-phase (N_x/y ) to anti-phase (2N_x/yC_z) transfer through the relaxation interference between ¹⁵N-¹³C dipole-dipole interaction and ¹⁵N CSA is negligible, so no attempt was made to remove these contributions. Since C’ and C^α carbons have no directly bonded protons under perdeuteration conditions, their longitudinal relaxation rates are small enough to satisfy that ${(2\mathrm{\pi }{\mathrm{J}}_{\mathit{\text{NC}}})}^2»{(R_1^C)}^2$, so ¹⁵N transverse relaxation can be treated as the average of in-phase N_x/y and anti-phase 2N_x/yC_z relaxation rates during modulation delays for both J-modulated and reference spectra. The pulses following the bracketed segment, coupled with the phase cycling described in the figure legend accomplish quadrature detection and selection of the TROSY component of the ¹H-¹⁵N HSQC spectrum as described in the literature [29].

Figure 1.

Pulse sequences for the measurement of ¹J_NC’, ¹J_NCα, and ²J_NCα couplings. A) the sequence for measurement of ¹J_NC’. B) the sequence to replace the part inside the dashed bracket of (A) to allow simultaneous measurement of ¹J_NCα, and ²J_NCα couplings. Narrow solid and wide open bars represent 90° and 180° rf pulses, respectively. The pulses are x phased unless otherwise indicated. The scale factor κ is set to 1 if t_CN is longer than the maximal t₁ acquisition time t_1,max, otherwise it is set to t_CN/t_1,max . The C’ 180° and 90° sinc pulses are centered at 174ppm with a null point at 56ppm. The C^α 180° and 90° reburp pulses are centered at 56ppm covering a band width of 50ppm. The sinc water flip-back pulse has a duration of 946µs. The delay τ_a is 0.91/(4J_NH) ≈ 2.45 ms. The phase cycling is: Φ₁ = {x, -x}, Φ₂ = {2(x), 2(−x)}, Φ₃ = {x} and Φ₄ = {−x, x}. The gradient G_H and the phase of Φ₃ are inverted along with the echo/anti-echo acquisition. The z gradients are: G₀ = 1.8G/cm, 0.5ms; G₁ = 26.6G/cm, 1ms; G₂ = 3.6G/cm, 0.5ms; G₃ = 5.4G/cm, 0.5ms; G_N = 31.9G/cm, 2ms; G_H = 32.3G/cm, 0.2ms.

Simply swapping the selective pulses on C’ and C^α will allow simultaneous measurement of ¹J_NCα and ²J_NCα couplings (Figure 1B) instead of ¹J_NC couplings. In principle, it is trivial to make a pulse sequence based on the current scheme for simultaneous measurement of ¹J_NC’, ¹J_NCα, and ²J_NCα, e.g., by simultaneously inverting or exciting both C’ and C^α. However fitting coupling constants to modulation curves with a more complicated relationship involves additional parameters and could be less accurate.

Under the circumstances described above, the intensity ratio of the ¹³C J-modulated spectrum over the reference spectrum is:

$$\frac{I^{J\text{mod}}}{I^{\mathit{\text{ref}}}}=\{\begin{array}{cc}(1-r)+r·\text{cos}(\pi ·{}^1{J}_{{\mathit{\text{NC}}}^{\text{'}}}·t_{\mathit{\text{CN}}})\hfill & {}^1{J}_{{\mathit{\text{NC}}}^{\text{'}}}\hfill \\ {(1-r)}^2+r^2·\text{cos}(\pi ·{}^1{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}})·\text{cos}(\pi ·{}^2{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}})\hfill & \hfill \\ +r·(1-r)·(\text{cos}(\pi ·{}^1{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}}))+\text{cos}(\pi ·{}^2{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}}))\hfill & {}^1{J}_{{\mathit{\text{NC}}}^{\alpha }}\text{and}{}^2{J}_{{\mathit{\text{NC}}}^{\alpha }}\hfill \end{array}$$ (1)

where the expressions on the top and bottom describe respectively the intensity ratios from the ¹J_NC’ and ¹J_NCα-²J_NCα experiments. The factor r takes into account non-ideal ¹³C π pulses and incomplete ¹³C enrichment. It varies between 0 and 1, with 1 corresponding to an ideal case in which the efficiency of both ¹³C incorporation and π pulses is 100%. The details are discussed in Appendix A. To ensure that r always falls in the legal range between 0 and 1, the following equations, in which cos²(a) is substituted for r, were used during the actual fitting process:

$$\frac{I^{J\text{mod}}}{I^{\mathit{\text{ref}}}}=\{\begin{array}{cc}{\text{sin}}^2(a)+{\text{cos}}^2(a)·\text{cos}(\pi ·{}^1{J}_{{\mathit{\text{NC}}}^{\text{'}}}·t_{\mathit{\text{CN}}})\hfill & {}^1{J}_{{\mathit{\text{NC}}}^{\text{'}}}\hfill \\ {\text{sin}}^4(a)+{\text{cos}}^4(a)·\text{cos}(\pi ·{}^1{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}})·\text{cos}(\pi ·{}^2{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}})\hfill & \hfill \\ +{\text{sin}}^2(a)·{\text{cos}}^2(a)·(\text{cos}(\pi ·{}^1{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}})+\text{cos}(\pi ·{}^2{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}}))\hfill & {}^1{J}_{{\mathit{\text{NC}}}^{\alpha }}\text{and}{}^2{J}_{{\mathit{\text{NC}}}^{\alpha }}\hfill \end{array}$$ (2)

Obviously, Eq.(1) and Eq.(2) describe the same relationship except that the coefficients in Eq.(2) are automatically confined within the correct range. The intensity ratio between the J-modulated and reference experiments depends on some fixed experimental parameters, namely the ¹³C percentage, ¹³C π pulse efficiency and J couplings of interest, but is indifferent to conditions that can affect the absolute signal intensities or relaxation properties. As a result, experiments involving samples of different concentrations can be combined. Experiments collected at different B₀ fields may also be combined, provided the ¹³C inversion pulses are properly calibrated to ensure nearly identical inversion efficiency. The couplings between amide ¹⁵N and H^α/H^β in cases of incompletely perdeuterated or nondeuterated proteins are active only for a duration of t₁ due to the presence of an ¹⁵N π pulse inside the J-modulation delay. Thus these couplings do not cause intensity modulation but merely some line-broadening in the ¹⁵N dimension, which does not affect the J-modulated measurement of NC couplings.

Residual dipolar couplings (RDCs) share spin operators with the Hamiltonian for first order scalar coupling and simply add to the observed splittings from scalar couplings. Hence, equation 1 and equation 2 apply equally well to cases where RDCs contribute with J+D replacing expressions in J alone.

3. Experimental

3.1 Sample Preparation

A His-tagged version of yARF1 was expressed in a deuterated, ¹⁵N, ¹³C enriched medium as previously described [31]. It was purified on a Hisx6 column and the His-tag removed by treatment with thrombin. The protein was further purified with a Q-sepharose column. The purified yARF1 which was pre-loaded primarily with GDP was then converted to a GTPγs form, that is only soluble in the presence of lipids, using the following procedure: A lipid mixture of DMPC/DHPC was added from a 40% (w/v) stock ([DMPC]:[DHPC] (q) =0.25) to a solution of the isolated yARF1. The resulting sample contains ~0.8mM yARF1·GDP, 10% (w/v) DMPC/DHPC (q=0.25), 10mM K₂HPO₄-KH₂PO₄ (pH 7), 50mM NaCl, 10mM K₂SO₄, 2mM MgCl₂, 5% D₂O, and 5mM dithiothreitol. The slowly hydrolyzing GTP analog, GTPγs, was then added to 5mM and EDTA was added to 2mM to chelate Mg²⁺ and increase the nucleotide exchange rate. Three units of calf intestinal phosphatase was then added to cleave the released GDP molecules, and GDP molecules produced from GTPγs hydrolysis as the sample ages, thereby minimizing the re-formation of yARF1·GDP during NMR studies. This mixture was incubated for over 1 hour and an additional 2mM MgCl₂ was added, resulting in a final NMR sample that contains ~0.8mM yARF1, 5mM GTPγs, 10% (w/v) DMPC/DHPC (q=0.25), 3U calf intestinal phosphatase, 10mM K₂HPO₄-KH₂PO₄ (pH 7), 50mM NaCl, 10mM K₂SO₄, 4mM MgCl₂, 2mM EDTA, 5% D₂O, and 5mM dithiothreitol.

3.2 Data Acquisition and Processing

The ¹J_NC’, ¹J_NCα, and ²J_NCα couplings were measured for the GTPγs -bound, myristoylated yeast ARF1 protein (²D, ¹³C, ¹⁵N) associated with DMPC/DHPC (q=0.25) bicelles, in isotropic solution and in anisotropic poly-acrylamide gel medium [31]. The myr-yARF1·GTPγs·bicelle complex tumbles at 25°C with an effective rotational correlation time of ~ 30ns [33,31], which corresponds to a molecule weight of ~70 kDa. The experiments were conducted on a 900MHz Varian VNMRS spectrometer equipped with a cold probe. The ¹⁵N acquisition time is 35ms. The modulation delays varied from 10ms to 100ms in 10ms steps for all experiments. The number of scans was ramped up as the relaxation delay increased to generate signals of comparable S/N at each point. For isotropic conditions, 8 scans were used for 10 and 20ms modulation delays, 12 for 30 and 40ms, 16 for 50 and 60ms, 20 for 70 and 80ms, 28 for 90 and 100ms. For the anisotropic condition, the number of scans was doubled for the corresponding modulation delays. The isotropic and anisotropic experiments take ~24 and ~48 hours respectively. Data were processed with the software NMRPipe [34]. A cosine square apodization function was applied prior to zero filling and Fourier transformation.

3.2 Error Analysis

The error in I^Jmod/I^ref is propagated from the S/N values of the J-modulated and reference spectra by:

$$\sigma =\frac{I^{J\text{mod}}}{I^{\mathit{\text{ref}}}}·\sqrt{{\left(\frac{1}{S/N_{J\text{mod}}}\right)}^2+{\left(\frac{1}{S/N_{\mathit{\text{ref}}}}\right)}^2}$$ (3)

The spectral S/N is defined as the ratio of the peak intensity over the root-mean-square (RMS) of noise. To evaluate the errors of the J couplings, Monte Carlo simulations were conducted where Gaussian noise with a mean of 0 and a deviation of σ (Eq. 3) was added to I^Jmod/I^ref at each time point. The error of J coupling was estimated as the standard deviation of least-squares fitted J couplings from 500 Monte Carlo simulations. The RDC error is the sum of the couplings errors from isotropic and anisotropic media.

The errors of Karplus parameters in Eq. 4 were also estimated by 500 Monte Carlo simulations where Gaussian errors were added to the ¹J_NCα and ²J_NCα couplings.

4. Results

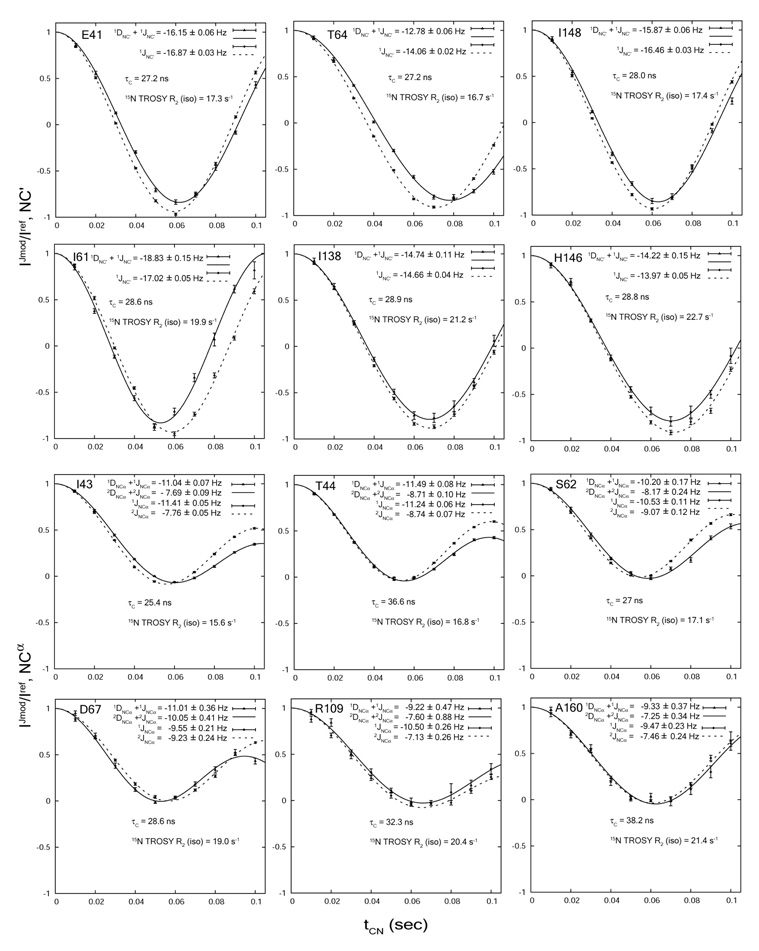
Figure 2 shows representative fits of couplings in isotropic (J) and anisotropic (J+D) media. The rotational correlation time τ_c at 25° and the ¹⁵N TROSY R₂ relaxation rates are also indicated for selected residues. For both ¹J_NC’ and ¹J_NCα, six representative fits are shown. The upper three fits are selected from those with RDC errors smaller than average, while the lower three fits are from those with RDC errors around average (0.19Hz for ¹D_NC’, and 0.67Hz for ¹D_NCα). The trend is clear that the measurement error is positively correlated with ¹⁵N TROSY R₂. It is also apparent that the RDC error comes mostly from the anisotropic measurement. The τ_c values indicate that these residues reside in the well-structured part of the protein rather than in the fast re-orientating regions. The J and residual dipolar couplings extracted based on Eq(1) are tabulated in Table 1. Also included in Table 1 are the back-calculated RDC’s based on the crystal structure of the non-myristoylated, GTP-bound mouse ARF1 protein without the N-terminal 17 residues (mARF1-GTP-Δ17) (PDB: 1o3y), which shares 76% sequence identity with the yeast ARF1 protein. The ²D_NCα couplings are expected to have a maximum value of only 0.5~0.6 Hz based on the ¹D_NC’ and ¹D_NCα couplings, so accurate measurement is inaccessible on this particular system. The measured ¹J_NC’, ¹J_NCα and ²J_NCα couplings range from 13.2 to 17.3 Hz, 8.3 to 13.1 Hz, and 5.8 to 10.4 Hz, while the typical ¹⁵N HSQC and TROSY linewidths in highly digitized spectra are 31 and 25 Hz respectively. Thus, these couplings are not expected to be well resolved using frequency separation experiments.

Figure 2.

Representative fits of intensity ratios for the measurement of ¹J_NC’ (A) and ¹J_NCα/²J_NCα (B)couplings in isotropic and aligned media. Residual dipolar couplings lead to differential periodicities between the isotropic and aligned conditions.

Table 1.

Scalar and dipolar couplings for yARF1-GTPγS in bicelles.

	1JNC’	1JNCa	2JNCa	1DNC’	1DNC’	1DNCa	1DNCa
L3	−14.98±0.01	−10.07±0.06	−8.12±0.07	0.51±0.02	*	−0.34±0.13	*
F4	−14.78±0.02	−10.38±0.08	−6.73±0.07	−0.66±0.04	*	0.13±0.17	*
S6	−14.89±0.02	−10.54±0.10	−7.03±0.08	0.17±0.06	*	−1.14±0.21	*
L8	−14.96±0.02	−9.88±0.13	−6.42±0.09	−0.88±0.04	*	0.73±0.27	*
F9	−14.43±0.02	−10.32±0.12	−6.67±0.10	0.09±0.05	*	0.21±0.26	*
S10	−14.93±0.02	−10.73±0.12	−7.33±0.14	0.64±0.08	*	−0.50±0.35	*
N11	−15.27±0.02	−10.72±0.09	−6.39±0.06	−0.08±0.03	*	0.25±0.19	*
L12	−15.44±0.02	−10.02±0.11	−6.80±0.11	−0.44±0.04	*	0.66±0.22	*
F13	−14.95±0.02	−10.70±0.12	6.85±0.10	0.15±0.05	*	−0.02±0.24	*
G14	−15.98±0.02	−11.89±0.07	−7.00±0.05	0.62±0.04	*	−0.60±0.14	*
N15	−15.46±0.01	−11.93±0.04	−8.87±0.04	−0.17±0.02	*	−0.92±0.06	*
R19	−14.44±0.03	−10.40±0.15	−8.97±0.17	1.07±0.10	1.05	−0.43±0.35	−0.36
I20	−14.97±0.05	−11.08±0.23	−8.29±0.21	−1.08±0.26	−0.89	-	-
M22	−14.30±0.11	−9.59±0.38	−9.59±0.39	-	-	−2.30±0.78	−1.34
G24	−15.34±0.21	-	-	-	-	-	-
L25	−16.22±0.16	−12.01±0.58	−9.81±0.60	-	-	-	-
G27	−14.99±0.04	−10.70±0.28	−9.75±0.31	0.97±0.17	0.68	0.70±0.61	0.03
A28	−15.49±0.03	−10.01±0.19	−6.35±0.14	−1.39±0.15	−0.98	-	-
G29	−15.19±0.30	-	-	-	-	-	-
K30	−14.70±0.71	-	-	-	-	-	-
T31	−14.96±0.09	−10.85±0.32	−6.64±0.24	0.14±0.41	0.24	-	-
V33	−15.11±0.07	−10.24±0.75	−5.76±0.27	0.11±0.17	0.33	0.04±1.29	−0.55
Y35	−14.45±0.07	−10.98±0.33	−6.59±0.24	−1.03±0.28	−0.77	-	-
K36	−15.47±0.06	−10.63±0.52	−6.37±0.35	−0.05±0.19	−0.1	-	-
L37	−14.30±0.05	−10.62±0.27	−6.96±0.23	0.55±0.24	0.27	-	-
L39	−14.84±0.09	−9.99±0.42	−6.63±0.41	-	-	0.23±1.67	0.51
G40	−15.34±0.06	−10.68±0.29	−6.32±0.20	0.30±0.18	0.17	-	-
E41	−16.87±0.03	−12.58±0.56	−6.55±0.22	0.71±0.08	0.69	−4.27±1.03	−1.49#
I43	−14.90±0.01	−11.41±0.05	−7.77±0.05	1.06±0.04	0.95	0.36±0.12	1.1
T44	−14.59±0.01	−11.24±0.06	−8.74±0.07	0.41±0.04	0.17	−0.25±0.14	−0.36
I46	−15.61±0.02	−11.97±0.08	−8.59±0.08	0.75±0.09	0.91	0.35±0.24	0.22
T48	−15.76±0.05	−10.39±0.31	−10.38±0.36	−0.33±0.22	−0.91	-	-
I49	−14.76±0.03	−9.51±0.17	−7.89±0.22	1.19±0.74	0.81	0.81±0.40	0.89
G50	−14.86±0.24	−10.44±0.51	−6.28±0.38	-	-	-	-
F51	−15.64±0.03	−12.82±0.07	−8.89±0.08	−1.08±0.10	−0.19	0.54±0.23	0.72
N52	−15.34±0.03	−11.77±0.16	−9.66±0.20	1.22±0.13	1.23	−0.35±0.40	0.47
T55	−15.44±0.02	−11.55±0.09	−9.06±0.11	−0.22±0.07	−0.92	−0.06±0.26	−0.06
V56	−14.73±0.02	−10.75±0.12	−8.43±0.13	0.92±0.07	0.58	1.02±0.31	0.83
Q57	−14.45±0.02	−11.15±0.09	−8.61±0.10	0.92±0.07	0.64	−0.56±0.19	−0.23
Y58	−14.72±0.03	−10.08±0.27	−8.96±0.31	0.41±0.10	0.39	0.93±0.49	0.72
K59	−14.84±0.02	−10.05±0.11	−8.12±0.13	1.33±0.07	1.2	−0.75±0.22	−0.36
I61	−17.02±0.05	−10.88±0.22	−6.71±0.17	−1.82±0.20	−0.92	0.73±0.76	0.86
S62	−13.94±0.02	−10.53±0.11	−9.05±0.12	1.02±0.08	0.91	0.33±0.28	0.29
F63	−14.56±0.03	−10.94±0.12	−8.29±0.14	−1.76±0.16	−1.69	0.31±0.51	0.13
T64	−14.06±0.02	−10.35±0.11	−8.63±0.14	1.28±0.08	1.13	0.81±0.37	0.56
D67	−14.24±0.03	−9.44±0.21	−9.36±0.24	−0.26±0.10	−0.41	−1.59±0.57	−1.77
V68	−16.47±0.05	−11.74±0.17	−7.69±0.14	1.07±0.14	0.41	0.50±0.44	0.91
G69	−13.76±0.08	-	-	0.16±0.26	0.31	-	-
G70	−14.26±0.35	-	-	-	-	-	-
Q71	−15.71±0.12	−11.67±0.56	−6.70±0.29	0.384±0.35	0.45	-	-
D72	−14.44±0.09	−10.35±0.37	−8.97±0.50	−0.62±0.30	−0.81	−0.05±1.24	0.61
R73	−14.47±0.12	−10.94±0.94	−7.23±0.61	0.79±0.38	0.43	-	-
S76	−14.86±0.10	−10.40±0.29	−6.40±0.26	-	-	−1.52±0.90	−0.89
R79	−14.89±0.23	-	-	-	-	-	-
H80	−14.51±0.12	−11.31±0.98	−6.11±0.73	-	-	-	-
Y81	−15.72±0.17	−10.63±0.46	−6.55±0.33	-	-	-	-
Y82	−15.23±0.08	−10.40±0.45	−6.56±0.43	-	-	1.41±1.30	0.65
N84	−15.39±0.02	−11.57±0.10	−8.19±0.10	-	-	-	-
T85	−16.12±0.10	-	-	−2.06±0.28	−1.7	-	-
E86	−14.87±0.06	−10.85±0.22	−7.97±0.24	0.93±0.23	1.13	-	-
G87	−15.85±0.11	−12.57±0.26	−7.52±0.25	−1.45±0.41	−0.85	-	-
V91	−14.53±0.11	−10.64±0.60	−8.41±0.60	−0.33±0.26	−0.01	−0.82±1.07	−0.76
D93	−14.06±0.07	−9.88±0.26	−7.71±0.19	−0.39±0.25	−0.83	-	-
N95	−15.46±0.08	−11.23±0.28	−6.87±0.25	0.30±0.39	0.04	-	-
D96	−15.17±0.05	−9.49±0.22	−6.63±0.23	0.28±0.22	0.15	0.12±0.78	0.75
R97	−14.56±0.03	−10.04±0.15	−7.93±0.16	−1.18±0.09	−0.73	-	-
S98	−15.29±0.04	−10.06±0.19	−6.41±0.14	0.19±0.19	−0.03	0.38±0.99	0.92
R99	−15.47±0.04	−10.05±0.29	−5.88±0.19	−1.08±0.18	−0.91	−0.99±1.42	−1.08
I100	−14.79±0.04	−10.22±0.24	−6.14±0.16	1.15±0.16	1.01	−0.28±0.95	−0.73
G101	−14.82±0.05	−10.69±0.26	−6.59±0.19	−0.07±0.18	−0.2	0.21±1.04	0.26
E102	−15.39±0.04	−10.79±0.18	−7.23±0.19	0.44±0.11	0.31	0.38±0.53	0.33
R104	−14.78±0.06	−10.57±0.18	−7.22±0.19	-	-	0.19±0.67	−0.1
E105	−14.80±0.05	−9.53±0.20	−6.18±0.15	−0.15±0.19	0.07	-	-
V106	−15.15±0.04	−10.30±0.20	−6.98±0.16	0.61±0.11	0.49	−1.35±0.55	−0.36
Q108	−14.70±0.05	−10.57±0.26	−7.09±0.29	−0.26±0.17	−0.29	-	-
R109	−14.55±0.06	−10.51±0.26	−7.13±0.26	0.87±0.23	0.33	1.12±0.74	0.64
M110	−15.12±0.08	−10.81±0.52	−7.72±0.62	1.94±0.45	0.92	-	-
L111	−14.38±0.19	-	-	-	-	-	-
N112	−14.59±0.65	−11.14±0.24	−6.76±0.19	−1.80±0.79	0.40#	−0.06±0.50	0.38
E113	−16.15±0.03	−10.91±0.11	−6.55±0.08	0.54±0.08	0.34	−0.22±0.33	−0.56
D114	−14.45±0.04	−9.15±0.16	−9.14±0.18	0.14±0.12	−0.03	−2.64±0.37	−1.44
E115	−14.22±0.06	−10.14±0.33	−6.77±0.31	0.44±0.28	0.54	-	-
L116	−15.54±0.07	−10.93±0.55	−7.87±0.53	−0.66±0.30	−1.02	0.03±1.39	0.71
R117	−15.25±0.08	-	-	-	-	-	-
N118	−15.26±0.11	−10.70±0.25	−6.65±0.17	-	-	1.13±1.69	0.45
A119	−16.19±0.03	−11.62±0.15	−6.59±0.09	−1.35±0.15	−0.64	1.18±0.67	0.02
A120	−15.16±0.29	-	-	-	-	-	-
W121	−16.42±0.41	-	-	-	-	-	-
F124	−13.34±0.17	−9.54±0.74	−8.35±0.90	-	-	-	-
A125	−13.15±0.09	−8.29±0.38	−8.26±0.35	−0.24±0.25	0.51	−2.14±0.84	−1.73
N126	−14.87±0.04	−12.77±0.11	−8.84±0.12	1.00±0.15	0.77	−0.04±0.33	0.51
K127	−15.27±0.04	−9.98±0.26	−8.28±0.32	-	-	-	-
Q128	−14.33±0.09	−10.16±0.36	−7.24±0.36	−0.84±0.36	0.06	-	-
D129	−16.08±0.06	−10.06±0.30	−6.59±0.27	0.21±0.26	0.47	-	-
L130	−16.09±0.03	−11.98±0.16	−6.35±0.09	0.88±0.11	1.09	−0.70±1.40	−1.35
E132	−15.35±0.03	−10.40±0.16	−6.92±0.15	1.19±0.11	1.26	1.20±0.49	0.72
A133	−16.14±0.03	−11.91±0.85	−6.11±0.43	−1.19±0.11	−1.03	−2.35±1.29	−1.61
M134	−15.51±0.01	−11.26±0.08	−9.02±0.09	0.53±0.07	0.64	−0.01±0.26	0.34
S135	−15.14±0.02	−12.79±0.06	−9.40±0.07	0.69±0.12	0.88	-	-
A136	−14.05±0.02	−10.23±0.10	−8.39±0.12	−1.22±0.12	−1.17	−0.87±0.34	−1.23
A137	−15.19±0.03	−9.75±0.17	−6.22±0.14	-	-	-	-
I139	−14.66±0.04	−10.26±0.24	−6.63±0.20	−0.09±0.16	−0.2	0.80±1.06	0.48
E141	−15.11±0.03	−10.43±0.22	−6.31±0.14	−1.27±0.10	−1.18	0.36±0.68	0.28
K142	−14.86±0.03	−10.02±0.21	−6.25±0.16	0.02±0.13	0.04	-	-
L143	−15.19±0.06	−10.40±0.22	−6.39±0.15	−1.11±0.24	−1.34	−1.05±1.03	−0.67
G144	−15.22±0.03	−10.96±0.16	−6.92±0.14	0.13±0.13	0.64	−0.16±0.48	−0.04
L145	-	−9.54±0.16	−6.15±0.11	-	-	-	-
H146	−13.97±0.05	−10.75±0.22	−6.89±0.21	−0.26±0.20	−0.03	−0.43±0.96	0.46
I148	−16.46±0.03	−10.52±0.19	−6.11±0.12	0.59±0.09	0.21	−1.74±0.87	−0.92
R149	−14.33±0.02	−11.00±0.09	−8.19±0.09	−0.18±0.07	−0.02	−0.78±0.22	−1.2
R151	−17.30±0.02	−12.57±0.06	−6.65±0.03	0.12±0.06	−0.31	0.28±0.28	0.55
I155	−15.28±0.02	−11.35±0.09	−8.32±0.10	−0.44±0.08	−0.59	−1.15±0.25	−1.14
A157	−14.26±0.03	−10.50±0.16	−8.54±0.16	0.43±0.59	0.07	−1.39±0.34	−1.32
T158	-	−12.61±0.11	−9.17±0.15	-	-	-	-
A160	−14.74±0.04	−9.44±0.24	−7.38±0.24	-	-	0.17±0.61	−0.02
T161	−14.71±0.10	−10.49±0.31	−6.93±0.25	-	-	−1.01±1.59	0.27
S162	−15.19±0.05	−10.82±0.31	−6.37±0.21	0.46±0.23	0.78	-	-
G163	−15.96±0.12	−10.30±0.18	−5.95±0.12	0.22±0.63	0.48	-	-
E164	−16.88±0.05	−12.17±0.20	−7.12±0.15	−0.78±0.29	−0.86	2.21±0.90	0.99
G165	−15.03±0.02	−9.91±0.11	−9.90±0.13	−0.74±0.07	−0.76	−0.43±0.39	0.16
L166	−14.87±0.03	−10.65±0.14	−7.42±0.15	0.60±0.10	0.47	0.55±0.40	−0.3
Y167	−14.13±0.06	−10.42±0.22	−6.80±0.19	1.27±0.43	0.99	−1.17±1.12	−1.46
E168	−15.11±0.05	−9.88±0.31	−7.37±0.49	−0.43±0.24	−0.75	1.06±0.93	0.74
G169	−15.30±0.06	−11.22±0.25	−7.07±0.25	0.76±0.24	0.35	-	-
L170	−14.87±0.05	−10.06±0.22	−7.47±0.26	0.00±0.15	0.27	−1.31±0.58	−1.38
W172	−15.01±0.04	−9.79±0.24	−6.96±0.24	−1.33±0.32	−0.77	-	-
S174	−14.76±0.05	−10.59±0.26	−7.50±0.37	−0.36±0.53	0.77#	-	-
N175	−15.39±0.03	−10.95±0.19	−6.38±0.12	0.02±0.17	0.36	-	-
S176	−15.67±0.04	−10.76±0.14	−6.93±0.12	0.20±0.20	−0.18	1.48±0.76	0.64
L177	−15.67±0.01	−10.63±0.09	−6.07±0.05	0.10±0.04	0.43	−0.12±0.26	0
K178	−15.05±0.01	−10.50±0.05	−6.81±0.04	1.10±0.02	1.27	−0.61±0.10	−0.95
N179	−15.02±0.01	−10.93±0.03	−7.36±0.03	−0.46±0.01	*	−0.01±0.06	*
S180	−15.70±0.01	−11.39±0.02	−7.81±0.02	0.32±0.01	*	0.42±0.04	*

^*back-calculated RDC values unavailable for the N-terminal residues due to their absence in the crystal structure, and the very C-terminal residues which are flexible and therefore excluded from fitting.

^-Data unavailable due to poor multiple-point fitting by Eq(2). For the isotropic measurement, fitting quality is deemed “poor” if the Monte Carlo simulation error is greater than 1Hz. RDCs with errors over 2Hz are deemed “poor” and excluded.

^#RDC data excluded from Figure 3 due to significant deviation from back calculated values.

It is worth noting that the ratio in the ¹J_NC’ experiment oscillates roughly between 1 and −1, depending on the level of ¹³C incorporation and inversion efficiency, while the ratio in the ¹J_NCα-²J_NCα experiment oscillates within a much narrower range over the same modulation delays (Figure 2). The smaller dynamic range for the latter experiment makes it more prone to experimental errors. Obviously the equation for the latter experiment also has a significantly longer period than a single cosine function. This is easily seen by rewriting the cos(πJ₁t)·cos(πJ₂t) function as 1/2·(cos(π(J₁+J₂)t))+cos(π (J₁−J₂)t)). Assuming J₁ = 11 Hz and J₂ = 7 Hz, the slow and fast components have periods of 0.5s and 0.11s respectively. It is impractical to take modulation delays up to the range of the slow modulation without substantial losses in sensitivity. The inability to sample all features of the curve also tends to lower the accuracy. As a result, the measurement accuracy of ¹J_NC’ is superior to those of ¹J_NCα and ²J_NCα. Errors derived from least-squares fits of 500 Monte Carlo simulations are give in Figure 2 for the residues used as examples. Cases where the simulated errors are greater than 1 Hz for the isotropic measurement are deemed too noisy to produce acceptable fits and are excluded from Table 1. RDC’s with errors greater than 2Hz are also excluded. These filtering steps resulted in a larger number of reliable ¹D_NC’ couplings than ¹D_NCα couplings (Table 1). The average measurement errors of ¹D_NC’ and ¹D_NCα couplings are 0.19 and 0.67 Hz for the accepted residues. The RMS between the experimental ¹D_NC’ and ¹D_NCα couplings and their back-calculated values are 0.36 and 0.55Hz respectively. A small number of accepted residues that deviate from the back-calculated values significantly more than RMS were excluded from Figure 3. These include 2 out of 93 for ¹D_NC’ (N112 and S174), and 1 out of 71 for ¹D_NCα (E41) (Table 1). One concern with the measurement of ¹J_NCα/¹D_NCα/ and ²J_NCα is that for Gly, Ser, and Thr, the ¹³C inversion efficiency could be degraded due to displacement of their chemical shifts from average values [10]. Here we used a reburp pulse centered on 56ppm with a bandwidth of 50ppm, which covers the entire C^α region (Figure 1, legends). We also explicitly take the inversion efficiency into account during data fitting (Eq. (1)). Based on the experimental and back-calculated ¹D_NCα values, the effects of C^α chemical shift dispersion do not seem particularly severe (data not shown).

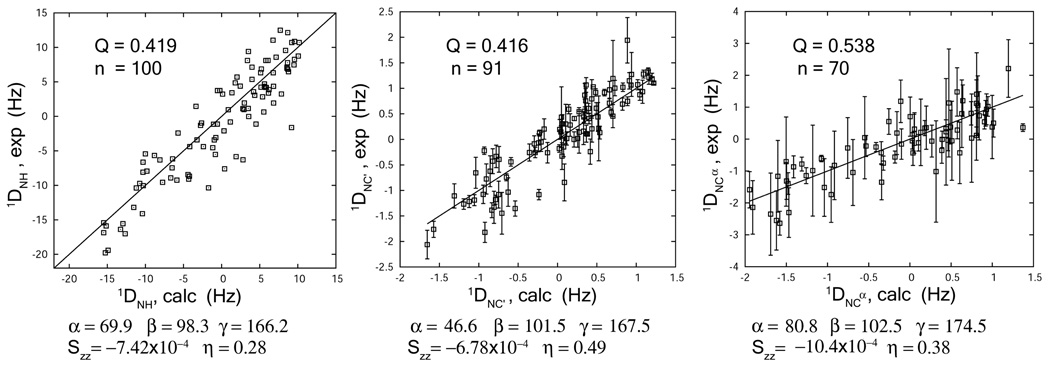
Figure 3.

Best fits of the ¹D_NC’ and ¹D_NCα couplings by single value decomposition to the crystal structure of non-myristoylated mARF1-GTP-Δ17. Fit of the backbone amide ¹D_NH couplings measured from an interleaved pair of TROSY-HSQC experiments and an HNCO-based 3D J-NH experiment is provided as a reference. The alignment tensor parameters are indicated, including the Euler angles (by z-y-z convention) α, β and γ for transforming the given molecular frame into the principle axis frame, the alignment principle order parameter S_zz and the asymmetry parameter η (η = (S_xx−S_yy)/S_zz ).

5. Discussion

Since a high-resolution structure of the bicelle-bound and myristoylated yeast ARF1·GTP is not available, the ¹D_NC’ and ¹D_NCα couplings are compared with the back-calculated values based on the crystal structure of the non-myristoylated, GTP-bound mouse ARF1 protein (PDB: 1o3y). These comparisons are presented in Figure 3. In addition to coming from a different organism, the construct used for this crystal structure is missing the N-terminal 17 residues (mARF1-GTP-Δ17) as well as the N-terminal myristoyl chain. However, mouse ARF1 shares 76% sequence identity with the yeast ARF1 protein. RDCs from the N-terminal 17 residues are excluded from Figure 3 due to their absence in the crystal structure. The relatively low Q factors indicate there is significant structural similarity between the two proteins. The deviation from a perfectly linear match is a combined effect of RDC measurement errors, structural differences between yeast and mouse proteins, structural perturbation upon myristoylation, N-terminal deletion, bicelle interaction, and any internal dynamics. Given these potential contributions, Q factors in the 0.4–0.5 range are quite acceptable.

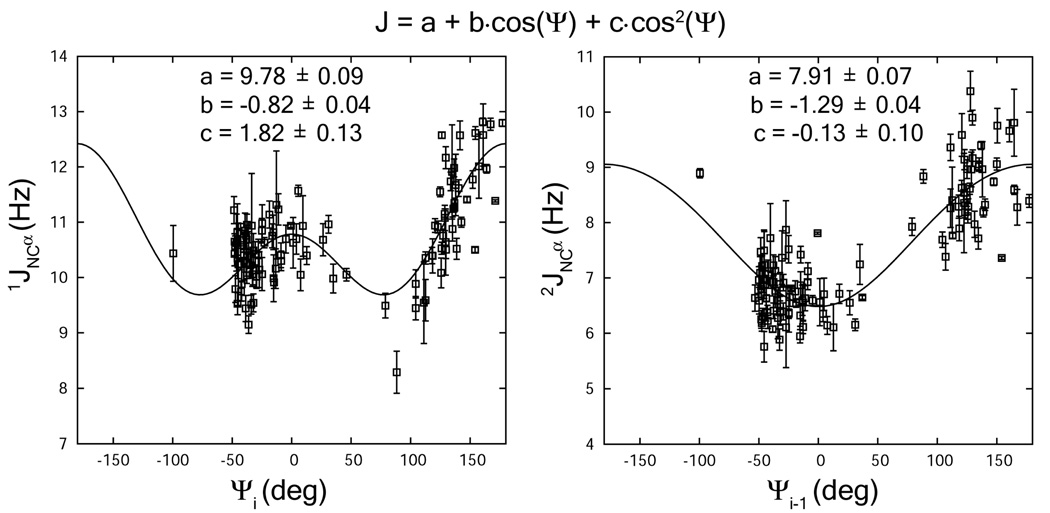
The 1-bond ¹J_NCα and 2-bond ²J_NCα scalar couplings are expected to follow a Karplus equation as a function of the backbone Ψ_i and Ψ_i−1 angles. We have used a functional form identical to that preciously presented [10] (Eq 4) and optimized parameters using angles derived from the mARF1-GTP-Δ 17 crystal structure. The fit to the optimized equations are shown in Figure 4. The coefficients as shown in Eq 4 are similar to previously reported values, 9.78±0.09, −0.82±0.04, and 1.82±0.13 for 1-bond ¹J_NCα, and 7.91±0.07, −1.29±0.04, and −0.13±0.10 for 2-bond ²J_NCα [10].

Figure 4.

Fitting of the ¹J_NCα and ²J_NCα couplings to the Karplus relationship as a function of backbone torsion angles Ψ_i and Ψ_i−1 respectively. The torsion angles were calculated from the crystal structure of non-myristoylated mARF1-GTP-Δ17.

$$\begin{array}{c}{}^1{J}_{{\mathit{\text{NC}}}^{\alpha }(i)}=(9.78\pm 0.09)+(-0.82\pm 0.04)\cdot \text{cos}({\mathrm{\Psi }}_i)+(1.82\pm 0.13)·{\text{cos}}^2({\mathrm{\Psi }}_i)\hfill \\ {}^2{J}_{{\mathit{\text{NC}}}^{\alpha }(i-1)}=(7.91\pm 0.07)+(-1.29\pm 0.04)·\text{cos}({\mathrm{\Psi }}_{i-1})+(-0.013\pm 0.10)·{\text{cos}}^2({\mathrm{\Psi }}_{i-1})\hfill \end{array}$$ (4)

The agreement and indicated precision of parameters suggests the data will be useful in optimizing backbone Ψ_i and Ψ_i−1 torsional angles.

Appendix A

For the ¹J_NC’ experiment (Fig 1A), the detectable signal at point b resides on the transverse ¹⁵N magnetization that is in phase with respect to ¹³C’:

$$\begin{array}{c}{I}^{J\text{mod}}=((1-p)+p·\frac{1+e}{2}·\text{cos}(\pi ·{}^1{J}_{\mathit{\text{NC}}\text{'}}·t_{\mathit{\text{CN}}})+p·\frac{1-e}{2}·\text{cos}(\pi ·{}^1{J}_{\mathit{\text{NC}}\text{'}}·t_1))·k\hfill \\ I^{\mathit{\text{ref}}}=((1-p)+p·\frac{1+e}{2}+p·\frac{1-e}{2}·\text{cos}(\pi ·{}^1{J}_{\mathit{\text{NC}}\text{'}}·t_1))·k\hfill \end{array}$$ (A1)

Here p is the percentage of ¹³C incorporation (p = 1 for 100% ¹³C), e is the inversion efficiency of ¹³C selective π pulses (e = 1 for 100% inversion), and k is the signal damping due to relaxation. The small relaxation difference between in-phase N_x/y and anti-phase 2N_x/yC’_Z is neglected because the longitudinal relaxation of ¹³C’ is much slower than the transverse relaxation of ¹⁵N, and therefore a single damping factor k is applied. Both I^Jmod and I^ref contain 3 terms. The first term (1−p) comes from the ¹²C’ labeled population whose signal is not modulated by ¹J_NC’ in either case. The other two terms are associated only with the ¹³C labeled population. The second term is the only difference between I^Jmod and I^ref, which represents the signal that is modulated in the filtered experiment but not in the reference. The third term containing cos(π·¹J_NC’·t₁) results from incomplete ¹J_NC’ refocusing during ¹⁵N chemical shift evolution from a non-ideal ¹³C π pulse, which causes p·(1−e)/2 of the total signal to be broadened by ¹J_NC’ after t₁ Fourier transform. When centered on chemical shift, this broadened peak has a normalized height h:

$$h=\frac{{\displaystyle {\int }_0^{\mathrm{\infty }}\text{exp}(-R·t)·\text{cos}(\pi ·J·t)·\mathit{\text{dt}}}}{{\displaystyle {\int }_0^{\mathrm{\infty }}\text{exp}(-R·t)·\mathit{\text{dt}}}}=\frac{R^2}{R^2+{(\pi ·J)}^2}$$ (A2)

Here R is the transverse relaxation rate during t₁ evolution plus any exponential apodization function added during post-processing.

Through substitution by r, given as follows:

$$r=\frac{p·\frac{1+e}{2}}{1-p+p·\frac{1+e}{2}+p·\frac{1-e}{2}·h}$$ (A3)

the expression for the ¹J_NC’ experiment in Eq.(1) can be derived. It must be mentioned that because h depends on the F₁ line-width (Eq. (A2)), the ratio r is different between semi-constant time and constant time experiments. However during our data fitting, the variation of r due to linewidth is neglected so that a single r is used with different t_CN values, assuming that (π·J)² ≪ R² (h ≈ 1) and that ¹³C inversion is nearly complete (e ≈ 1). The first assumption is also reasonable because the N-C coupling is quite small and an apodization function is generally used to ramp the data down to zero at the end of a relatively short acquisition time, thereby imposing a large relaxation rate.

For the ¹J_NCα-²J_NCα experiment, the ¹³C labeling and inversion efficiency for both C^α _(ι)and C^α _(ι−1) has to be considered. Because the weak J-coupling Hamiltonians commute, a simple recursive relationship is observed with every addition of a J-coupled carbon:

$$\begin{array}{c}{I}_n^{J\text{mod}}=I_{n-1}^{J\text{mod}}·((1-p_n)+p_n·\frac{1+e_n}{2}·\text{cos}(\pi ·J_n·t_{\mathit{\text{CN}}})+p_n·\frac{1-e_n}{2}·\text{cos}(\pi ·J_n·t_1))\hfill \\ I_n^{\mathit{\text{ref}}}=I_{n-1}^{\mathit{\text{ref}}}·((1-p_n)+p_n·\frac{1+e_n}{2}+p_n·\frac{1-e_n}{2}·\text{cos}(\pi ·J_n·t_1))\hfill \end{array}$$ (A4)

Here I_n represents the intensity from a system of n J-coupled carbons. The ¹³C percentage and the inversion pulse efficiency of the n-th carbon are p_n and e_n. It is simple to solve the recursive relation above to get:

$$\begin{array}{c}{I}_n^{J\text{mod}}=k·{\displaystyle \prod _{i=1}^n((1-p_i)+p_i·\frac{1+e_i}{2}·\text{cos}(\pi ·J_i·t_{\mathit{\text{CN}}})+p_i·\frac{1-e_i}{2}·\text{cos}(\pi ·J_i·t_1))}\hfill \\ I_n^{\mathit{\text{ref}}}=k·{\displaystyle \prod _{i=1}^n((1-p_i)+p_i·\frac{1+e_i}{2}+p_i·\frac{1-e_i}{2}·\text{cos}(\pi ·J_i·t_1))}\hfill \end{array}$$ (A5)

For n=2, as illustrated by the ¹J_NCα-²J_NCα experiment, the intensity ratio between the filtered and reference experiments adapts the following general form with 5 floating parameters, a, b, c, ¹J_NCα and ²J_NCα:

$$\begin{array}{cc}\frac{I^{J\text{mod}}}{I^{\mathit{\text{ref}}}}\hfill & =(1-a-b-c)+a·\text{cos}(\pi ·{}^1{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}})·\text{cos}(\pi ·{}^2{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}})\hfill \\ \hfill & +b·\text{cos}(\pi ·{}^1{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}})+c·\text{cos}(\pi ·{}^2{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}})\hfill \end{array}$$ (A6)

Here the coefficients must satisfy a, b, c ≥ 0 and a + b + c ≤ 1. Again, the small effect of line-width variations on the coefficients a, b, and c are neglected. Data fitting by direct application of Eq.(A6) cannot ensure that a, b, and c fall in the legal range. In our actual test, a, b, and c are represented by cosine square functions:

$$\begin{array}{cc}\frac{I^{\mathit{\text{fill}}}}{I^{\mathit{\text{ref}}}}\hfill & =(1-{\text{cos}}^2(a)-{\text{cos}}^2(b)-{\text{cos}}^2(c))+{\text{cos}}^2(a)·\text{cos}(\pi ·{}^1{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}})·\text{cos}(\pi ·{}^2{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}})\hfill \\ \hfill & +{\text{cos}}^2(b)·\text{cos}(\pi ·{}^1{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}})·{\text{cos}}^2(c)·\text{cos}(\pi ·{}^1{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}})\hfill \end{array}$$ (A7)

This works well for most couplings, but the ¹D_NCα couplings obtained using Eq. (A7), however, agree poorly with the back-calculated values based on the crystal structure of mARF1-GTP-Δ17 (Q factor = 1.24, data not shown). In contrast, the simplified relations, with only 3 floating parameters, shown in the bottom lines of Eq.(1)/(2) significantly improves the agreement (Figure 3). This suggests that in Eq. (A6), the extra degrees of freedom led to over-interpretation of the input data with limited S/N, and trapped the fitting procedure in an erroneous minimum.

In the derivation of the bottom line in Eq. (1), the following restraints are imposed to avoid over-fitting:

$$\begin{array}{c}{\displaystyle {\int }_0^{\mathrm{\infty }}\text{exp}(-R·t)·\text{cos}(\pi ·{}^1{J}_{{\mathit{\text{NC}}}^{\alpha }}·t)·\mathit{\text{dt}}·{\displaystyle {\int }_0^{\mathrm{\infty }}\text{exp}(-R·t)·\text{cos}(\pi ·{}^2{J}_{{\mathit{\text{NC}}}^{\alpha }}·t)·\mathit{\text{dt}}}}\hfill \\ \approx {\displaystyle {\int }_0^{\mathrm{\infty }}\text{exp}(-R·t)·\text{cos}(\pi ·{}^1{J}_{{\mathit{\text{NC}}}^{\alpha }}·t)·\text{cos}(\pi ·{}^2{J}_{{\mathit{\text{NC}}}^{\alpha }}·t)·\mathit{\text{dt}}·{\displaystyle {\int }_0^{\mathrm{\infty }}\text{exp}(-R·t)·\mathit{\text{dt}}}}\hfill \end{array}$$ (A8)

This approximation is quite good when (π·J)² ≪ R². Through this approximation, the following equation is derived:

$$\begin{array}{cc}\frac{I^{J\text{mod}}}{I^{\mathit{\text{ref}}}}\hfill & =(1-r_1)·(1-r_2)+r_1·r_2·\text{cos}(\pi ·{}^1{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}})·\text{cos}(\pi ·{}^2{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}})\hfill \\ \hfill & +r_1·(1-r_2)·\text{cos}(\pi ·{}^1{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}})+r_2·(1-r_1)·\text{cos}(\pi ·{}^2{J}_{{\mathit{\text{NC}}}^{\alpha }}·t_{\mathit{\text{CN}}})\hfill \end{array}$$ (A9)

where,

$$r_i=\frac{p_i·\frac{1+e_i}{2}}{1-p_i+p_i·\frac{1+e_i}{2}+p_i·\frac{1-e_i}{2}·h_i}$$ (A10)

Eq. (A9) is further simplified to Eq. (1) (bottom) by requiring r₁ ≈ r₂ and thus replacing them with a single parameter r. The ¹³C incorporation level and inversion efficiency usually vary within a small range (i.e., p₁ ≈ p₂ and e₁ ≈ e₂), and the small difference in h_i associated with the nearly equal ¹J_NCα and ²J_NCα is further scaled down by a small number p·(1-e)/2 (Eq. (A10)). Thus r₁ ≈ r₂ is a good approximation.