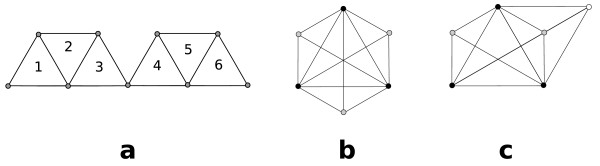
Figure 2.
(a) The K3s marked by 1 and 2 share two nodes, as do the K3s marked by 2 and 3, 4 and 5, and 5 and 6. Each of these pairs is thus 3-adjacent by definition 1. Since 1 and 2 and 2 and 3 are 3-adjacent, 1 and 3 are 3-clique-connected by definition 2. But since 3 and 4 share only one vertex, they are not 3-adjacent. (b) Each of the grey nodes constitutes a K4 together with the three black nodes. Thus, all three K4s are 4-adjacent. (c) An example of three K4s that are 4-clique-connected.