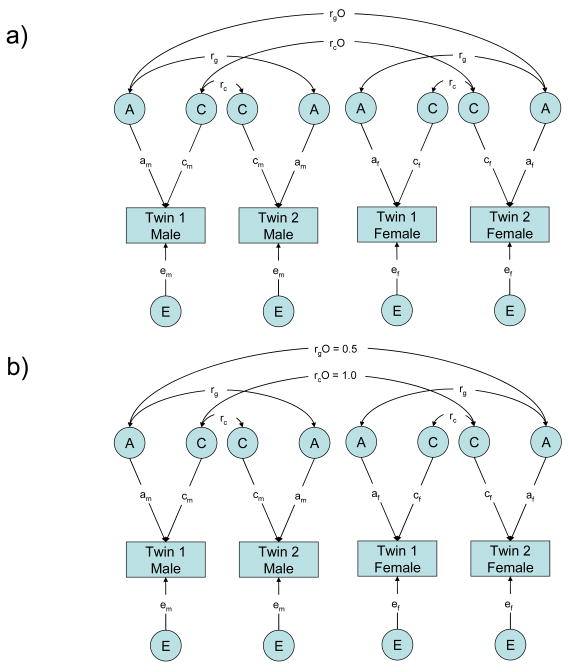
Figure 2.

Full Sex-Limitation Model and Nested Models

Full sex-limitation model and nested models. Note: A = additive genetic influence; C = shared environment influence; E = non-shared environment influence; path coefficients a, c, and e = effects of A, C, and E on a trait, with subscript “m” for males and “f” for females; rg = genetic correlation between same-sex twins, which is fixed at 1.0 for MZ twins and 0.5 for DZ twins; rc = shared environment correlation between same-sex twins, which is fixed at 1.0 for MZ and DZ twins; rgO = genetic correlation between opposite-sex twins; rcO = shared environment correlation between opposite-sex twins. Opposite-sex twins are represented as twin 1 male and twin 2 female, and are linked by rgO and rcO. (a) Full sex limitation model. This model estimates seven parameters: am, cm, em, af, cf, ef, and rgO or rcO. This model allows qualitative sex differences by allowing the genetic and shared environmental correlations (rgO and rcO) between opposite-sex twins to vary. The model also allows quantitative sex differences by allowing the ACE parameters for males and females (am, cm, em, af, cf, ef) to differ. Variances differences between the sexes are allowed (this cannot be seen in the path diagram). (b) Common effects model, which is nested in the full sex-limitation model. The common effects model constrains the genetic and shared environmental correlations between opposite-sex twins (rgO and rcO) to be 0.5 and 1.0, respectively (thereby treating the opposite-sex twins in the same way as the same-sex DZ twins who have a genetic correlation of 0.5 and a shared environmental correlation of 1.0). This model estimates six parameters (am, cm, em, af, cf, ef) and allows variance differences between the sexes. Please see Figure 1 for the path diagram of the scalar and null models.