Abstract
We studied adjustments of digit forces to changes in the friction. The subjects held a handle statically in a three-digit grasp. The friction under each digit was either high or low, resulting in eight three-element friction sets (such grasps were coined the grasps with complex friction pattern). The total load was also manipulated. It was found that digit forces were adjusted not only to the supported load and local friction, but also to friction at other digits (synergic effects). When friction under a digit was low, its tangential force decreased and the normal force increased (local effects). The synergic effects were directed to maintain the equilibrium of the handle. The relation between the individual digit forces and loads agreed with the triple-product model: , where is normal force of digit i, L is the load (newtons), is a dimensionless coefficient representing sharing the total tangential force among the digits , and is a coefficient representing the relation between the tangential and normal forces of digit i (the overall friction equivalent, OFE). At each friction set, the central controller selected the grasping template—a three-element array of products—and then scaled the template with the load magnitude.
Introduction
When people manipulate handheld objects the digit forces are adjusted to friction. Three friction conditions were examined in the literature: 1) symmetric friction: same friction at all digit contacts; 2) asymmetric friction: high friction at one side of the grasp, for instance beneath the thumb, and low friction at the other side, i.e., beneath the fingers; and 3) complex friction pattern: the friction at each digit is different.
In symmetric friction conditions more slippery and heavier objects are grasped with more force (Johansson and Westling 1984; Westling and Johansson 1984). It has been reported that “the static grip force was approximately proportional to the weight of the object” (Westling and Johansson 1984) and “the slope of this relation was different … being higher the more slippery the surface structure” (Westling and Johansson 1984). Adjustment of the grip force to the loads and symmetric friction conditions has been confirmed in many studies (Aoki et al. 2006; Birznieks et al. 1998; Burstedt et al. 1997, 1999; Cadoret and Smith 1996; Cole and Johansson 1993; Edin et al. 1992; Johansson 1996; Johansson and Westling 1987; Kinoshita et al. 1995; Quaney and Cole 2004). Tactile afferents from individual digits play a role in this force adjustment (Edin et al. 1992; Johansson and Westling 1984, 1987).
Under the asymmetric friction conditions the tangential digit forces were smaller at the more slippery contacts and the object slightly tilted toward the more slippery side (Burstedt et al. 1997; Edin et al. 1992; Quaney and Cole 2004). Similar force patterns have been observed when the object was grasped by two digits of one hand, single digits of two hands, or by two subjects who touched the object with one index finger each (Burstedt et al. 1997). It was concluded that digit coordination during human manipulation emerged from independent neural networks controlling each engaged digit. The authors also recognized a higher level of control related to actions before object grasping (selecting grasp configurations and temporal synchronization of digit movements) as well as coping with the weight and size of the object. In five-digit grasps, under asymmetric surface conditions and the instruction to avoid object tilting, the performers exerted smaller tangential forces at the more slippery interface and compensated the arising moment of the tangential forces by the oppositely directed moment of the normal forces (Aoki et al. 2006).
The complex friction conditions in which the friction beneath individual digits was varied one by one were addressed, to the best of our knowledge, in only two studies. Aoki et al. (2007) studied the five-digit grasps in which individual digits were in contact with either sandpaper (high friction) or rayon (low friction). On the whole there were 32 digit–friction combinations, such as HLHLH, LHHHH, and the like, where the letters correspond to either high-friction (H) or low-friction (L) contacts at the thumb, index, middle, ring, and little finger, respectively. The force adjustments to the friction were classified as local and synergic. In the present context, the term local designates an effect of friction at a given digit on the force exerted by this digit. The term synergic refers to changes of digit force in response to changes in friction for other digits (Aoki et al. 2006). In some subjects, the local reactions were always present—i.e., at the more slippery contacts the normal forces were always larger and the tangential forces were smaller than those at the high-friction contacts—and the object equilibrium was maintained by the synergic compensating adjustments. In other subjects, the local reactions were not exhibited, ostensibly because of the inhibition of the local reactions by higher-order (synergic) neural mechanisms.
Three-digit grasps were studied by Burstedt et al. (1999). The subjects grasped a cylindrical object from above, lifted, and held it. The digits either all contacted the same surface material (rayon or sandpaper; the symmetric friction condition) or one of the digits contacted a surface material that was different from the others (the complex friction condition). In the latter case, the subjects adapted the digit forces to the local frictional conditions with only small influences from the friction at the other two digits (i.e., the local effects were significant while the synergic effects were not). The authors concluded that “no clear strategy could be identified that could account for the robust influences by the frictional condition on the load distribution among digits” (Burstedt et al. 1999). It is not clear whether such a conclusion is specific to a grasp studied or whether it is valid for all grasps. Three-digit grasps of cylindrical objects from above possess one special mechanical property: to achieve the object equilibrium the three normal forces should intercept at one point (see, e.g., Zatsiorsky 2002). However, other grasps such as the prismatic grasp (i.e., grasping a glass with a liquid) do not have this property. In general the results of the two studies are inconclusive.
The present study is intended to explore how the CNS adjusts digit forces to the complex friction conditions in three-digit prismatic grasps similar to grasping a glass with liquid. We explain the research question with an example. Suppose a performer holds a glass filled with liquid using the thumb, index, and middle fingers. The friction at the thumb and the index finger is high (H), whereas the friction at the middle finger is low (L). The entire set can be marked as HHL, where the consecutive letters refer to the thumb, index, and middle fingers, respectively. Whatever the friction set is the following equilibrium conditions have to be satisfied: 1) the normal forces should be large enough to prevent slipping of the object; 2) the normal forces of the thumb and the two fingers combined must be equal; 3) the sum of the tangential forces should equal the object weight, and 4) the resultant moment of force exerted on the object should be zero. According to the literature data (see above) we should expect the local friction effect on the middle finger—i.e., the finger would exert a larger normal force than it exerts in the HHH task (when the friction at all fingers is high). However, such an increase of the forces of the middle finger will break the object equilibrium. First, the resultant normal force of the two fingers will be larger than that of the thumb force (and thus the object will accelerate in the thumb direction). Second, the normal forces of the index and middle fingers will differ in magnitude and thus will exert a moment of force with respect to the thumb, which acts as a pivot. If not compensated, such a moment will result in tilting the object and spilling the liquid. The central controller in such a situation has two main options: 1) allow the local friction adjustment to emerge and then compensate the perturbation by synergic actions (e.g., by exerting a counterbalancing moment of tangential forces) or 2) inhibit the local reactions.
If at a given friction set the supported load varies among the trials, the central controller also has two options: 1) to change the force-sharing pattern with the load (for instance, decrease the sharing percentage of the force exerted by the weakest finger when the load increases) or 2) maintain the sharing percentage constant (i.e., select a force distribution template and then scale it with the load).
The ultimate goals of the present study have been to determine the strategies used by the CNS to deal with the changes of the friction at the individual digit–object interfaces and load. Two hypotheses were explored. According to the hypotheses the CNS:
1) Does not inhibit local adjustments to friction; instead it compensates the perturbation effect of the local reactions on the object equilibrium by synergic actions of other digits.
2) Uses a single force distribution pattern (grasping template) for each friction set and scales the template with the load.
Both hypotheses have implications beyond the immediate goals mentioned earlier. In particular, confirming the above-cited hypotheses would corroborate two hypotheses suggested recently: 1) a hypothesis that immediate reactions to a perturbation may contribute to the induced disturbance of the equilibrium rather than restore it (Hasan 2005); and 2) a hypothesis that control of force magnitude is achieved by proportional scaling of elementary forces exerted by the contributing elements, whether these are individual fingers or muscles (Valero-Cuevas 2000).
Methods
Subjects
Eight male subjects participated in the experiment (age 28.6 ± 4.0 yr, weight 71.6 ± 16.1 kg, height 173.6 ± 7.4 cm, hand length from the middle fingertip to the distal crease of the wrist with hand extended 18.7 ± 1.1 cm, hand width at the MCP level with hand extended 9.2 ± 0.5 cm). Because the hand size of females is usually smaller than that of males, only male subjects were recruited to avoid confounding factors related to the hand anatomy. The subjects were all right-hand dominant and had no history of neuropathies or trauma of their upper limbs. All subjects gave informed consent according to the policies of the Office for Research Protections of The Pennsylvania State University.
Apparatus
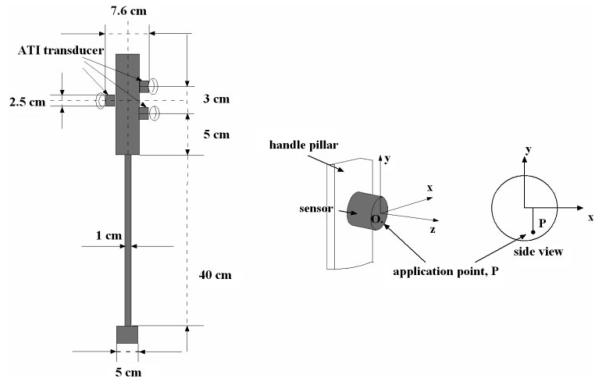
Three six-component force/moment transducers (Nano-17, ATI Industrial Automation, Garner, NC) were mounted on an aluminum handle at the bottom of which a vertical aluminum bar was attached (diameter 1 cm, length 40 cm; Fig. 1). The center points of the index and middle finger sensors were positioned respectively 1.5 cm above and below the center of the thumb sensor, which was at the midpoint of the handle. The mass of the handle with the aluminum bar was 500 g. Four loads (200, 400, 600, or 800 g) were fixed at the bottom of the rod. With the suspended loads, object weights were 6.71, 8.67, 10.63, and 12.59 N, respectively. The moments of inertia of the system with respect to a center at the thumb level were 0.054, 0.111, 0.171, and 0.233 kg·m2, respectively. At all suspended loads, location of the center of gravity of the system was much below the grasp. This was done to make the rotational equilibrium stable: any angular deviation of the bar from the vertical generated a restoring gravity moment. When holding such an object, the subjects did not have to be concerned about maintaining the vertical orientation of the handle and preventing the tilt; if inclined, the handle would restore the vertical orientation by itself. Voluntary rotation of the handle required substantial efforts; for example, with the load of 800 g a static inclination from the vertical of 3.5° would require a torque of 0.215 Nm. Thus for the performers the tilt prevention requirements were highly relaxed. Although such a low location of the center of mass is not typical for many objects manipulated in everyday life (although still possible; think about a golf club held in one arm as an example), it has one experimental advantage. In objects with the center of mass close to the hand dual effects of friction manipulation can be expected: 1) the digit force sharing is changed and 2) the object is more or less tilted. This makes data analysis and interpretation more difficult. Limiting the experimental effects to the digit forces would, as we expected, allow us to find out an unimpeded adjustment to the complex friction patterns preferred by the CNS. A level was positioned at the top of the handle to visually help the subjects to keep the handle vertically oriented.
Fig. 1.
Schematic drawing of the apparatus with 3 digits on the sensors. Caps with different friction surfaces that were attached above the sensors are not shown.
Different friction conditions of digit–object interface were achieved by exchanging the contact surfaces. Caps with either rayon or sandpaper surfaces were affixed to the interfacing portion of the sensors. The diameter of the cap contact surface was 2.5 cm, which was large enough for subjects to comfortably adjust their grasping pattern. The height of the caps—i.e., the distance from the cap surface to the sensor surface—was 5 mm. The grip width with the caps attached was 7.6 cm. Grip surfaces used for high and low frictions were 100-grit sandpaper and rayon, respectively. The friction coefficients (μ) between the skin and these materials were determined previously (Aoki et al. 2006): 1.34 ± 0.05 for the sandpaper and 0.49 ± 0.05 for the rayon.
The force/moment signals from the sensors were digitized by two 32-channel 12-bit A/D converters (PCD-6033E, National Instruments, Austin, TX). The sampling frequency was 1,000 Hz. The digital signals were processed by a PC computer (Dell Dimension 8200, Round Rock, TX) with a customized program written in LabVIEW 6.1 (National Instruments).
Experimental conditions
The experimental design included 32 combinations of eight friction conditions and four loads. Both the friction conditions and the loads were balanced. The eight frictions were introduced to eight subjects in a balanced order and then four loads were introduced in a balanced order with the friction fixed. At each friction condition each subject performed 16 trials (4 loads × 4 trials). The eight friction conditions were HHH, HLL, HHL, HLH, LLL LHH, LHL, and LLH, where the letters correspond to the friction condition for the thumb, index, and middle fingers, respectively. H (high friction) represents sandpaper and L (low friction) represents rayon. As an example, the abbreviation LHL designates the task where the thumb and middle finger contacted rayon while the index finger was in contact with sandpaper. Each subject performed 128 trials (8 friction conditions × 16 balanced load conditions).
Experimental procedure
Before the experiment, subjects were given an orientation session to familiarize them with the experimental tasks and apparatus. Then, subjects washed their hands with soap and warm water to normalize skin condition and waited for 5 min to stabilize the friction coefficient between the contact surface and the digits.
Subject sat in a chair alongside a table, with the right upper arm positioned at approximately 45° abduction in the frontal plane and 45° flexion in the sagittal plane. The elbow joint was flexed about 45°. The forearm, but not the wrist and hand, was placed on the brace. The brace was fastened to the table and the forearm was fixed on the brace with an elastic band. The forearm was pronated 90° such that the hand was in a natural grasping position.
Subjects were instructed to take the handle from the rack with their left hand, and place it in the right hand. Then, the subjects were instructed to hold the handle vertically in the air with natural grip force. Special attention was paid to digit placement on the sensors ensuring that the center of each digit tip coincided with the center of the corresponding sensor. If slipping occurred, the trial was terminated and repeated again after 10 s. The number of slips was <10 in all subjects (out of 128 trials). After the data collection in a trial stopped, the subjects placed the handle back on the rack and took a break. The investigator would change the load or friction caps and informed the subject that he can start the next trial.
Each trial took 6 s, after which the subject took a 12-s break. Breaks of ≥3 min were provided between two friction conditions. The total duration of each experiment was about 1.5 h. The room was air-conditioned. During all experimental sessions, the ambient temperature and humidity were almost constant, 23°C and 34%, respectively. No visible signs of sweating were observed. Fatigue was never a problem.
An additional two-part control test was performed. The goal of the test was to check whether systematic changes in the grasping forces, such as those arising from fatigue or sweating, occurred during the experiment. Before and after the main experiment, the subjects grasped and held the handle with the thumb, index, and middle fingers for 16 trials, each of which lasted 3 s. The friction condition was the HHH with the 400-g load. The data obtained in the first and the second sets of measurements were compared.
Data analysis
Signals were set to zero before each trial. Data-acquisition software written in LabVIEW was used to convert digital signals into the force and moment values. Data processing was performed using Matlab (The MathWorks, Natick, MA). The raw force/moment data were filtered with a fourth-order Butterworth low-pass filter at 5 Hz.
The net mechanical effect of the index and middle fingers was treated as arising from the action of an imagined virtual finger (VF) (Arbib et al. 1985; Baud-Bovy and Soechting 2001; Iberall 1987; Santello and Soechting 2000). The main variables were computed as follows.
1) The normal forces of the digits and VF, and .
2) The tangential forces of the digits and VF, and . Upward tangential forces were defined as positive.
Triple-product model of digit forces
The preliminary results have shown that the relations between: 1) tangential forces and load, 2) normal forces and tangential forces, and 3) the normal forces versus load were linear for all digits and friction sets. In the triple-product model the linearity of the preceding relations has been recognized.
The proportionality of the tangential forces and the load should be expected from an evident consideration: at the zero loads the tangential forces are also zero (provided that at the zero loads none of the tangential force is negative, i.e., directed downward, which never happened in experiments). Thus the tangential forces equal
| (1) |
where is a tangential force of digit i, L is the load (newtons), and is a dimensionless coefficient (<1.0). Coefficients specify the sharing percentage of the total tangential force (load) among the digits. Thus if measured without errors, .
Coefficients correspond to the dimensionless normal force: tangential force ratios; they specify the amount of normal force per unit of tangential force. If at the zero tangential force the normal force is also zero the relation between the normal and tangential force is
| (2) |
Such a relation corresponds to the instruction (or the internal rule followed by the subject) “exert minimal normal force necessary to prevent slipping.” If this rule is not strictly followed the regression intercept (ai) may differ from zero and the equation would be . Because of the linearity of Eq. 2 the coefficient can be viewed as a scaling factor.
| (3a) |
which represents the triple-product model of the digit force control. Equation 3a can also be written as
| (3b) |
where is a dimensionless coefficient representing a relation between a normal force of digit i and the load. Note that can be either computed from the product or determined experimentally.
A full set of coefficients and will be called a grasping template. The grasping template can be written as a 2 × 3 matrix, where the rows correspond to k(1) and k(2) coefficients and the columns to the individual digits. The template can be understood as a set of digit forces at L = 1. When multiplied by the load magnitude—following Eqs. 1 and 3a—the template equals the tangential and normal digit forces, respectively.
Statistical analysis
The , , and coefficients were computed from the relevant regression analyses. A two-way repeated-measures ANOVA was performed on the coefficients with the factors LOCAL FRICTION (LF, two levels) and FRICTION AT OTHER DIGITS (FOD, four levels). For an individual digit, the local friction condition was defined as the friction beneath the finger. In the present experiment it can be either high (H) or low (L). The synergy effect is the effect of friction at digits A and B on the force exerted by digit C. There were four friction conditions for A and B: HH, HL, LH, and LL. For the VF, the synergy effects were understood as the effects of the thumb friction on the VF forces.
The repeated-measures ANOVA was preceded by testing its assumption of sphericity, for which Mauchly's test of sphericity was used. If sphericity was violated, the degrees of freedom were adjusted as necessary with the Greenhouse–Geisser method. The significance level was set as 0.05. Paired Hotelling's T-squared tests were performed for the 16 trial sets from the two-part control tests performed before and after the main experiment for each subject. Statistical analyses were performed in the statistics toolbox of Matlab 7.0 (The MathWorks) and SPSS 13.0 (SPSS, Chicago, IL).
Checking for accuracy and possible trend in the data
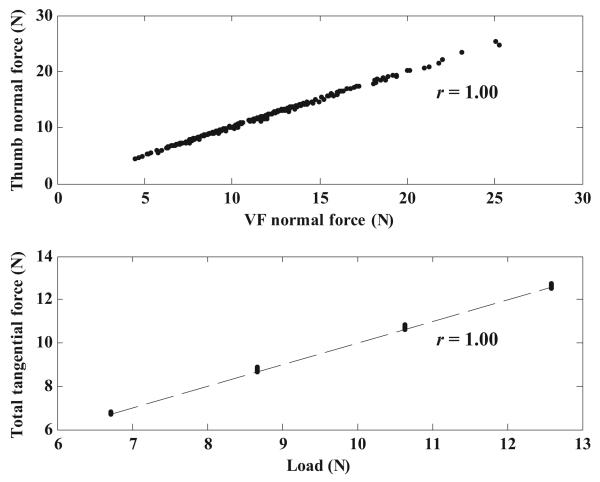
To check the data for accuracy, the following comparisons were regularly performed for all trials: 1) the normal forces of the thumb and VF (these forces should be equal) and 2) the total tangential force and the load (these values should also be equal). The deviations were very small. Exemplary illustrations are presented in Fig. 2.
Fig. 2.
Relations between the thumb and virtual finger (VF) forces. Top graph: thumb normal force vs. VF normal force. Bottom graph: total tangential force vs. load. Results of all trials in the main experiment are plotted. r is the correlation coefficient between the variables.
In the main experiment, the subjects performed 128 trials in 1.5 h. During such a lengthy experiment a trend in the data may occur. For instance, the friction between the digits and the contact materials might change systematically or fatigue might take place. To determine whether such systematic changes occurred we performed three tests. First, a subject held the handle (HHH condition, load 400 g) 128 times. The time course of the experiment was the same as that in the main experiment. There were no systematic changes in the digit forces over the entire period of measurements. The mean of total normal force was 14.07 N and the mean of total tangential force was 8.81 N (the weight of the handle-plus-load assembly was 8.83 N). Second, we averaged the group data for each trial number (i.e., for trial numbers 1, 2, …, 128). Then we regressed the averages on the trial number. There was no systematic trend: the regression coefficient was close to zero. This means that balancing the trials achieved its goal: possible carryover effects were eliminated. Third, we compared the 16 trial observations performed before and after the main experiment. The paired Hotelling's T-squared test showed that there was no significant effect of the 128-trial main experiment on the digit forces and moments (P = 0.271). We concluded that there were no systematic changes in the grasping pattern and digit forces during the experiment.
Results
We present first the data on the local and synergic friction effects and then on the grasping template hypothesis and the triple-product model.
Local and synergy friction effects on digit forces
To answer the question on the existence of the local and synergy effects we analyzed the , , and coefficients. The main conclusion from these analyses is that both the local and synergic effects were present but for different digits the strength of the effects was different.
Coefficients
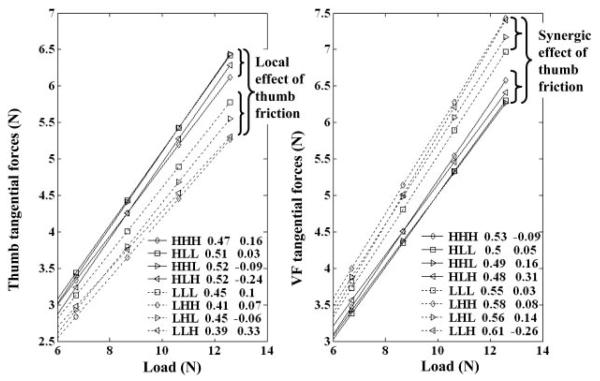
These coefficients denote the sharing of the total tangential force among the digits, a percentage of the load supported by the digit. When the thumb was at a low-friction contact the tangential forces were smaller than those at a high-friction contact; in contrast, the VF forces were larger (Fig. 3). For the thumb this is an example of the local friction effect, whereas for the VF it can be designated as the synergy effect. These opposite-in-sign changes modulated the moment of the tangential forces generated by the thumb and VF (such a moment was previously coined the friction-induced moment; Aoki et al. 2006). The moment will not be analyzed here.
Fig. 3.
Local and synergic effects of the friction at the thumb contact on the tangential forces. Left panel: thumb forces. Right panel: VF forces. Group averages. Each data point is based on average data of 8 subjects (each subject's average is computed from 4 trials). SE varied from 0.082 to 0.256 N and had an average of 0.158 N. SE bars are not shown to avoid a messy picture. Friction sets with the thumb at a low-friction (L) contact (LLL, LHH, LHL, and LLH) are printed with dotted lines. Solid lines represent the tasks with the high-friction (H) contact at the thumb. Left panel: LOCAL FRICTION EFFECT designates the difference induced by the high or low-friction contact at the thumb. Two other smaller figure brackets show the synergic effects, i.e., the effect of FRICTION AT OTHER DIGITS on the thumb force. Numbers in the bottom right insets are the regression coefficients and intercepts (the regression model was used for computations). Small values of the intercepts (between −0.24 and 0.33 N) allowed using in the future Eq. 1, . Values of r2 between and L were ≥0.99 for all friction sets. Compare the right and the left panels: the thumb friction, H or L, induced opposite changes of the thumb and VF forces.
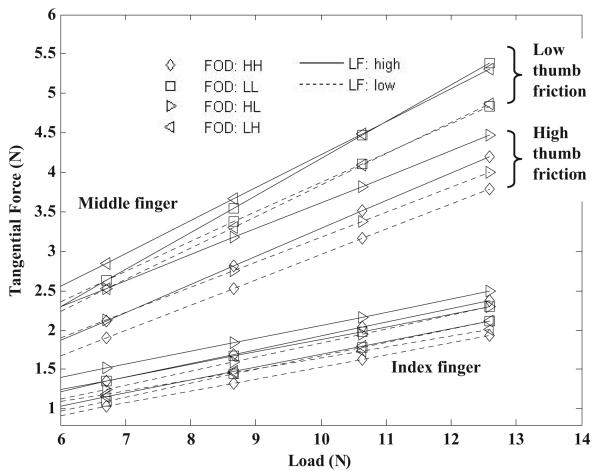
The tangential forces of the index and middle fingers as functions of the load and friction are presented in Fig. 4. The middle finger forces were larger than the index finger forces—i.e., the middle finger served as the main load supporter. The middle finger supported from 55.7 to 73.2% of the VF tangential force (mean = 66.7%). Although there were no visible mechanical reasons for such an unequal force allocation, the CNS nonetheless preferred this sharing pattern. For the middle finger, the synergic effects of the thumb friction can be seen in the figure: at the HH and HL friction conditions the tangential forces were smaller than those in the LL and LH sets.
Fig. 4.
Tangential forces of the index and middle fingers as a function of load and friction. Group averages. Solid or dotted lines designate the local friction: dotted lines, low local friction; solid lines, high local friction. Following symbols designate the friction at other digits: diamonds (◇), high friction at the other two digits; squares (□), low friction at the other two digits; right triangles (▷), high friction at the first digit and low at the second; left triangles (◁), low friction at the first digit and high friction at the second. LF, local friction; FOD, friction at other digits. All r2 values between the tangential forces and the load were >0.97. Note the large differences in the tangential forces of the individual fingers at the same loads but at the different friction sets. Note also the synergic effect of the thumb friction on the middle finger forces: the forces were smaller with the thumb at the high-friction contact (i.e., at the HH and HL friction sets).
The repeated-measures ANOVA results are presented in Table 1 (because of space limitations, only P values are shown in the table). More detailed information on the ANOVA, including the data on the degrees of freedom after the Greenhouse–Geisser correction, is provided in the appendix. The thumb tangential forces were affected statistically significantly both by the friction at the thumb and the friction at other digits. Synergic effects were also significant for the middle finger force. The local effects on the tangential forces of the index and middle fingers did not reach the level of statistical significance. The effects of the LF × FOD interaction were not significant for all the digits.
Table 1.
Two-way repeated-measure ANOVA of coefficients and P-values
| Thumb | Index | Middle | |
|---|---|---|---|
| Local friction (LF, two levels) | 0.005 | 0.17 | 0.068 |
| Friction at other digits (FOD, four levels) | 0.003 | 0.283 | 0 |
| LF × FOD | 0.508 | 0.255 | 0.985 |
Statistically significant P-values (i.e., P < 0.05) are underlined. Values of P < 0.001 are printed as 0. When the requirements for the sphericity of the repeated-measure ANOVA test were violated, the degrees of freedom were corrected with the Greenhouse–Geisser method.
Coefficients
These coefficients denote the digit normal force per unit of the load supported by the digit. In contrast to the coefficients, the coefficients depended significantly on the local friction for all the digits (see Table 2). The effect of friction at other digits was not significant for the index finger.
Table 2.
Two-way repeated-measure ANOVA of coefficients and P-values
| Thumb | Index | Middle | |
|---|---|---|---|
| Local friction (LF, two levels) | 0.003 | 0.011 | 0.028 |
| Friction at other digits (FOD, four levels) | 0.027 | 0.166 | 0.02 |
| LF × FOD | 0.04 | 0.394 | 0.086 |
Statistically significant P-values (i.e., P < 0.05) are underlined. When the requirements for the sphericity of the repeated-measure ANOVA test were violated, the degrees of freedom were corrected with the Greenhouse–Geisser method. The corresponding P-values are printed in italics.
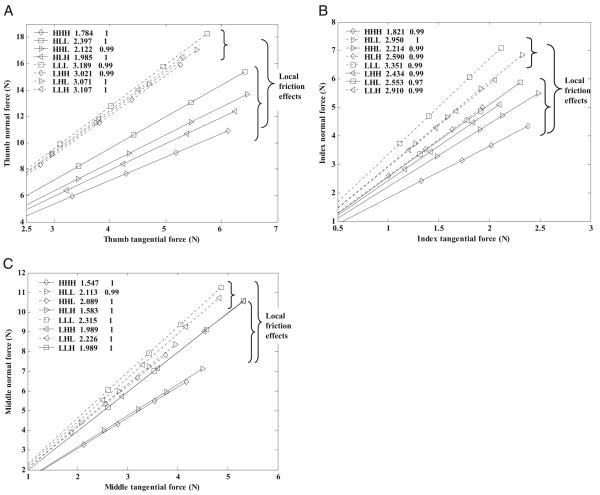
When digit i was at the low-friction contacts, the coefficient was larger than when the digit was at high-friction contacts—i.e., per equal increase of the tangential forces the normal forces increased to a larger degree. Figure 5, A–C shows the normal–tangential force relations for the thumb, index, and middle fingers, respectively.
Fig. 5.
Normal digit forces as a function of tangential forces for different friction sets. Group averages. Dotted lines represent the low-friction contacts at the corresponding digits; the solid lines, high-friction contacts. Numbers in the figures are the coefficients (regression slopes) and the coefficients of correlation squared (r2). Rightmost figure brackets: regression line scattering arising from the local friction effects. Leftmost figure brackets: spreading of the lines at the same local friction (the synergic effects).
The coefficients are the scaling factors that relate normal and tangential digit forces, ensuring that the object does not slip from the hand. Although all the coefficients depended on the local friction they were not completely defined by it. For the thumb and middle finger the synergy effects were statistically significant, indicating that when the CNS selects the coefficients it takes into consideration friction conditions at other digits.
Coefficients
These coefficients denote the digit normal forces per unit of the load supported by all the digits together. The coefficients ignore how the total load is shared among the individual digits. They can be computed directly from the experimental recordings and/or as the products . When both methods were used the results were similar. The two-way repeated-measures ANOVA revealed that both the local friction and the friction at other digits affected the coefficients (with the exception of the local effects on the middle finger force where P = 0.073; Table 3). The synergic effects on the index finger force that were not significant for coefficients and (the P values were only 0.283 and 0.166, respectively; see Tables 1 and 2) were highly significant for the coefficients (P = 0.001).
Table 3.
Two-way repeated-measure ANOVA of coefficients and P-values
| Thumb | Index | Middle | |
|---|---|---|---|
| Local friction (LF, two levels) | 0.002 | 0.018 | 0.073 |
| Friction at other digits (FOD, four levels) | 0.001 | 0.001 | 0 |
| LF × FOD | 0.029 | 0.804 | 0.268 |
Statistically significant P-values (i.e., P < 0.05) are underlined. When the requirements for the sphericity of the repeated-measure ANOVA test were violated, the degrees of freedom were corrected with the Greenhouse–Geisser method. The corresponding P-values are printed in italics. Values of P < 0.001 are printed as 0.
The normal force–load relations for the thumb are shown in Fig. 6. In the tasks where the thumb was in contact with the low-friction surface the thumb forces as well as the coefficients (regression slopes) were larger than those in the tasks with the high-friction contact beneath the thumb. This difference illustrates the LOCAL FRICTION effect found in the repeated-measures ANOVA (see Table 3). The force values and the coefficients for the tasks that correspond to the same contact surface beneath the thumb also differ from each other. Their spreading illustrates the synergy effect—i.e., the effect of friction at the index and middle fingers on the thumb forces.
Fig. 6.
Dependency of the digit normal force on the load for different friction sets. Group averages. At all loads, the group averages are based on 32 individual observations (8 subjects × 4 trials). Numbers in the figure are the coefficients and the coefficients of correlation squared (all r2 ≥ 0.98). A: thumb forces. Friction sets with the thumb at a low-friction contact (LLL, LHH, LHL, and LLH) are printed with dotted lines. Solid lines represent the tasks with the high-friction contact at the thumb. LOCAL FRICTION EFFECT is the scattering induced by the high- or low-friction contact at the thumb. Two other smaller figure brackets show the synergic effects (i.e., the effect of friction at other digits on the thumb force). Two distinct groups were mainly determined by the local friction effect. B: middle finger forces. Dotted lines designate the low-friction contact at the middle finger. Two groups of the regression lines were mainly distinguished by the friction at the thumb (the synergic effect). Two small figure brackets show the local friction effect (i.e., the spreading induced by the high-or low-friction contact at the middle finger). At a given thumb friction, the forces were larger at the low-friction contact at the middle finger. C: index finger force. Dotted lines designate the low-friction contact at the index finger. In contrast to the thumb and the middle finger, the regression lines do not cluster into 2 distinct groups. Largest forces are exerted in the HLL and LLL tasks when both the index and middle finger were at the low-friction contact. Smallest forces were exerted in the HHH task when all the digits contacted high-friction surfaces.
For the middle finger, in contrast to the thumb, the clustering of the regression lines into the two distinct groups was mainly a result of the synergic friction effects of thumb (Fig. 6B). There was no visible clustering for the index finger (Fig. 6C), whereas both the synergic and local effects of friction were statistically significant (see Table 1).
Grasping template and its scaling
The data support a hypothesis that for each friction set the CNS specifies a certain grasping template and scales it with the load magnitude. Note that such a strategy is not necessitated by the task mechanics and represents one of the choices available to the CNS. For instance, one can easily imagine the situation when the sharing of the load force among the digits depends on the load magnitude. To test the grasping template hypothesis we ascertained the accuracy of the triple-product model, which can be seen as a formal description of the hypothesis. The accuracy of the model is understood here as the correspondence between the model estimates and 1) actual data and 2) equilibrium requirements.
Overall, the model approximated the results well: 1) the coefficients of correlation between the independent variables (load or tangential forces) and dependent variables (digit forces) were always >0.99; thus the relations were linear (see Figs. 36); 2) the regression intercepts were small, <1 N (with the exception of , where some of the intercepts slightly exceeded 1 N); 3) the sum of was very close to 1.0 (from 1.0017 to 1.0063), as expected; and 4) the difference was close to zero and thus the handle equilibrium in the horizontal direction was secured. Finally, the correspondence between data predicted from the model and actual normal force data was within acceptable limits: the root mean square was on average 1.157 ± 0.034 N. We concluded that the model accuracy is good enough to support the hypothesis.
Discussion
The experiments have shown the existence of strong local and synergic effects to changes in friction. We discuss first the local and synergic effects of friction and then the triple-product model and the grasping template hypothesis.
Local and synergic adjustments of digit forces
The finding of this study is that digit forces, which are scaled to the supported load, are also adjusted to the LOCAL FRICTION as well as to the FRICTION AT OTHER DIGITS (synergic fine-tuning). In some cases the synergic effects are manifested more strongly than are the adjustments to the local friction (e.g., the middle finger tangential forces; Fig. 6B). Thus if the “coordination of fingertip forces during manipulation emerges from independent neural networks controlling each engaged digit,” as suggested by Burstedt et al. 1997, such a coordination—i.e., a combination of local adjustments to friction—is subordinated to higher levels of control, in particular the synergic fine-tuning. Burstedt et al. (1997) mentioned a higher level of control related to actions before object grasping (selecting grasp configurations and temporal synchronization of digit movements) as well as coping with the weight and size of the object. The present results add to this list the friction at the digit tip–object interfaces and the equilibrium maintenance. The latter means that the synergy adjustments should always be present. Such synergic responses are mechanically necessary; otherwise, the object equilibrium cannot be maintained.
On the whole, the data support an idea that the digit force tuning to the object features arises from both 1) local adjustments to the friction at the particular digit (“independent neural networks”) and 2) overall synergic reactions to the friction at other digits directed at the maintenance of the object equilibrium. It seems plausible that the two mechanisms are realized by different neural pathways. Identification of these pathways is a challenge for future research.
Local and synergic reactions are a general phenomenon of multifinger coordination; they are not limited to force adjustments to local friction. The local and synergic digit force adjustments were previously demonstrated in the experiments in which performers were holding a motorized handle while the handle width was forcibly either increased or decreased (Zatsiorsky et al. 2006). Handle expansion/contraction did not perturb the handle equilibrium; both the resultant force and moment acting on the handle remained the same. To retain the object equilibrium, the simplest strategy for the performer would be to do nothing (i.e., not to change the digit forces). However, when a digit was perturbed a restoring force tending to return the digit to its previous position arose (the local digit force adjustment). The force perturbed the object equilibrium and its action was counterbalanced by the synergic force changes of all the digits.
In the experiment with the motorized handle, the local mechanisms (e.g., stretch reflexes) were directed to keep a digit posture constant; however, they worked against the object equilibrium whereas the synergic force adjustments restored it. In the present experiment, the local force adjustments prevent the object from slipping. Similarly to the previous experiment, they worked against the object equilibrium. The synergic force adjustments kept it at equilibrium.
The template hypothesis
The hypothesis agrees well with the data obtained by Valero-Cuevas (2000) on the voluntary force production by the index finger. In the referenced study, the contributing muscles and their relative activity did not change with the force magnitude. Similar to the present research the force was controlled by scaling the magnitude of a constant coordination pattern.
Presently it is not clear whether scaling of a certain template is a universal mechanism of control in multifinger grasping. For instance, if not only the grasping force but also a moment of force are simultaneously produced and the force demand increases while the torque demand stays constant, a proportional increase of all the digit forces will change not only the grip force but also the moment. This will induce tilting of the object, which is not desirable. Thus it is quite possible that the mechanism of force control found in the present experiment is not universal and is valid only for prehension tasks with zero moment production.
It would be interesting to systematically explore the tasks where the outcome force and torque levels can be or cannot be controlled by proportional scaling of contributing forces. The examples are 1) the joint torques and the contributing muscle forces, 2) endpoint forces and the muscle forces, and 3) the grasping forces and the individual digit forces.
How is a template specified?
The input data in the current experiment can be presented as eight triplets consisting of H and L symbols and the outputs as the eight sets of three and three coefficients. The CNS essentially maps the sets of H and L triplets into the sets of and triplets. The triplets can be determined from products.
Coefficients: Tangential Force Sharing
The strategy used to specify the coefficients evidently includes the following components.
1) Local tangential force adjustments: at the low-friction contact the tangential force decreases and vice versa for the high-friction contact.
2) Synergy reactions that are opposite to the local effects. The synergy adjustments are directed at maintaining the sum of the constant (). If tangential force at digit i decreases, the total tangential force at two other digits, j and k, increases by an equal amount.
The individual tangential forces, in percentages to the supported load, can be presented as the result of algebraic summation of the local and synergic effects. The forces equal to
| (4) |
where is a nominal value of the used as a reference for comparison [e.g., average computed over all eight friction sets]; is the local friction effect at digit i; and are synergic effects of friction at digits j and k on the tangential force of digit i. The sum [] is an overall synergy effect on digit i. ε is an error term that includes possible interaction effects. Equation 4 is similar to the equation of main effects in a multivariate ANOVA. The equation is accurate when the interaction effects are negligibly small. In the present experiment, the LF × FOD interaction effects were not significant for the tangential forces of all three digits (see Table 1). The decomposition according to Eq. 4 allows for computing the magnitude of effects.
To estimate the local and synergic effects we used the following technique: 1) the grand averages of over all eight friction sets were taken as the nominal values (the reference values); 2) the local effects of friction on [i.e., ] were estimated as
| (5) |
where the subscript series h(1,2,3,4) designates the friction sets with high-friction contacts of a given digit and subscript series l(1,2,3,4) designates the sets with low-friction contact of this digit. Then substituting the values of the nominal and in Eq. 4 we obtained the estimate of the overall synergic effects [i.e., , friction at other digits] on the of a given finger (these estimates are compromised by the error terms ε). The average values of across all the friction sets were 0.4653, 0.1764, and 0.3614 for the thumb, index, and middle finger, respectively. Because the coefficients are just sharing percentages we will write them as 46.53, 17.64, and 36.14%, for clarity. The values computed according to Eq. 5 were 3.19, 1.06, and 1.81%, for the thumb, index, and middle finger, respectively. Table 4 illustrates the computation of the synergic effects for the thumb and the middle finger.
Table 4.
Synergic effects on the thumb and the middle finger tangential force sharing (%)
| Thumb |
Middle Finger |
||||
|---|---|---|---|---|---|
| Friction | Actual , % |
Synergic Effect, % |
Friction | Actual , % |
Synergic Effect, % |
| HHH | 48.49 | −1.22 | HHH | 32.60 | −5.35 |
| HLL | 50.65 | 0.94 | HLH | 35.80 | −2.14 |
| HHL | 50.59 | 0.87 | LHH | 41.92 | 3.97 |
| HLH | 49.13 | −0.58 | LLH | 41.47 | 3.52 |
| LLL | 45.69 | 2.34 | HLL | 31.54 | −2.79 |
| LHH | 41.65 | −1.69 | HHL | 29.34 | −4.98 |
| LHL | 43.61 | 0.26 | LLL | 38.35 | 4.02 |
| LLH | 42.40 | −0.93 | LHL | 38.07 | 3.74 |
Computation: Thumb: (i) H contact: Nominal value (grand average) + LEth = 46.53 + 3.19% = 49.72%. Synergic effect from the index and the middle fingers = actual k(1) − 49.72%. (ii) L contact: Nominal value − LEth = 46.53 − 3.19% = 43.34%. Synergic effect from the index and the middle fingers = actual . Middle finger: (i) H contact: Nominal value + LEmi = 36.14 + 1.81% = 37.95%. (ii) L contact: Nominal value (grand average) − LEmi = 36.14 − 1.81% = 34.33%.
From Table 4 it follows that synergic effects of the thumb on the middle finger and vice versa, are similar. The synergic effects are positive (printed in italics) when the second digit is at the low-friction contact (printed in bold); the effects are negative when another digit is at the high-friction contact. In other words, when a digit, such as the thumb, is at the high-friction contact and its tangential force increases the middle finger tangential force decreases. For the HHH task, the decrease (with respect to the overall average of middle finger at high friction) is as large as 5.35%. When the middle finger is at the high-friction contact its sharing percentage is from 32.60 to 41.92% and when this finger is at the low-friction contact the percentage is from 29.34 to 38.35%, depending on the friction at other digits. For the index finger the synergic effects are <1.9% and in four tasks of eight they are <1%.
Coefficients: Overall Friction Equivalents
In two-digit pinch grasps, the CNS adjusts the grasping force to the friction: the lower the friction, the stronger the grasping force (Westling and Johansson 1984). The force is always larger than is absolutely necessary to prevent slipping. Pataky et al. (2004) hypothesized that the CNS uses for the force adjustments not the actual friction coefficient but an “operative friction coefficient,” which is smaller than the actual one—i.e., the CNS “assumes” that the object is more slippery than it actually is. As a result, for the vertical object orientation the relation between the weight of the object and the normal force reduces to a simple proportionality , where fn and ft are the normal and tangential forces, respectively, and scaling factor μo is the “operative friction coefficient.” Similar proportionality was found in this study with the two substantial differences from the previous research: 1) the scaling factors depend on friction at all three digits and 2) they are different for different digits. This is why we coined them the overall friction equivalents (OFEs).
The linearity of the relations between the normal and tangential digit forces (Fig. 5) suggests that for each friction set the CNS uses the same OFE across all the loads. Differently from the tasks in which friction was the same for all engaged digits, in the grasps with complex friction pattern the OFE should somehow be determined from different friction values at different digits. Note that from the perspective of pure mechanics, averaging friction at different contact points does not make sense.
To estimate the local and synergic effects on the same methods as (i.e., Eqs. 4 and 5) were used. The local friction effects on —determined from Eq. 5—were equal to 0.513, 0.204, and 0.347, for the thumb, index, and middle fingers, respectively. This means, for instance, that when the thumb was at the low-friction contact it exerted normal force per unit of tangential force 0.513 N larger than the average force over all friction sets. Because of the space limitations the complete results will not be presented here. We just briefly note that 1) the synergic effects were both positive and negative; as a result of the friction at other digits the values increased in some tasks and decreased in others; 2) in the LLL and HHH tasks the synergic effects were opposite, positive in the LLL task and negative in the HHH task [the values for the thumb were 3.1887 and 1.784, respectively], and 3) for the middle finger the synergic effects were positive when the thumb was at the low-friction contact and they were negative when the thumb was at the high-friction contact—i.e., when the friction at the thumb decreased its normal force increased and as a consequence, the normal force of the middle finger also increased.
Coefficients: Does the Central Controller Uses Generalized Motor Programs?
Conceptually, the coefficients are similar to the grip force:load force ratio broadly used in studies on grasping (Burstedt et al. 1999; Jaric et al. 2005, 2006; Johansson and Westling 1984; Serrien and Wiesendanger 2001). The coefficients are computed, however, for the individual digits, not for the total grip force. They are affected by the entire friction set—i.e., by the friction at all the digits.
We suggest a speculative hypothesis on a sensory origin of the observed patterns of digit force adjustments. These adjustments may be viewed as a particular example of a multidigit prehension synergy; under a synergy we imply a neural organization that adjusts elemental variables (digit forces) to ensure stability of important performance variables such as the total force and total moment of force exerted on the object (cf. Latash et al. 2002, 2004). Several models have been suggested to account for such synergies. Some of them are based on explicit feedback loops from sensory receptors (Todorov and Jordan 2002), whereas others consider back-coupling circuits within the CNS (Latash et al. 2005), or even purely feedforward control schemes (Goodman and Latash 2006). Because the task of the current study was steady state and allowed the action of sensory feedback loops, we will assume that the findings reflect the action of such loops.
Most everyday prehensile tasks, such as holding a glass of liquid, deal with objects whose orientation with respect to gravity is not inherently stable in the hand. Because humans are able to keep such objects vertical without looking at them, we assume that the neural controller tries to balance the force-related sensory signals from the two sides, the thumb and VF. This assumption is indirectly corroborated by experiments that showed the importance of sensory signals from the fingers for the control of grip force (Cole and Johansson 1993; Johansson and Westling 1984). Thus we suggest that the controller tries to keep constant a function STOT (a sensory signal-based estimate of all the forces between the digits and the object)
| (6) |
where S represents sensory functions of force signals; i refers to individual fingers; th stands for the thumb; n and t refer to normal and tangential forces, respectively; and d represents lever arms (external torque is assumed zero). Equation 6 is a sensory counterpart of the requirement for rotational equilibrium. The first term is related to moment produced by normal forces, whereas the second term reflects sensory signals related to moment of tangential forces. Both terms are written to allow nonstatic solutions, such as moving the glass horizontally or vertically while keeping its vertical orientation. This rule may be applicable independently of passive restoring torques that were very large in the current study and typically small in earlier studies (e.g., Shim et al. 2003, 2005) and in most everyday tasks. We assume that, depending on local conditions (e.g., friction), individual S-functions may be adjusted and suggest the following hypothesis: S-functions are smaller for “disadvantaged” fingers, such as for fingers under low friction; in a limit case, when a finger cannot apply any tangential force, its S-function is zero. This hypothesis suggests, in particular, that changing friction under a finger may be accompanied only by changes in the force produced by that finger (local effects) that would keep its S(f) unchanged. However, if a change in an S-function is large (e.g., pressing on a very slippery surface), local force adjustments may be unable to keep the corresponding S(f) unchanged and STOT constant. Then adjustments in other finger forces will be required, effects that we term “synergetic.” Certainly, digit force adjustments have to comply with mechanical constraints. Thus the introduced hypothesis on a sensory origin of digit force adjustments is valid only within a subspace of force variables that are compatible with the mechanical constraints of the task.
In appearance, there is a certain analogy between our findings on proportional force scaling and the theory of generalized motor programs (Schmidt 1975, 1980), although the analogy is questionable. Even if the theory of general motor program were valid (which is doubtful; for discussion see Ostry and Feldman 2003), most probably the CNS has no motor program before performance that is then implemented during the performance. The tasks, such as grasping an object with the HLH or LHL contacts, are rather unusual and were not learned previously. It seems that the CNS determines or computes the grasping template during each trial separately using sensory clues as an input. As follows from the presented data, the computation results do not depend on the supported load and exerted forces; they depend only on the friction triplets. This suggests that the grasping templates and the digit force magnitudes are determined by the different control mechanisms. Revealing the mechanisms that are used by the central controller for mapping friction triplets into the grasping templates remains a challenge.
In summary, so far, the following mechanism of the grasp adjustments to the different object slipperiness has been suggested: when the skin receptors signal that the contact friction is low the central controller increases the grasping force and, if possible, decreases the tangential force at the low-friction contact. This explanation works adequately when friction at all digit tips is the same (symmetric grasps) or it is the same for all fingers but is different for the thumb (asymmetric grasps). In grasps with complex friction patterns, when friction at each digit can differ from friction at other digits, the preceding explanation may still be valid but with limitations; it is evidently not sufficient to explain all the findings. Revealing the mechanisms of the digit force adjustments to the complex friction patterns is a challenging task and this paper is one of the first steps in this direction. The study has demonstrated that 1) there exist regular patterns of the mapping of the friction sets onto the sets of digit forces (in contrast to earlier publications that stated that no clear strategy could be identified that could account for the robust influences by the frictional condition on the load distribution among digits); 2) digit force adjustments can be classified as local and synergic; the obtained data on the local reactions agree with the previous findings for the symmetric and asymmetric grasps; 3) the magnitude of the local and synergic force adjustments was estimated; 4) for each friction set, the central controller selects a grasping template and then scales the template with the load magnitude; 5) a concept of the overall friction equivalent is introduced; and 6) the digit force adjustments to the different friction patterns can be succinctly described with the suggested triple-product model.
These observations have direct implications for two recently suggested hypotheses. In particular, Hasan (2005) suggested that immediate responses to a perturbation could aggravate—rather than counteract—the effects of the perturbation. Our findings of the local and synergic adjustments corroborate this hypothesis. Our proportional scaling model agrees well with findings reported by Valero-Cuevas (2000) showing that index finger force scaling might result from proportional involvement of the muscles contributing to the fingertip force production.
Acknowledgments
The editorial help of M. K. Budgeon is appreciated.
Grants
This work was supported in part by National Institutes of Health Grants AR-048563, AG-018751, and NS-35032
Appendix
Sphericity testing and adjustments of degrees of freedom
Table A1.
Measure:
| Factor | Sum of Squares |
df | Mean Square |
F | Sig. |
|---|---|---|---|---|---|
| Local friction (LF) | |||||
| Sphericity assumed | 0.065 | 1 | 0.065 | 16.498 | 0.005 |
| Greenhouse–Geisser | 0.065 | 1.000 | 0.065 | 16.498 | 0.005 |
| Friction at other digits (FOD) | |||||
| Sphericity assumed | 0.009 | 3 | 0.003 | 6.406 | 0.003 |
| Greenhouse–Geisser | 0.009 | 2.530 | 0.004 | 6.406 | 0.005 |
| LF × FOD | |||||
| Sphericity assumed | 0.001 | 3 | 0.000 | 0.800 | 0.508 |
| Greenhouse–Geisser | 0.001 | 2.068 | 0.001 | 0.800 | 0.472 |
Table A2.
Measure:
| Factor | Sum of Squares |
df | Mean Square |
F | Sig. |
|---|---|---|---|---|---|
| Local friction (LF) | |||||
| Sphericity assumed | 0.007 | 1 | 0.007 | 2.344 | 0.170 |
| Greenhouse–Geisser | 0.007 | 1.000 | 0.007 | 2.344 | 0.170 |
| Friction at other digits (FOD) | |||||
| Sphericity Assumed | 0.007 | 3 | 0.002 | 1.357 | 0.283 |
| Greenhouse–Geisser | 0.007 | 1.842 | 0.004 | 1.357 | 0.290 |
| LF × FOD | |||||
| Sphericity Assumed | 0.002 | 3 | 0.001 | 1.458 | 0.255 |
| Greenhouse–Geisser | 0.002 | 2.202 | 0.001 | 1.458 | 0.264 |
Table A3.
Measure:
| Factor | Sum of Squares |
df | Mean Square |
F | Sig. |
|---|---|---|---|---|---|
| Local friction (LF) | |||||
| Sphericity Assumed | 0.021 | 1 | 0.021 | 4.639 | 0.068 |
| Greenhouse–Geisser | 0.021 | 1.000 | 0.021 | 4.639 | 0.068 |
| Friction at Other Digits | |||||
| Sphericity Assumed | 0.099 | 3 | 0.033 | 13.837 | 0.000 |
| Greenhouse–Geisser | 0.099 | 1.856 | 0.053 | 13.837 | 0.001 |
| LF *FOD | |||||
| Sphericity Assumed | 0.000 | 3 | 0.000 | 0.050 | 0.985 |
| Greenhouse–Geisser | 0.000 | 2.119 | 0.000 | 0.050 | 0.958 |
Table B1.
Measure:
| Factor | Sum of Squares |
df | Mean Square |
F | Sig. |
|---|---|---|---|---|---|
| Local friction | |||||
| Sphericity Assumed | 17.692 | 1 | 17.692 | 20.886 | 0.003 |
| Greenhouse–Geisser | 17.692 | 1.000 | 17.692 | 20.886 | 0.003 |
| Friction at Other Digits | |||||
| Sphericity Assumed | 1.193 | 3 | 0.398 | 3.720 | 0.027 |
| Greenhouse–Geisser | 1.193 | 2.320 | 0.514 | 3.720 | 0.041 |
| LF *FOD | |||||
| Sphericity Assumed | 0.475 | 3 | 0.158 | 3.307 | 0.040 |
| Greenhouse–Geisser | 0.475 | 2.108 | 0.225 | 3.307 | 0.063 |
Table B2.
Measure:
| Factor | Sum of Squares |
df | Mean Square |
F | Sig. |
|---|---|---|---|---|---|
| Local friction | |||||
| Sphericity Assumed | 8.330 | 1 | 8.330 | 11.603 | 0.011 |
| Greenhouse–Geisser | 8.330 | 1.000 | 8.330 | 11.603 | 0.011 |
| Friction at Other Digits | |||||
| Sphericity Assumed | 18.536 | 3 | 6.179 | 2.276 | 0.109 |
| Greenhouse–Geisser | 18.536 | 1.253 | 14.791 | 2.276 | 0.166 |
| LF *FOD | |||||
| Sphericity Assumed | 3.778 | 3 | 1.259 | 0.857 | 0.479 |
| Greenhouse–Geisser | 3.778 | 1.098 | 3.442 | 0.857 | 0.394 |
Table B3.
Measure:
| Factor | Sum of Squares |
df | Mean Square |
F | Sig. |
|---|---|---|---|---|---|
| Local friction | |||||
| Sphericity Assumed | 3.906 | 1 | 3.906 | 7.689 | 0.028 |
| Greenhouse–Geisser | 3.906 | 1.000 | 3.906 | 7.689 | 0.028 |
| Friction at Other Digits | |||||
| Sphericity Assumed | 1.381 | 3 | 0.460 | 7.930 | 0.001 |
| Greenhouse–Geisser | 1.381 | 1.158 | 1.192 | 7.930 | 0.020 |
| LF *FOD | |||||
| Sphericity Assumed | 0.407 | 3 | 0.136 | 2.515 | 0.086 |
| Greenhouse–Geisser | 0.407 | 2.077 | 0.196 | 2.515 | 0.114 |
Table C1.
Measure:
| Factor | Sum of Squares |
df | Mean Square |
F | Sig. |
|---|---|---|---|---|---|
| Local friction | |||||
| Sphericity Assumed | 1.551 | 1 | 1.551 | 24.342 | 0.002 |
| Greenhouse–Geisser | 1.551 | 1.000 | 1.551 | 24.342 | 0.002 |
| Friction at Other Digits | |||||
| Sphericity Assumed | 0.633 | 3 | 0.211 | 8.847 | 0.001 |
| Greenhouse–Geisser | 0.633 | 2.031 | 0.312 | 8.847 | 0.003 |
| LF *FOD | |||||
| Sphericity Assumed | 0.058 | 3 | 0.019 | 3.642 | 0.029 |
| Greenhouse–Geisser | 0.058 | 1.911 | 0.030 | 3.642 | 0.056 |
Table C2.
Measure:
| Factor | Sum of Squares |
df | Mean Square |
F | Sig. |
|---|---|---|---|---|---|
| Local friction | |||||
| Sphericity Assumed | 0.079 | 1 | 0.079 | 9.338 | 0.018 |
| Greenhouse–Geisser | 0.079 | 1.000 | 0.079 | 9.338 | 0.018 |
| Friction at Other Digits | |||||
| Sphericity Assumed | 0.199 | 3 | 0.066 | 18.013 | 0.000 |
| Greenhouse–Geisser | 0.199 | 1.342 | 0.148 | 18.013 | 0.001 |
| LF *FOD | |||||
| Sphericity Assumed | 0.003 | 3 | 00018 | 0.330 | 0.804 |
| Greenhouse–Geisser | 0.003 | 1.942 | 0.002 | 0.330 | 0.719 |
Table C3.
Measure:
| Factor | Sum of Squares |
df | Mean Square |
F | Sig. |
|---|---|---|---|---|---|
| Local friction | |||||
| Sphericity Assumed | 0.081 | 1 | 0.081 | 4.452 | 0.073 |
| Greenhouse–Geisser | 0.081 | 1.000 | 0.081 | 4.452 | 0.073 |
| Friction at Other Digits | |||||
| Sphericity Assumed | 1.117 | 3 | 0.372 | 23.014 | 0.000 |
| Greenhouse–Geisser | 1.117 | 1.114 | 1.004 | 23.014 | 0.001 |
| LF *FOD | |||||
| Sphericity Assumed | 0.022 | 3 | 0.007 | 1.410 | 0.268 |
| Greenhouse–Geisser | 0.022 | 2.292 | 0.010 | 1.410 | 0.275 |
References
- Aoki T, Latash ML, Zatsiorsky VM. Adjustments to different local friction in multi-finger prehension. Brain Res. 2007;0000:000–000. [Google Scholar]
- Aoki T, Niu X, Latash ML, Zatsiorsky VM. Effects of friction at the digit-object interface on the digit forces in multi-finger prehension. Exp Brain Res. 2006;172:425–438. doi: 10.1007/s00221-006-0350-9. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Arbib MA, Iberall T, Lyons D. Coordinated control programs for movements of the hand. In: Goodwin AW, Darian-Smith I, editors. Hand Function and the Neocortex. Springer-Verlag; New York: 1985. pp. 111–129. [Google Scholar]
- Baud-Bovy G, Soechting JF. Two virtual fingers in the control of the tripod grasp. J Neurophysiol. 2001;86:604–615. doi: 10.1152/jn.2001.86.2.604. [DOI] [PubMed] [Google Scholar]
- Birznieks I, Burstedt MK, Edin BB, Johansson RS. Mechanisms for force adjustments to unpredictable frictional changes at individual digits during two-fingered manipulation. J Neurophysiol. 1998;80:1989–2002. doi: 10.1152/jn.1998.80.4.1989. [DOI] [PubMed] [Google Scholar]
- Burstedt MK, Birznieks I, Edin BB, Johansson RS. Control of forces applied by individual fingers engaged in restraint of an active object. J Neurophysiol. 1997;78:117–128. doi: 10.1152/jn.1997.78.1.117. [DOI] [PubMed] [Google Scholar]
- Burstedt MK, Flanagan JR, Johansson RS. Control of grasp stability in humans under different frictional conditions during multidigit manipulation. J Neurophysiol. 1999;82:2393–2405. doi: 10.1152/jn.1999.82.5.2393. [DOI] [PubMed] [Google Scholar]
- Cadoret G, Smith AM. Friction, not texture, dictates grip forces used during object manipulation. J Neurophysiol. 1996;75:1963–1969. doi: 10.1152/jn.1996.75.5.1963. [DOI] [PubMed] [Google Scholar]
- Cole KJ, Johansson RS. Friction at the digit-object interface scales the sensorimotor transformation for grip responses to pulling loads. Exp Brain Res. 1993;95:523–532. doi: 10.1007/BF00227146. [DOI] [PubMed] [Google Scholar]
- Edin BB, Westling G, Johansson RS. Independent control of human fingertip forces at individual digits during precision lifting. J Physiol. 1992;450:547–564. doi: 10.1113/jphysiol.1992.sp019142. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Goodman SR, Latash ML. Feedforward control of a redundant motor system. Biol Cybern. 2006;95:271–280. doi: 10.1007/s00422-006-0086-4. [DOI] [PubMed] [Google Scholar]
- Hasan Z. The human motor control system's response to mechanical perturbation: should it, can it, and does it ensure stability? J Mot Behav. 2005;37:484–493. doi: 10.3200/JMBR.37.6.484-493. [DOI] [PubMed] [Google Scholar]
- Iberall T. The nature of human prehension: three dexterous hands in one; Proc 1987 IEEE Int Conf on Robotics and Automation; Raleigh, NC. 1987. pp. 396–401. [Google Scholar]
- Jaric S, Collins JJ, Marwaha R, Russell E. Interlimb and within limb force coordination in static bimanual manipulation task. Exp Brain Res. 2006;168:88–97. doi: 10.1007/s00221-005-0070-6. [DOI] [PubMed] [Google Scholar]
- Jaric S, Russell EM, Collins JJ, Marwaha R. Coordination of hand grip and load forces in unidirectional and bidirectional static force production tasks. Neurosci Lett. 2005;381:51–56. doi: 10.1016/j.neulet.2005.01.086. [DOI] [PubMed] [Google Scholar]
- Johansson RS. Sensory control of dexterous manipulation. In: Wing AM, Haggard P, Flanagan JR, editors. The Neurophysiology and Psychology of Hand Movements. Academic Press; Toronto: 1996. pp. 381–414. [Google Scholar]
- Johansson RS, Westling G. Roles of glabrous skin receptors and sensorimotor memory in automatic control of precision grip when lifting rougher or more slippery objects. Exp Brain Res. 1984;56:550–564. doi: 10.1007/BF00237997. [DOI] [PubMed] [Google Scholar]
- Johansson RS, Westling G. Signals in tactile afferents from the fingers eliciting adaptive motor responses during precision grip. Exp Brain Res. 1987;66:141–154. doi: 10.1007/BF00236210. [DOI] [PubMed] [Google Scholar]
- Kinoshita H, Kawai S, Ikuta K. Contributions and co-ordination of individual fingers in multiple finger prehension. Ergonomics. 1995;38:1212–1230. doi: 10.1080/00140139508925183. [DOI] [PubMed] [Google Scholar]
- Latash ML, Danion F, Scholz JP, Schoner G. Coordination of multielement motor systems based on motor abundance. In: Latash ML, Levin MF, editors. Progress in Motor Control. Effects of Age, Disorder, and Rehabilitation. Vol. 3. Human Kinetics; Champaign, IL: 2004. pp. 97–124. [Google Scholar]
- Latash ML, Scholz JP, Schoner G. Motor control strategies revealed in the structure of motor variability. Exerc Sport Sci Rev. 2002;30:26–31. doi: 10.1097/00003677-200201000-00006. [DOI] [PubMed] [Google Scholar]
- Latash ML, Shim JK, Smilga AV, Zatsiorsky VM. A central back-coupling hypothesis on the organization of motor synergies: a physical metaphor and a neural model. Biol Cybern. 2005;92:186–191. doi: 10.1007/s00422-005-0548-0. [DOI] [PMC free article] [PubMed] [Google Scholar]
- Murray RM, Li Z, Sastry SS. A Mathematical Introduction to Robotic Manipulation. CRC Press; Boca Raton, FL: 1994. [Google Scholar]
- Ostry DJ, Feldman AG. A critical evaluation of the force control hypothesis in motor control. Exp Brain Res. 2003;221:275–288. doi: 10.1007/s00221-003-1624-0. [DOI] [PubMed] [Google Scholar]
- Pataky T, Latash ML, Zatsiorsky VM. Tangential load sharing among fingers during prehension. Ergonomics. 2004;47:876–889. doi: 10.1080/00140130410001670381. [DOI] [PubMed] [Google Scholar]
- Quaney BM, Cole KJ. Distributing vertical forces between the digits during gripping and lifting: the effects of rotating the hand versus rotating the object. Exp Brain Res. 2004;155:145–155. doi: 10.1007/s00221-003-1711-2. [DOI] [PubMed] [Google Scholar]
- Santello M, Soechting JF. Force synergies for multifingered grasping. Exp Brain Res. 2000;133:457–467. doi: 10.1007/s002210000420. [DOI] [PubMed] [Google Scholar]
- Schmidt RA. A schema theory of discrete motor skill learning. Res Q Exerc Sport. 1975;82:225–260. doi: 10.1080/02701367.1981.10607893. [DOI] [PubMed] [Google Scholar]
- Schmidt RA. Past and future issues in motor programming. Psychol Rev. 1980;51:122–140. doi: 10.1080/02701367.1980.10609279. [DOI] [PubMed] [Google Scholar]
- Serrien DJ, Wiesendanger M. Bimanual organization of manipulative forces: evidence from erroneous feedforward programming of precision grip. Eur J Neurosci. 2001;13:1825–1832. doi: 10.1046/j.0953-816x.2001.01548.x. [DOI] [PubMed] [Google Scholar]
- Todorov E, Jordan MI. Optimal feedback control as a theory of motor coordination. Nat Neurosci. 2002;5:1226–1235. doi: 10.1038/nn963. [DOI] [PubMed] [Google Scholar]
- Valero-Cuevas FJ. Predictive modulation of muscle coordination pattern magnitude scales fingertip force magnitude over the voluntary range. J Neurophysiol. 2000;83:1469–1479. doi: 10.1152/jn.2000.83.3.1469. [DOI] [PubMed] [Google Scholar]
- Westling G, Johansson RS. Factors influencing the force control during precision grip. Exp Brain Res. 1984;53:277–284. doi: 10.1007/BF00238156. [DOI] [PubMed] [Google Scholar]
- Zatsiorsky VM. Kinetics of Human Motion. Human Kinetics; Champaign, IL: 2002. pp. 3–75. [Google Scholar]
- Zatsiorsky VM, Gao F, Latash ML. Prehension stability: experiments with expanding and contracting handle. J Neurophysiol. 2006;95:2513–2529. doi: 10.1152/jn.00839.2005. [DOI] [PMC free article] [PubMed] [Google Scholar]