Table 1.

Bifurcation analysis and unstable periodic solutions of repressilator rings with even number of genes. We use the continuation package Auto (Doedel 2007) to obtain the bifurcations of rings of size n (1). The parameter c, defined in equation (2.2), is swept in the biologically relevant range c ∈ [0.001,30] by changing c₁ with c₂ = 0.12, c₃ = 0.16 and c₄ = 0.06 constant. In agreement with analytical calculations, a branching point corresponding to a pitchfork bifurcation (P) is found at c = 2. A series of HBs linked to the emergence of unstable periodic solutions are found subsequently. Floquet analysis indicates that the first unstable orbit to emerge has only one unstable direction, regardless of the dimension of the system, and that the maximal Floquet multiplier decreases with increasing n. Hence, this periodic solution is quasi-stable: if it is reached, the divergence away from it is slow, and gets slower for longer rings. Other unstable orbits are present but their high instability makes them irrelevant to the observed dynamics. A similar structure of unstable orbits exists in odd rings (see the electronic supplementary material). The figure on the right shows the bifurcation diagrams for even rings of length n = 6, 12, 16. The unstable periodic orbits, shown as dark grey dashed lines, emerge through HBs.

n	bifurcation	c	stable/all directions	max. Floquet	period (min)
2	P	2.00	—	—	—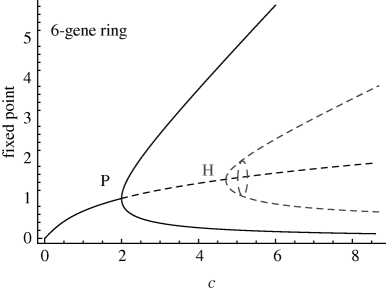
4	P	2.00	—	—	—
6	P	2.00	—	—	—
	HB	4.68	11/12	6.2	132
8	P	2.00	—	—	—
	HB	3.00	15/16	3.9	186
10	P	2.00	—	—	—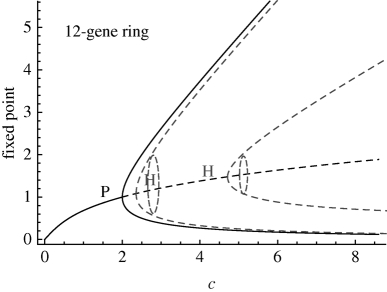
	HB	2.56	19/20	3.0	239
	HB	9.62	17/20	9.0	104
12	P	2.00	—	—	—
	HB	2.36	23/24	2.5	291
	HB	4.68	21/24	6.2	132
14	P	2.00	—	—	—
	HB	2.24	27/28	2.2	342
	HB	3.51	25/28	4.8	160
	HB	16.9	23/28	10.5	94
16	P	2.00	—	—	—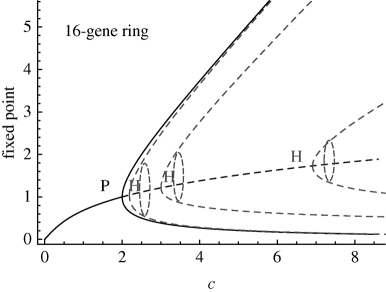
	HB	2.18	31/32	2.0	393
	HB	3.00	29/32	3.9	186
	HB	6.91	27/32	7.8	113
18	P	2.00	—	—	—
	HB	2.15	35/36	1.8	444
	HB	2.71	33/36	3.4	213
	HB	4.71	31/36	6.2	132
	HB	27.33	29/36	11.5	89