Comparison of 3D joint moments using local and global inverse dynamics approaches among three different age groups

Abstract

A complete understanding of joint moment is important to locomotion research. The purpose of the study was to compare stance phase lower extremity joint moments, calculated by a three-dimensional (3D) inverse dynamics model and expressed in global and local coordinate systems, to examine the influence of different coordinate systems on joint moment profiles. Additionally, aging influences on joint moments were examined. Thirty healthy (10 young, 10 middle-aged and 10 old) participants were involved in the current study. Kinematic and kinetic data were collected using standard gait study protocol. Results suggested that globally expressed joint moments were significantly different than those expressed locally. Furthermore, significant moment differences were found between young and old age groups. The older adults produced less evertor muscle moments at the ankle joint. However, aging effect was not significant for majority of the joint moment comparisons. It is concluded that coordinate system need to be carefully chosen, and specified in 3D joint moment analysis, while significant error introduced by using 2D analysis need to be considered.

1. Introduction

A complete understanding of joint moment is important in the general study of human locomotion. Sagittal plane joint moments estimated by using two-dimensional (2D) inverse dynamics analysis has been a popular and useful tool in understanding the mechanisms of human gait [1–3]. This is a simplified procedure, which requires only one camera to capture human movement, a few marker position data to define joint centers and center of mass (COM) locations, and a fixed coordinate system to interpret the results.

Recently, three-dimensional (3D) inverse dynamics analyses of sagittal plane joint moments have been presented [4–5]. Alkjaer et al. [4] compared the ankle, knee and hip joint moments in sagittal plane utilizing two- and three-dimensional models during normal walking and concluded that the simpler 2D approach seems appropriate for gait analysis because little differences were found in the overall joint moment patterns between the 2D and 3D models. Nevertheless, the sagittal view provides only part of the information. This is especially true at the hip joint where hip abductor moments play an important role in maintaining trunk balance in the frontal plane [6].

Several studies were also conducted to reveal joint moments in three planes (sagittal, frontal and transverse) using 3D inverse dynamics approach [7–10]. While intersubject variability has been examined in these studies, no age group differences in three dimensional analyses were addressed, which led to the necessity of comparing 3D joint moments among different age groups in the current study. Furthermore, lower extremity joint moments were found to be an important variable explaining age-related musculoskeletal deterioration. It is also believed that 3D joint moment analysis will further aid in answering the fundamental questions associated with age-related slip-induced fall accidents.

To describe human motion in space, appropriate coordinate systems have to be adopted in 3D inverse dynamics analysis. Various coordinate systems categorized as global coordinate system (also referred to as lab-fixed coordinate) and local coordinate system (also referred to as body-fixed coordinate) have been utilized in previous studies. Local coordinate system can be constructed using fixed triads located on each segment or derived from mathematical approach based on body landmarks [7–8,11–14]. It has been proposed that different coordinate systems (i.e., local versus global) affect the accuracy of joint moment calculation as well as the interpretation of results [4]. However, 3D lower extremity joint moment differences introduced by different coordinate systems have not been fully addressed.

The purpose of the present study was to compare 3D lower extremity joint moment profiles expressed in both global and local coordinate systems during stance phase in order to answer the following questions. (a) Does global and local coordinate systems affect the 3D joint moments? (b) What are the differences of 3D joint moments among three age groups during normal walking? Based on the evidence that joint moment generation may be influenced by the individual's walking speed [15–16], the effect of walking velocity will be examined along with aging effect in this study. It was hypothesized that under different coordinate systems, the sagittal joint moment profiles will be similar while significant differences will be found in the two other planes of reference (frontal and transverse). Additionally, we hypothesize that the elderly will generate less joint moments than their younger counterparts.

2. Methods

A total of 30 healthy participants (Table 1) in three age groups (10 young, 10 middle-aged and 10 old) were involved in the walking experiment. All of these participants gave their informed consent, which was approved by the Institute Review Board of Virginia Tech. All participants were screened for past musculoskeletal and neurological disease and injury.

Table 1.

Means and ranges of participants' anthropometric measurement

	Young	Middle-aged	Elderly
Age (years)	23.5a (19–35)b	46.2 (40–54)	72.6 (68–86)
Height (cm)	173.39 (156.8–191.5)	171.09 (159.7–182.5)	166.09 (154.8–179)
Weight (kg)	72.32 (59–99.6)	78.82 (56.7–117)	72.93 (53.5–92)

^aMean.

^bRange.

The participants were instructed to walk across a linear walking track (1.5 m × 15.5 m) embedded with two force platforms (BERTEC #K80102, Type 45550-08, Bertec Corporation, OH 43212, USA) at their natural gait speed. The floor of the walking track was covered with vinyl floor surface (Armstrong). Normalization period (>10 min of normal walking) was introduced to each participant to ensure natural gait characteristic before any data collection [17]. The participants were dressed in a tight shirt and shorts and provided standard experimental shoes for realistic consideration and to minimize shoe sole differences. Twenty-six small spherical reflecting markers were placed according to the marker set-up described by Lockhart et al. [17] (please refer to Electronic addendum 1 for details). A six-camera ProReflex system (Qualysis) was used to collect the three-dimensional posture data of the participants as they walked over the dry vinyl floor surface. Kinematic and force-plate data were recorded at 120 Hz and 1200 Hz, and digitally low-pass filtered by a zero-phase fourth-order Butterworth filter with a cut-off frequency of 6 Hz and 12 Hz, respectively.

A 3D biomechanical model was constructed to compute net joint moments around the lower extremity joint centers (please refer to Electronic addendum 2 for details on rotation sequence) during stance phase with respect to the anatomical axes. Ankle and knee joint centers were defined as the midpoints between medial and lateral malleolus and condyle markers, respectively. Hip joint center was defined as the 37% close to the proximal great trochanter marker. The 3D inverse dynamics model was based on the free-body segment method [18]. The global coordinate system was constructed based upon the fixed laboratory coordinate which is identical with the coordinate space utilized in the motion capture system.

The local coordinate system is essentially an orthogonal space in geometry. Because no fixed triads were used, the Gram-Schmidt orthogonalization process [19], together with limited marker position data from each segment, was utilized to construct local segmental coordinate system. Given an arbitrary basis {α₁, α₂, α₃} for a three-dimensional inner product space V, the Gram-Schmidt orthogonalization process [20] constructs an orthogonal basis {γ₁, γ₂, γ₃} for V. Two vectors γ₂ and γ₃, which are both orthogonal to the γ₁, are computed as:

$${\gamma }_2={\alpha }_2-\frac{\langle {\alpha }_2,{\gamma }_1\rangle }{{\Vert {\gamma }_1\Vert }^2}{\gamma }_1,$$

$${\gamma }_3={\alpha }_3-\frac{\langle {\alpha }_3,{\gamma }_1\rangle }{{\Vert {\gamma }_1\Vert }^2}{\gamma }_1-\frac{\langle {\alpha }_3,{\gamma }_2\rangle }{{\Vert {\gamma }_2\Vert }^2}{\gamma }_2$$

Referring to the musculoskeletal model, for each segment, at least two non-parallel vectors approximately located in frontal plane can be established. Furthermore, three principle axes {γ₁, γ₂, γ₃} for each segment can be derived consequently, where γ₁ represents the Z-axis and the variables α₂ and α₃ are defined as those two vectors that are not scalar multiples of γ₁, γ₂ or γ₃.

For example, at the ankle joint, the flexion/extension (F/E) axis of the local system intersected the malleoli. The longitudinal axis about which the internal/external rotation (Int/Ext) moment was expressed originated at the ankle joint center and projected towards the heel marker, using Gram-Schmidt orthogonalization process. At the knee joint, the F/E axis intersected the epicondyles. The longitudinal axis about which the Int/Ext moment was expressed originated at the knee joint center and projected towards the ankle joint center. At the hip joint, the longitudinal axis was defined as the connection between the hip and knee joint centers. The F/E axis originated at the hip joint center and projected towards the great trochanter marker. The adduction/abduction (Add/Abd) axes of ankle, knee and hip were all defined by the cross product of the corresponding Int/Ext and F/E axes.

One representative gait cycle was selected for each participant. Joint moments were calculated using a custom written MATLAB (The MathWorks, Inc., Natick, MA, USA) program. Cubic spline interpolation method was employed to extend the resulting curves. Stance phase was extracted and scaled to 100% and an ensemble average was created by averaging multi curves. Joint moments were normalized to the participant's weight.

Six percentages (0%, 20%, 40%, 60%, 80% and 100%) from each data curve of each participant were collected for statistical analysis. Coordinate system (global and local) effect on joint moments was tested using paired t-test. A Bonferroni adjustment for multiple tests was performed in order to reduce the probability of committing a Type I error. The adjusted alpha level for the coordinate system effect test was set at 0.0083 (i.e. 0.05/6). Aging effect (young, middle-aged and old) was tested using ANCOVA with walking velocity as a covariate. For all pairs, Turkey–Kramer HSD test was applied as post-hoc test. Significant level p < 0.05 was utilized for the aging effect tests.

3. Results

3.1. 3D joint moment patterns

Sagittal plane joint moment patterns expressed in both global and local coordinates were in general agreement with previous literature [4,7,9,10]. Frontal and transverse plane results, however, showed considerable large variances.

At the ankle joint (Fig. 1), moments occurred mainly in the sagittal plane. The planterflexor was dominant in controlling the forward rotation of the tibia after landing phase. Sagittal plane joint moment patterns were consistent across all the participants while both transverse and frontal joint moments varied from person to person.

Fig. 1.

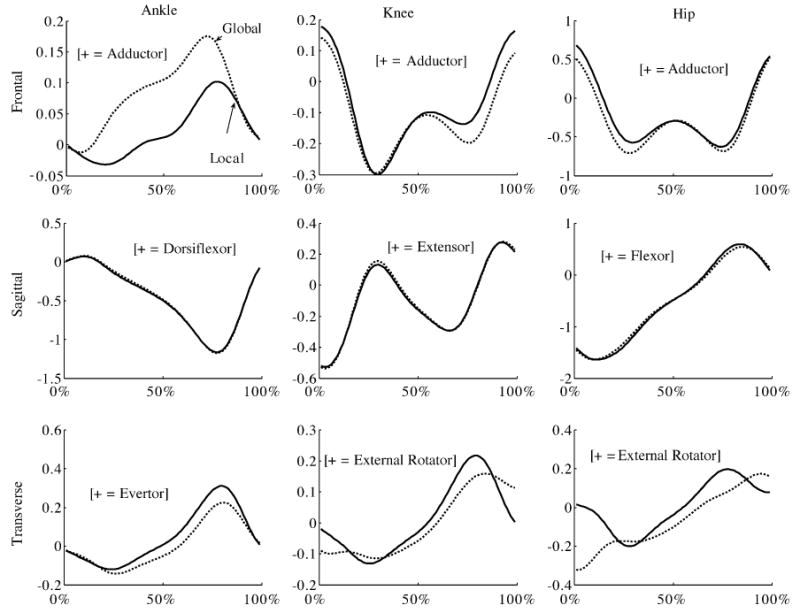
Normalized joint moments (N m/kg) about ankle, knee and hip in frontal, sagittal and transverse planes under global and local coordinates. X-axis represents relative time, with 0% indicating heel contact and 100% indicating toe-off. The curves represent averaged global joint moments (—) and averaged local joint moments (⋯).

At the knee joint (Fig. 1), frontal plane moments increased almost to the level of sagittal plane moments. At the same time, frontal patterns were consistent across all the participants unlike ankle frontal joint moment patterns. A stabilizing knee abductor moment was generated to counter the stress from the upper body weight. Transverse plane moments were relatively less demanding in terms of the magnitude, and the pattern even reversed because of individual differences.

At the hip joint (Fig. 1), frontal and sagittal moment patterns were consistent across all the participants. During landing phase, extensor muscle moment climbed to the peak rapidly to move body center of mass forward, and during push off phase, flexor muscle moment was dominant to pull connected lower extremity forward. The large hip abductor muscle moment during stance phase countered the upper body which was medial to the stance hip.

3.2. Joint moments under global and local coordinates

Significant coordinate effect was found in majority of joint moment percentage comparisons.

At the ankle joint, frontal joint moments expressed in global coordinate were significantly greater than joint moments expressed in local coordinate at 20%, 40% and 60%. This result suggested that global coordinate tended to overestimate the adductor muscle moments in the middle of the stance phase (in comparison to local adductor moments). In the sagittal plane, coordinate system effect was found to be significant at 0%, 20% and 40%, though the mean joint moment curves (Fig. 1) in sagittal plane were closely matched. At 20% and 40%, sagittal moments expressed in global coordinate were significantly lower than sagittal moments expressed in local coordinate. This result suggested planterflexor muscle moments were underestimated in global coordinate at the beginning of stance phase. In the transverse plane, joint moments expressed in global coordinate were significantly greater than joint moments expressed locally at 40%, 60% and 80%. Again, global coordinate underestimated the role of evertor muscle moments in the transverse plane (in comparison to local transverse moments).

At the knee joint, coordinate system effect was found to be significant across the entire frontal joint moment curve. Besides, as shown in Fig. 1, joint moments expressed in global coordinate were lower than those expressed in local coordinate, which made it clear that adductor muscle moments were underestimated during the entire stance phase. In the sagittal plane, mean joint moment curves expressed in both coordinates were closely matched, though significant effect was still found at 20%, 40% and 100%. Greater joint moments found in global coordinate at these percentages indicated the amplified extensor muscle moments in frontal plane. In the transverse plane, coordinate system effect was significant across the entire moment curve. Specifically, joint moments expressed in global coordinate were less than those expressed in local coordinate at 0%, 40%, 60% and 80%. However, opposite results existed at 20% and 100%. Considering Fig. 1, transverse knee joint moment patterns were altered between global and local coordinates.

At the hip joint, coordinate systems significantly affected the entire moment curves across all three planes. In the frontal plane, significant lower global joint moments indicated the underestimation of adductor muscle moments in global coordinate, which were also observed in frontal knee joint moments. In the sagittal plane, global moments were significantly less at 20% and at 80%, where peak extensor and flexor moments occurred, than local moments. This result suggested peak hip sagittal moments were underestimated in global coordinate. In the transverse plane, moment patterns were altered in a way similar to knee transverse joint moments. Especially at the instants of heel-contact and toe-off, joint moments expressed in local coordinate were approaching zero, while those expressed in global coordinate were still present with large magnitudes.

3.3. Joint moment differences among age groups

Walking velocity for each age group was summarized as following (mean/S.D.): young, 134.74/12.69 cm/s; middle-aged, 142.92/11.71 cm/s; old, 125.44/15.83 cm/s.

Overall, no significant age group effect was found among most of the data comparisons, even though the mean joint moment curves (Fig. 2) showed large disagreement among age groups. For the several significant comparisons, young participants generally produced greater joint moments than their older counterparts.

Fig. 2.

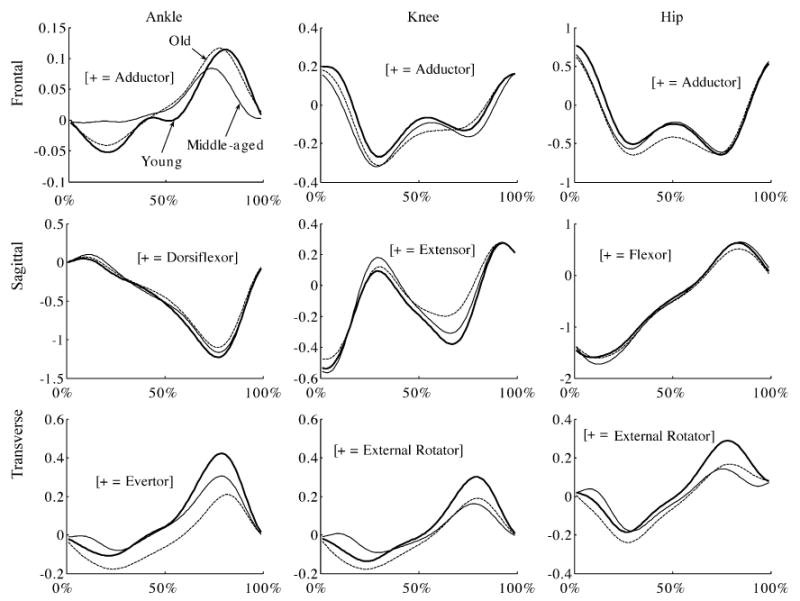
Normalized joint moments (N m/kg) about ankle, knee and hip in frontal, sagittal and transverse planes between young, middle-aged and elderly group under local coordinate. X-axis represents relative time, with 0% indicating heel contact and 100% indicating toe-off. The curves represent younger (—), middle-aged (—) and older (⋯) age groups.

At the ankle joint, no significant age group effect was found in both frontal and sagittal planes. In the transverse plane, aging effect was found at 40% and 60% with walking speed as a covariate. Furthermore, Turkey–Kramer HSD test showed joint moments produced by both young and middle-aged groups were greater than those produced by the old group, though no significant difference was found between young and middle-aged groups. This result suggested that old people produced less ankle evertor muscle moments than young and middle-aged people.

At the knee joint, no significant aging effect was evident at all the percentages that were tested in frontal plane. At 80% in the sagittal plane, aging effect was present. Post-hoc test showed the old individuals generated greater extensor moments than the younger individuals. In addition, aging effect was also found at 40% in the transverse plane. Furthermore, young and middle-aged participants were tested to generate less internal rotator moments than their old counterparts.

At the hip joint, aging effect was found to be significant at 0% in the frontal plane. Post-hoc test at 0% indicated young group generated greater adductor muscle moments than their old counterparts. At 20% and 60% in the frontal plane, both age group and walking speed had a significant effect. No statistical difference was found among age groups in the sagittal plane. In the transverse plane, the only age group effect was evident at 40%, where post-hoc test indicated young group generated less internal rotator moments than their old counterparts.

4. Discussion

The objective of current study was to indicate the possible influence of local and global coordinate system on the joint moment expressions. Locally expressed joint moments were hypothesized to be more meaningful because human planar motion would no longer hold in unusual gait conditions such as human responses to slips and falls accidents. Application of Gram-Schmidt orthogonalization process in local coordinate construction and investigation of aging effect was also thought to facilitate complete understanding of human gait.

Two important points are discussed in evaluating the 3D joint moment profiles under local and global coordinates. First, the variance or the range of joint moments for each of the specific moment profile is relatively large. This is particularly true for ankle invertor/evertor moments, as well as transverse moments at the knee and hip joints. Such large variances in the ankle invertor/evertor moments are due to the fact that these moments have small moment arms and cross the joint center. Previously, Eng and Winter [7] have observed that during the propulsive phase, seven out of nine participants exhibited an evertor moment while two exhibited an invertor moment. Apkarian et al. [8] also observed large individual differences in transversal knee and ankle joint moments. Another factor contributing to the large variances across all three planes is the large age span in the participant groups. The joint moment profiles presented here are the ensemble averages of all 30 participants who represented three age groups spanning from 20 to 86 years of age. The literature suggested that age is a significant influencing factor on both kinetic and kinematic variables during gait. Therefore, it is reasonable to expect joint moment variances increase as the age span increases. This may make our average joint moment curves difficult to interpret. In order to address this problem, we investigated the 3D joint moment differences resulting from age differences in the third part of our results section and this will be discussed further in this section.

As indicated in the second part of our results (joint moment comparison between global and local coordinates), significant coordinate system effect on 3D lower extremity joint moments was found in majority of percentage comparisons through controlled paired t-test. Generally, global coordinate system tended to underestimate adductor muscle moment in the frontal planes in all the joints but the ankle joint. Also, global coordinate system tended to underestimate sagittal plane moments. This phenomenon is particularly true for ankle frontal results and all three transverse results, whose patterns changed significantly (please refer to Fig. 1). Regardless of individual differences, hip external/internal rotator moment pattern expressed locally was similar to previous 3D kinetic analysis [7–8]. As to ankle adductor/abductor moment differences, the pattern under global coordinates was similar to Apkarian et al. [8] from three participants' data. Eng and Winter [7] observed similar pattern from nine participants' data in their study. Considering our large sample size, both patterns (different global and local patterns mentioned above) are possible.

Even though the averaged sagittal moment curves in both coordinate systems (Fig. 1) looked very close, significant differences were evident through paired t-test, especially for the entire hip sagittal moments. This result suggested the limitation of 2D joint moment analysis in sagittal planes. Therefore, 2D analysis with the underlying assumption of human planar motion, is not sufficient to investigate those activities (such as maintaining dynamic stability during slip and fall conditions) which will involve significant movement in 3D space.

In general, little aging effect was present in current 3D joint moment analysis of normal gait. However, several significant aging effect are present in all the three transverse planes and old participants generated greater internal rotator moments than their young counterparts during the middle of the stance phase. Considering the role of transverse moments in balance maintaining during normal walking, it can be suggested that the older adults may need more internal rotator muscle moments to bring their lower extremity back to middle progressive line.

In conclusion, current study confirmed our hypothesis that the coordinate system does have a significant effect on 3D joint moment expression. Additionally, aging effect is not obvious in current normal gait. However, considering the fact that normal walking is a effortless daily activity, it can be expected that aging effect will be pronounced in highly strength-demanding gait conditions (such as reactive reactions when people experience slippery surface).

Appendix A. Supplementary data

Supplementary data associated with this article can be found, in the online version, at doi:10.1016/j.gaitpost.2005. 06.011.