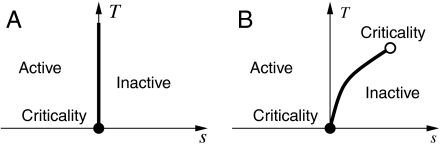
Fig. 1.

Space–time phase diagrams. (A) Generic space–time phase diagram for KCMs (11). There is a first-order phase boundary that occupies the s = 0 axis, separating an active fluid phase from an inactive “glass.” The critical point s = T = 0 is identified with a filled circle: No motion takes place in this state, and the approach to this point is characterized by scaling behavior and slow dynamical relaxation (22, 23). (B) Sketch of the space–time phase diagram for the softened FA model, under the assumption that the probability of violating constraints ϵ has an Arrhenius form, as described in the main text. The first-order phase boundary moves away from the s = 0 axis and ends in a new finite-temperature critical point, identified with an open circle. The scaling behavior in the vicinity of this point is analogous to the critical behavior near liquid–vapor transitions and is different from the scaling near s = T = 0.