Fronts, jumps and secondary introductions suggested as different invasion patterns in marine species, with an increase in spread rates over time

Abstract

Not all introduced (invasive) species in a region will spread from a single point of introduction. Long-distance dispersal or further introductions can obscure the pattern of spread, but the regional importance of such processes is difficult to gauge. These difficulties are further compounded when information on the multiple scale process of invasive species range expansion is reduced to one-dimensional estimates of spread (e.g. km yr⁻¹). We therefore compared the results of two different metrics of range expansion: maximum linear rate of spread and accumulation of occupied grid squares (50 × 50 km) over time. An analysis of records for 54 species of introduced marine macrophytes in the Mediterranean and northeast Atlantic revealed cases where the invasion process was probably missed (e.g. Atlantic Bonnemaisonia hamifera) and suggested cases of secondary introductions or erratic jump dispersal (Dasysiphonia sp. and Womersleyella setacea). A majority of species analysed showed evidence for an accumulation of invaded sites without a clear invasion front. Estimates of spread rate are increasing for more recent introductions. The increase is greater than can be accounted for by temporally varying search effort and implies a historical increase in vector efficiency and/or a decreased resistance of native communities to invasion.

1. Introduction

Geographical range expansion following a species' introduction is one of the most conspicuous stages of the invasion process. Introduced species are frequently considered to spread behind an invasion front. The front may be a continuous feature, with most suitable sites behind the front occupied, or the front may be a more diffuse feature with outlying colonies established by rarer long-distance dispersal events. Such variability in invasion pattern can be generated with different dispersal kernels, as shown when comparing the spread patterns produced under diffusion, leptokurtic and stratified diffusion models (Gilbert et al. 2004). A view of invasion patterns as the consequence of an often unknown dispersal kernel may, however, underestimate the importance of other processes at a regional scale. For example, multiple introductions, rather than dispersal from an invaded site, can result in a spread of occupied sites across a region. The distinction becomes important when formulating policy. If a species is appearing across a region by multiple introductions, a policy of ‘fire breaks’ or quarantines of the already occupied sites is likely to be ineffective in preventing further introductions.

Any regional summary of invasions will be hampered by the multiple scales involved in both introduction and spread processes. A single measure of spatial spread will always be an incomplete measure of the invasion process (Pyšek & Hulme 2005). We therefore propose that a contrast between different measures of range expansion will be more informative in summarizing the regional scale patterns. Two commonly used approaches are measurements of maximum range size and estimates of the area of occupation (e.g. Weber 1998). These measurements tend to emphasize different aspects of the spreading pattern occurring during an invasion. For example, rare long-distance events will have a greater influence on measures of maximum range extent than on area-based metrics of spread. Furthermore, statistical confidence in different measures of spread may be informative. Where a species is spreading behind a front, both the range extent and area of occupation metrics are likely to be estimated with a high degree of confidence. In comparison, multiple introductions may not result in a clear expansion of range margins even if there is increased local occupation of sites around each occupied area.

We tested the approach of using different measures of spread in a regional summary using data on invasion patterns for introduced macroalgae and a seagrass on European shores (Mediterranean Sea and northeast Atlantic Ocean). There are significant knowledge gaps concerning the means of introduction for macrophytes (Williams & Smith 2007) that may obscure the choice of an appropriate management strategy. There are likely to be a high number of potential arrival points to a region for species that may be associated with vectors including maritime traffic and transfers of stock in aquaculture. Furthermore, macrophytes have dispersal patterns that could obscure the means of range expansion. While reproductive stages are typically thought to have relatively short dispersal distances (less than 100 m, Dudgeon et al. 2001), the restricted dispersal of juvenile stages can be augmented by drifting clumps of adult seaweeds; potentially over long distances (Santelices 1990; Ingolfsson 1995; Thiel 2003). The adult drifting stage is more significant if algal fragments can regenerate and re-attach to a substratum (Rodriguez 1996; Collado-Vides 2001; Khou et al. 2007; Fonck et al. 2008). The dispersal of algae may also be enhanced over short and long ranges by a wide range of anthropogenic vectors offering hitchhiking opportunities for seaweeds (Hewitt et al. 2007).

The analyses presented in this study show how a comparison of different metrics of spread can be used to provide a regional summary of the invasion process. Regression models were fitted to an extensive database of geographical records. The approach provides a means to separate the classical pattern of invasion behind a front from one of multiple start points or a major role for long-distance vectors. Dates of initial introductions allow a comparison between spread patterns of old and recent introductions and an evaluation of contemporary changes in the mean rate of spread.

2. Material and methods

(a). Database

Following the definition of Williamson & Fitter (1996), we considered ‘introduced’ to mean a species that has been brought outside its native range via human activities and which is found in the wild, but not necessarily successfully established (as evidence needs to be gathered with recurring observations on the same site). Also, there is a discontinuity between the native area and the area of introduction (Boudouresque & Verlaque 2002), involving a vector of introduction. Consequently, natural range extension of native species indirectly caused by human activities (e.g. global warming) is not taken into account. Nevertheless, we included Lessepsian migrants, i.e. species that are native to the Red Sea and have been introduced into the Mediterranean Sea by range expansion through the Suez Canal (Por 1978).

We collated data about the 126 marine macrophytes (125 algae and one seagrass) considered as introduced in Europe including the Mediterranean Sea (Boudouresque & Verlaque 2002; Ribera-Siguan 2002; Wallentinus 2002; M. Verlaque unpublished data). Geographical data about non-native range were collected from various published and unpublished sources (journals and books, ‘grey’ literature, mainly reports and theses; personal data, including personal communications from other colleagues). For bibliographical reviews (e.g. Furnari et al. 2003), the original publications referred to were consulted to obtain the most reliable information on date and locality.

For each species, we included all records made under different names (i.e. synonyms or misidentifications). All observations were geo-referenced using a geographical information system. All homonyms and outdated names of locations in old literature were carefully checked. The date of observation was recorded as the year of observation if indicated. When the date could not be inferred from the manuscript, we chose the year of publication (or submission) as the date of observation. In some cases, ambiguity about the first observations (especially when no exact locality was given) led us to use subsequent observations as the start point.

As the grain size of the areal rate of spread estimates was based on 50 km grid squares, we discarded species that had a restricted distribution (all observations within a radius of 50 km). Furthermore, isolated introductions in the Macaronesian Islands (Canaries and Azores) were also excluded from analyses. This left a total of 54 species, eight of which were considered to have had separate primary introductions in both the Mediterranean and the Atlantic.

(b). Estimates of spread rate

Two approaches were used to define an empirical rate of spread for each species in the database. One of these (maximum rate of spread) was spatially explicit, involving a measurement of distance from the point of first observation. The second approach (accumulated area of spread) was based on the number of grid squares containing records for the target species.

The maximum rate of spread method involved taking the location of the first date of observation in each area (Atlantic or Mediterranean) as the start of the invasion process. All subsequent observations were considered as potential measures of the invasive front. For each of these observations, we measured the least cost distance from the origin of introduction using a GIS-based routine. This distance was defined as the shortest path between the two points, without crossing the coastline. The algorithm followed the coastline unless there was a shorter route across open water. The coastline itself was defined using a raster grid with a grid size of 0.01° in a WGS84 projection.

Linear regression was used to estimate the maximum rate of spread from a biplot of distances from the point of origin against the year of observation. Before fitting a regression line, the data were filtered so that only points representing an increase on the previously measured maximum distance were included. Using a linear regression estimate of range expansion, although widely deployed in invasive species studies, is undermined by the constraint that ranges cannot decrease. By constraining the independent variable, significant fits become more likely. To estimate how likely, a null model simulation was used. This consisted of a sequence of random numbers, transformed into points where a number was only included if it represented an increase on previous numbers. Regressions through repeated random sequences (n = 1000) provide an estimate of type I error. This suggested that a more appropriate α value for regression estimates of maximum rate of spread was 0.005.

The accumulated area of spread was based on the total number of grid cells containing at least one record for the target species. We used near-equal area grid cells of ca 50 × 50 km (Nogués-Bravo & Araújo 2006). The grid was superimposed on the coastline, resulting in 386 coastal cells in the Atlantic (not including the Baltic, with the most northerly occupied cell at 65° N on the Norwegian coast) and 520 coastal cells in the Mediterranean (all coastlines, but not including the Marmara or Black Seas).

The accumulation of occupied grid squares is not a spatially explicit estimate of spread, but models fitted to such data can still be informative. Among the many potential nonlinear functions available to fit to the data, we wished to make a specific contrast: is the pattern of occupation equivalent to the discovery of a pre-existing population or is there evidence for an increase in site occupation with time? This contrast is of particular relevance to groups such as macroalgae, which include some inconspicuous species for which there is some doubt about the date of introduction or the native range.

If a species is already present across a fixed number of sites but is no longer expanding in range, I, the accumulation of records over time can be described by the following equation, which describes an asymptotic increase to the point when all occupied sites have been discovered:

2.1

where I is the number of grid squares recorded as occupied by the species at time t, a is the (fixed) total number of sites occupied across the region, t is the time in years since the first record and b is a time-invariant search effort.

Equation (2.1) always produced a statistically significant fit to the data. To test the hypothesis that an increase in occupied sites was occurring, the model can be expanded to make the number of occupied sites vary as a function of time. With a fixed per grid square rate of colonization of new sites, c, the dynamics of occupied sites will follow a logistic function:

2.2

where, for t = 0:

In equation (2.2), the term a reflects the potential distribution following range expansion rather than the actual range as in equation (2.1). The other term, k, can be related to the size of the population when the invasion was first recorded. Although I₀ is consistent with equation (2.2), at time zero no observations have been made; so I₀ actually represents the sum of occupied squares when a time series starts. When t > 0, I₀ can be considered as the observed number of cells owing to the non-zero value for e^−bt. If k equals a − 1, then the introduced species is predicted to have been in one cell only when first recorded. Smaller values of k imply a larger number of introduction sites and/or some spatial spread before the species was first recorded as introduced. If k and c are equal to zero, equation (2.2) reverts to equation (2.1): there is no new spatial spread following the first record and the accumulation of sites reflects a discovery process rather than a colonization process. As equation (2.1) is nested in equation (2.2), it is possible to test using analysis of variance whether the addition of the parameters k and c significantly improves the fit (Crawley 2002). The statistical comparison of fitted equations was therefore used as a test that each species was spreading to an increasing number of grid squares.

Fits for the equations were estimated using the nonlinear curve fitting tools in Sigmaplot. The software uses an iterative procedure to minimize the residual error using the Marquardt–Levenberg algorithm. Constraints were applied in the fitting process to keep all parameter estimates positive and for range parameters (a and k) to be less than the total number of grid cells in each region (NE Atlantic and Mediterranean).

3. Results

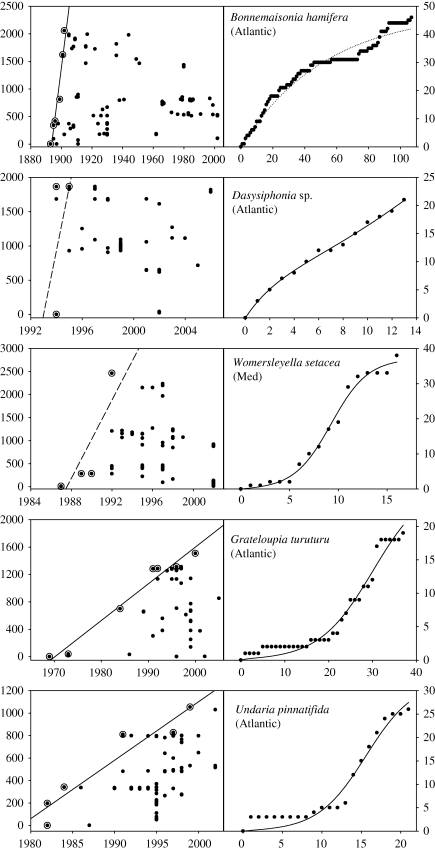
For the 62 combinations of species and region, 17 had a significant fit for a linear rate of spread and there were 49 examples showing evidence for a range expansion in the number of occupied grid squares (examples in figure 1). For the limited number of species introduced to both regions, there were no significant differences in the estimated spread rate between the northeast Atlantic and Mediterranean (paired t-test for colonization rate: t = 0.48, n = 6).

Figure 1.

Range expansion histories for five invasive seaweeds, chosen to illustrate the species with one or both of the potential spread regressions being significant. Plots on the left of each species pair show the distance (in km) from the point of origin. Solid lines represent significant linear regression estimates for the maximum rate of spread. Only points representing new maximum distances from the origin (circled points) are used in estimating the linear regression. Dashed lines indicate that the linear regression fit was not significant at the 0.005 level. Plots on the right of each species pair represent the accumulation of occupied grid squares. Solid curves represent an invasive spread: a significant fit for equation (2.2) (see text). A dotted line indicates that the accumulation of occupied sites can be described with a discovery model only (equation (2.1)). Horizontal axes are in years AD (left of each pair) or number of years since the first record (right of each pair).

Cross-tabulation of the significant and non-significant results for colonization rate and maximum spread rate resolves the species into four groups (table 1; full results and authorities for all species in the electronic supplementary material).

Table 1.

Spread measurements of macrophyte invasions in Europe (labelled by the region of invasion as A, Atlantic; M, Mediterranean Sea), with the two methods: maximum spread (km yr⁻¹) and accumulated area of spread (colonization rate, c). Spread coefficients are only shown where the models gave a significant fit. This separates the species list into four groups depending on the combinations of significance across the two metrics.

species	area	year of first observation	number of records	number of grid squares occupied	maximum spread (km yr−1)	colonization constant, c
species group A
Apoglossum gregarium	M	1992	10	9	—	—
Antithamnionella ternifolia	M	1926	4	2	—	—
Botryocladia madagascariensis	M	1991	16	9	—	—
Chondria curvilineata	M	1981	5	4	—	—
Chondria pygmaea	M	1991	12	9	—	—
Cladophora herpestica	M	1948	9	7	—	—
Gracilaria arcuata	M	1931	6	3	—	—
Laurencia caduciramulosa	M	1991	9	5	—	—
Leathesia verruculiformis	A	1994	6	3	—	—
Sarconema filiforme	M	1945	11	5	—	—
Scytosiphon dotyi	A	1987	7	5	—	—
species group B
Bonnemaisonia hamifera	A	1893	128	46	218	—
Ceramium strobiliforme	M	1990	8	3	132	—
species group C
Acanthophora nayadiformis	M	1813	55	33	—	0.0134
Aglaothamnion feldmanniae	A	1965	14	10	—	0.1039
Aglaothamnion halliae	A	1960	21	9	—	0.071
Antithamnion amphigeneum	M	1989	13	9	—	1
Antithamnionella boergesenii	M	1937	4	4	—	0.0292
Antithamnionella elegans	A	1961	13	5	—	0.0896
Antithamnionella spirographidis	M	1913	27	19	—	0.0362
Antithamnionella sublittoralis	M	1980	5	4	—	0.1212
Asparagopsis armata	A	1923	249	46	—	0.2394
Asparagopsis armata	M	1923	128	61	—	0.0394
Bonnemaisonia hamifera	M	1967	25	18	—	0.0576
Caulerpa mexicana	M	1941	14	8	—	0.01
Caulerpa racemosa var. cylindracea	M	1990	98	37	—	0.5002
Caulerpa racemosa var. lamourouxii	M	1951	12	8	—	0.0202
Caulerpa racemosa var. turbinat-uvifera	M	1926	13	10	—	0.0427
Caulerpa scalpelliformis	M	1929	14	6	—	0.0106
Caulerpa taxifolia	M	1984	87	14	—	0.7638
Codium taylorii	M	1958	7	6	—	0.105
Colpomenia peregrina	M	1918	18	13	—	0.0405
Dasya baillouviana	A	1950	22	10	—	0.035
Dasysiphonia sp.	A	1994	64	21	—	0.1099
Fucus evanescens	A	1894	31	16	—	0.0229
Ganonema farinosa	M	1808	9	8	—	0.0102
Goniotrichiopsis sublittoralis	M	1989	4	3	—	0.0953
Gracilaria vermiculophylla	A	1997	58	13	—	0.3803
Grateloupia subpectinata	A	1947	10	6	—	0.0461
Lomentaria hakodatensis	A	1984	9	6	—	0.0893
Neosiphonia harveyi	M	1958	31	18	—	0.0889
Pleonosporium caribaeum	A	1973	9	6	—	0.0744
Polysiphonia paniculata	M	1967	7	7	—	0.7402
Solieria dura	M	1944	3	3	—	0.0802
Stypopodium schimperi	A	1974	17	13	—	0.2418
Ulva pertusa	A	1993	8	5	—	0.1067
Womersleyella setacea	M	1987	74	38	—	0.53
species group D
Acrothamnion preissii	M	1969	49	18	37	0.0635
Anotrichium okamurae?	A	1922	24	11	17	0.035
Antithamnionella elegans	M	1882	72	39	27	0.0513
Antithamnionella spirographidis	A	1927	33	23	21	0.1011
Antithamnionella ternifolia	A	1906	84	44	27	0.0327
Asparagopsis taxiformis (invasive form)	M	1993	15	11	126	0.151
Codium fragile subsp. fragile	A	1845	215	87	22	0.0556
Codium fragile subsp. fragile	M	1950	43	19	76	0.1807
Colpomenia peregrina	A	1905	119	65	38	0.0519
Grateloupia turuturu	A	1969	55	19	54	0.163
Halophila stipulacea	M	1894	31	27	14	0.0477
Hypnea spinella	M	1928	19	16	68	0.1006
Neosiphonia harveyi	A	1832	56	39	13	0.0307
Sargassum muticum	A	1972	552	100	69	0.3269
Undaria pinnatifida	A	1982	97	26	52	0.3273

The first group contained 11 species (table 1, group A) that did not appear to be expanding the number of occupied cells and did not have linear rates of range expansion. As the discovery model (equation (2.1)) was a significant fit to the data for this group but the colonization model did not provide a significant improvement in fit, the increase in occupied grid cells seems to represent an accumulation in records of an already established species rather than a spreading process. The clearest feature identifying this group was, however, a low number of records relative to other cases, where rate of spread models were significant fits to the data.

Two species (table 1, group B) appeared to have a linear range of expansion without evidence for a spread into new grid squares. For Ceramium strobiliforme, only a few grid squares are occupied. Given such a low level of current occupancy, the lack of a significant improvement in fit for a colonization model is not surprising. By contrast, the situation with Bonnemaisonia hamifera in the Atlantic (figure 1) does not seem to reflect a lack of data and the accumulation of sites is well described by a rise to an asymptote (equation (2.1)). This pattern is what would be expected for a species where the accumulation of sites reflects the discovery of the pre-existing, fixed, range.

A group of 34 species (table 1, group C) appeared to be expanding in the number of occupied squares, but with a rate of change in the maximum range size that was not linear. This group had fewer records per species than the group where both colonization and maximum rate models were significant (mean of 7.2 points per species where maximum rate of spread was significant when compared with a mean of 4.1 records where the linear regression was not significant, t = 4.1, p < 0.05, d.f. 17, equal variances not assumed, means significantly different). Membership of this group may therefore reflect a lower power for the linear regression. A conventional estimate of statistical power in the regression model is difficult to estimate owing to the constraints on the rate of spread measurement. As an alternative, the relationship between the number of points and the log-transformed regression p-value was examined. This relationship had a negative slope, reflecting more confidence in rejecting the null hypothesis with larger samples. Residuals for algae in group A were not significantly different from zero, implying that these algae may have been found to have significant expansion rates if more data were available. The mean of residuals for group C was, however, positive—indicating that the non-significant p-values of this group fall outside the average pattern and are therefore likely to represent appropriate rejection of the null hypothesis rather than low sample size (electronic supplementary material). This suggests that the pattern is not entirely artefactual. For the two species with the highest number of records, Dasysiphonia sp. and Womersleyella setacea (figure 1), the argument for the lack of data is also less convincing, as the plots show a very rapid spread to the range limits. This may be evidence for multiple introductions or particularly rapid secondary dispersal once introduced. For example, the distances of records from the first record for W. setacea appear to fall into three groups, potentially representing different introductions and expansion around these foci.

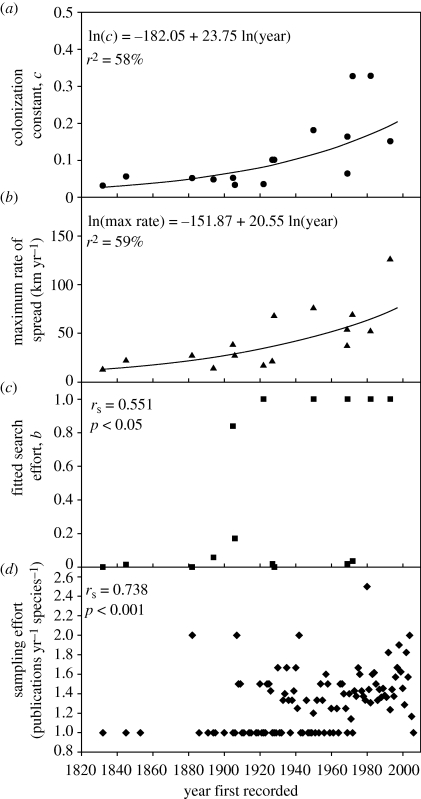
Finally, group D (table 1, group D; n = 15) comprised species that had both a significant linear rate of spread and evidence for a range expansion in colonized grid squares. The average maximum rate of spread for this group is 44 km yr⁻¹. When spread rate estimates are plotted against the year of first introduction, there is a trend for more recent introductions to have higher rates than the earlier introduced species (figure 2a,b). The relationships are best described by an exponential function.

Figure 2.

Variation in spread metric values and indices of collection effort as a function of the year of first observation. (a) Colonization rate, c, (b) maximum spread (in km yr⁻¹), (c) fitted search effort (constant b), (d) sampling effort (or number of publications in which observations of the species are mentioned, per year of observation). Only points for taxa where both the maximum rate of spread and colonization rate were defined by significant regression relationships are shown (species group D).

The apparent increase in spread rates may be an artefact of an increase in search effort over time. The increased effort is evident in significant increases in estimates of the fitted parameter b (equation (2.2); figure 2c) and in the trend for the number of publications containing species records (figure 2d). Such increases in search effort do not, however, seem sufficient to explain the exponential rise in the rate of spread. The effect of a time-varying search effort was investigated in simulations using the average parameter values (a = 200, c = 0.11, I₀ = 12) and the average span of invasion (78 years). When the search effort (b) rose linearly from 0 to 1 over the 78 years in a simulated introduction process, the value of c estimated by fitting a curve to the data increased by 12 per cent from the non-varying search rate baseline of 0.11. With a sigmoid increase from low to high search effort (0–1 over 78 years), the estimated value of c increased by 44 per cent compared with the average value used to simulate the spread. While this demonstrates that a large increase over time in b can bias estimates of c under a model where b is fitted as a constant, the size of the bias is less than the observed variation. The observed increase in c over an equivalent period of time was greater than 165 per cent (figure 2a): exceeding what might be expected under the strongest likely artefacts from a time-varying search effort (example shown in the electronic supplementary material).

4. Discussion

Using different measures of spread rate identifies species that are increasing in the number of occupied areas without a clear invasion front. There are slightly more species with no defined invasion front than there are species spreading evenly from a fairly localized introduction. The former group (group C) is either repeatedly introduced to Europe in a manner that confounds the spread pattern or the existing vectors act to obscure any progressive range expansion. It is perhaps not surprising that algae can lack invasion fronts while expanding the number of sites occupied. The vectors of hull fouling and aquaculture act with many points of entry to the region and over a range of scales within Europe. The analyses suggest that while the appropriate management response to restrict range spread for some species (group D) may be localized eradication and quarantine, for a second group (C) these approaches will be ineffectual.

Significance testing for two estimates of rate of spread divides species into four groups. Species belonging to the first group (A), with no significant fit for both accumulation of area or maximum rate of spread, may be placed there through low statistical power. Many of the ‘A’ group are inconspicuous, difficult to detect (e.g. small size for Leathesia verruculiformis) or hard to discriminate from native species. The spread of these species may well have been missed, so that the only signal left to be retrieved is an accumulation of records for an established species.

Some species may lack sufficient data to establish an accumulation of area and therefore fall into the second group (B). This group includes Ceramium strobiliforme that was present in only a few grid cells in the Mediterranean Sea. By contrast, the remaining species of this group, the red alga Bonnemaisonia hamifera (Atlantic), has been recorded numerous times in separate locations. This species follows a ‘discovery only’ pattern despite a significant estimate for the maximum rate of spread. The significant maximum rate of spread in this case may be an artefact of a chance separation between two early records and the subsequent search around these locations (the English Channel area, where the species was first recorded, and the northern North Sea). The clustering of search effort around these foci is evident in two clouds of points expanding in the upper and lower half of the spread plot for B. hamifera (figure 1). The absence of a statistically significant estimate for c in the presence of a high number of records means that we can reject the hypothesis that B. hamifera was a new introduction with two sites of origin in the late nineteenth century. A more reasonable alternative is that the original invasion has been missed and all that can be retrieved from the historical record is the accumulation of sites in a now widely established species. Interestingly, the later introduction of B. hamifera to the Mediterranean has not been missed and there is a clear accumulation of occupied grid squares.

The third group of species classified by cross-tabulation of significance tests are those where the introduced species are accumulating occupied grid squares at an increasing rate but there is no linear range expansion (group C). These species may have been introduced multiple number of times to new locations since the first record or their association with vectors may lead to particularly irregular ‘jump’ dispersal. This is especially true for Dasysiphonia sp. and W. setacea, two recently introduced species for which a great number of records are available. For these species, erratic long distance transports or secondary introductions seem to have occurred in the early stages of the invasion process. An alternative point of introduction for Dasysiphonia sp. may have occurred 10 years before the first year record used in our analysis (Sjøtun et al. 2008).

In some circumstances, such as with an Allee effect, the maximum rate of spread can be an increasing function of time (Hastings et al. 2005). This may have been the case for one species in group C, Caulerpa taxifolia. This assessment is based on a lower adjusted r² value in an exponential model when compared with a linear model and a highly significant fit for the nonlinear model. The pattern was for an increasing maximum rate of spread over time. It is not clear whether this pattern reflects an ecological trait (many of which are shared with other species). The pattern of an increasing spread may be an artefact of low sample size, as it is dependent on a single early point (graph in the electronic supplementary material).

The remaining 14 species of group D, for which both rate of accumulation and maximum rate of spread are significant, are those where secondary introductions do not obscure the invasion front and jump dispersal, if it occurs, is both reasonably consistent and has a scale less than the total coastline length considered.

There is good evidence that the rate of spread over time is increasing despite some increase in detection effort. This is unlikely to be a simple result of changes in the level of effort, as effort is estimated directly when fitting the colonization rate curves. To have a collection effort artefact, the later estimates of c need to be made in a context where initial effort is low, followed by a rapid increase. At the same time, earlier introduced species would have to have constant or decreasing collection effort. These situations seem unlikely, in addition to being insufficient to produce artefacts on c of the same size as the observed change over time. An alternative to a change in effort would be a lag owing to time for a species to increase to abundance high enough to be recorded in a grid square. To cause an apparent increase in c over time, however, the time to grow to recordable size has to be related to the date of introduction. Species that arrived at the start of the time period would need to become conspicuous more rapidly than later arriving species so that later arriving species could become widespread before being noticed and hence have an apparently high spread rate. While this possibility cannot be ruled out, a ‘time to accumulate’ bias towards earlier arriving species seems unlikely. The alternative is that rates of spread have increased in more recent years. Increasing rates may be due to greater economic activity and the associated rise in the number of trips and distances moved by anthropogenic vectors. Other possible causes for the acceleration in spread rates also include ecological processes that facilitate successful colonization by the introduced species: a progressive deterioration of natural communities or the facilitation of novel colonizers by the previously introduced species (Grosholz 2005). If estimates of the maximum rate of spread showed an increase while estimates of c from the accumulated rate of spread did not increase at the same rate, one might conclude that the efficiency of vectors was improving over time while the local resistance to introduced species was being maintained. A comparison of the fitted regressions suggests the opposite. Estimates of c are increasing relatively more rapidly than the maximum rate of spread estimates, perhaps indicating that local conditions are changing more rapidly than the efficiency of vectors.

Apparent increases in invasion frequency or rate have been previously shown to be potential artefacts of the means of estimation. For example, Wilson et al. (2007) used two points in time and therefore constrained earlier introductions to a narrower range of potential rates than later introductions. This constraint does not apply to the current study. The most well-known example of an increase in invasion rate over time is the acceleration in the number of invasive species in San Francisco Bay (Cohen & Carlton 1998). This is a different measure to the one based on rates of spread as it is based on the total number of introduced species. Further analyses for curves of the accumulation of invasive species number over time have shown that an exponential increase is not necessarily evidence of a rise over time in arrival or establishment; constant arrival rates can also produce exponentially increasing numbers of introduced species to a site (Costello & Solow 2003; Wonham & Pachepsky 2006). The acceleration in rates presented in this paper is fundamentally different from the accumulation of species at a site. The rates are independent estimates of the range dynamics of individual species. This makes the conclusions more robust than is the case for methods that are based on the interpretation of a single curve.

In conclusion, a dual model-fitting approach allows novel information to be extracted from historical datasets of invasion. This has identified species where the invasion probably occurred before the first record in the literature and species with secondary introductions and/or unpredictable jump dispersal. Over half of the examined invasions lack a clear invasion front, meaning that algae are arriving or being transported unpredictably across the region. For macroalgae, the classic invasion model of introduction and spread from a particular location may not be adequate to describe the majority of species' range expansion dynamics. This may well be the case for other groups, particularly those associated with vectors that have a high number of potential arrival locations. The models described are straightforward to fit and are applicable to any spatially referenced invasion time series. This statistical model-fitting facilitates comparison between different invasive species and has potential applications for managing invasions and policy-making. Parameter values from the fitted models suggest that there has been an increase in species invasion rates over time, potentially indicating a decreasing resistance of native communities to invasion, as well as an increased effectiveness of transport vectors.