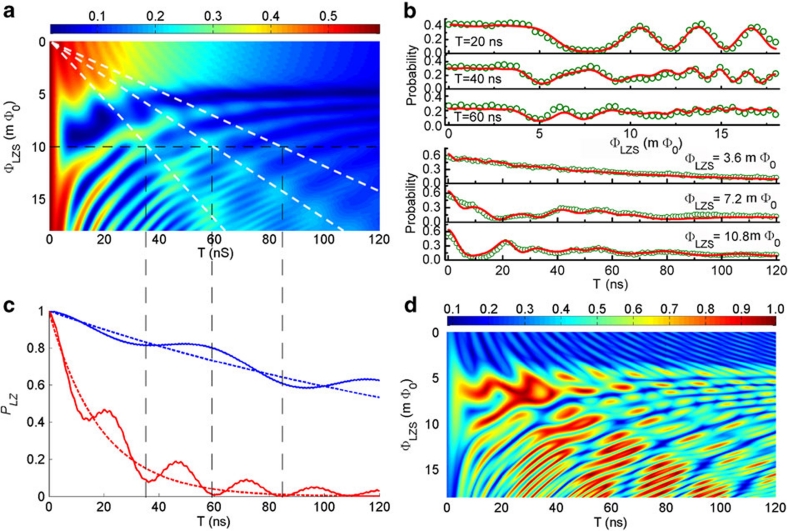
Figure 3. Numerically simulated LZS interference pattern and control of a generalized W state in a phase qubit coupled to two TLSs.

(a) The numerically simulated population of |1 after the triangular flux pulse is plotted as a function of the width and amplitude of the triangular flux bias. The horizontal dotted line indicates the location of Φ_LZS=10 mΦ₀ and the vertical dotted lines indicate the locations of 'hot spots' at Φ_LZS=10 mΦ₀. The oblique dotted lines are lines of constant sweeping rate. 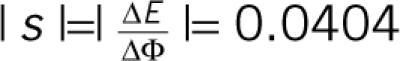The parameters used are determined experimentally: ω_01,dc/2π=16.747 GHz, GHz/mΦ₀, ω_TLS1/2π=16.590 GHz, ω_TLS2/2π=16.510 GHz, Δ₁/2π=10 MHz, Δ₂/2π=32 MHz, 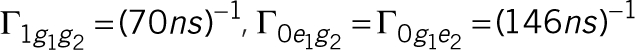γ^(deph)=(45 ns)⁻¹. (b) The upper panel shows the dependence of population of |1 on Φ_LZS at T=20, 40 and 60 ns, respectively. The lower panel shows the dependence of population of |1 on T at Φ_LZS=3.6, 7.2 and 10.8 mΦ₀, respectively. The circles represent the experimental data and the lines from the theory. (c) LZ transition probabilities of M₁ (blue line) and M₂ (red line) at Φ_LZS=10 mΦ₀ as a function of pulse width. They are quite different from the asymptotic LZ transition probabilities (blue dotted line and red dotted line). (d) The resulting w as a function of T and Φ_LZS.