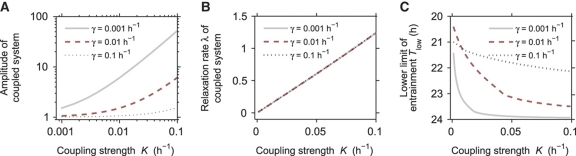
Figure 5.

Coupling constrains the entrainment range of oscillators. (A) Coupling increases the amplitude of the synchronized, coupled system. Given are numerically calculated results of two coupled Poincaré oscillators (see Equation 3). Coupling is quantified by the coupling strength parameter K. The amplitude increase upon coupling is more pronounced, if the individual oscillators are weak, i.e., for small relaxation rates γ. (B) Coupling makes oscillators more rigid by increasing the relaxation rate λ of the coupled system. Shown are numerically calculated results of two coupled Poincaré oscillators. Note that the effect of the coupling strength on the rigidity of the coupled system is not dependent on the amplitude relaxation rates γ of the individual oscillators. As coupling slightly affects the period of the coupled system, the relaxation rate λ was normalized by multiplying with the coupling-dependent period. (C) Coupling makes an oscillatory system harder to entrain. Two coupled Poincaré oscillators were entrained by adding a periodic square-wave forcing of period T, alternating with equal duration and a forcing between 0 and 0.1 (term F in Equation 3). The lower limit of entrainment T_Low of the coupled system was calculated for different coupling strengths K and different amplitude relaxation rates γ of individual oscillators. As the coupling affects the intrinsic (non-forced) period itself, the lower limit of entrainment was normalized with respect to a constant intrinsic period of 24 h to visualize the effects on the entrainment range that are entirely due to the effects on the rigidity and amplitude of the coupled system.