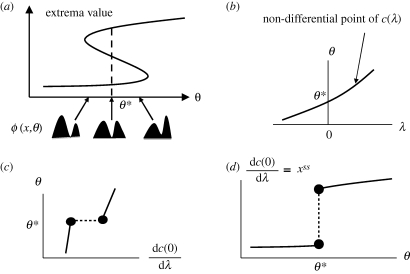
Figure 2.

(a) The solid line represents the extrema of the ϕ(x), which corresponds to the stable and unstable fixed points of the nonlinear differential equation model. θ is a bifurcation parameter. When θ = θ*, the two wells of ϕ(x) have equal height. (b) For each value of θ, the double-well ϕ(x) yields a non-analytical point λ*(θ). This line crosses the λ = 0 when θ = θ*. (c) θ as a function of dc(0)/dλ. (d) dc(0)/dλ is in fact the position of the lower minima of ϕ(x), which is the mean concentration of X in the system.