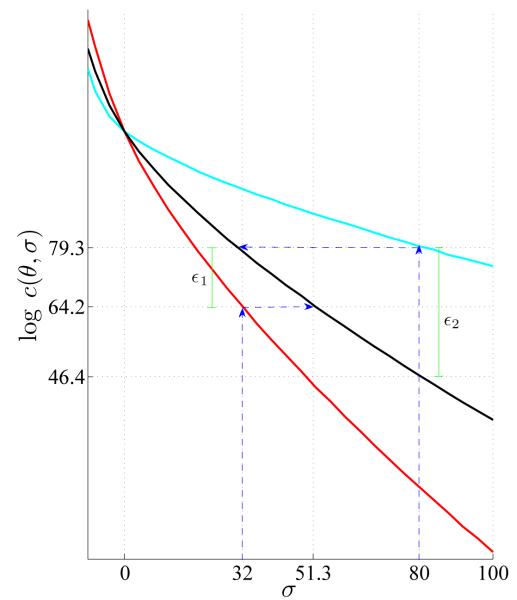
Figure .6.

Normalizing constants on a log scale for antagonistic (cyan), independence (black) and synergistic (red) models for a range of σ values, as obtained by an adjusted Monte Carlo method using equation 14 and Appendix A. The effect of choosing an appropriate σ_ind to minimize the adjustment by the estimate of $E\left[e^{{\stackrel{‒}{\sigma }}_{\mathrm{epi}}\left(\mathbf{x}\right)-{\stackrel{‒}{\sigma }}_{\mathrm{ind}}\left(\mathbf{x}\right)}\right]$ in equation 13 can be seen by comparing the difference between the constant obtained by choosing the optimal σ_ind and choosing σ_epi = σ_ind. For example, for the constant desired with σ_syn = 32, the corresponding optimal value for σ_ind(x) is 51.3. If σ_ind = 32 were used, the estimate of the expected value $E\left[e^{{\stackrel{‒}{\sigma }}_{\mathrm{epi}}\left(\mathbf{x}\right)-{\stackrel{‒}{\sigma }}_{\mathrm{ind}}\left(\mathbf{x}\right)}\right]$ in the approximation of c(θ, σ) would have to adjust for a large discrepancy (approximately ∊₁ on the log scale). Similarly, for the constant desired with σ_ant = 80, the optimum is σ_ind = 32, and if σ_ind = 80 were used, the adjustment by the expectation in the estimate would be approximately ∊₂ (log scale). Choosing the optimal value of σ_ind minimizes the effect of the adjustment.