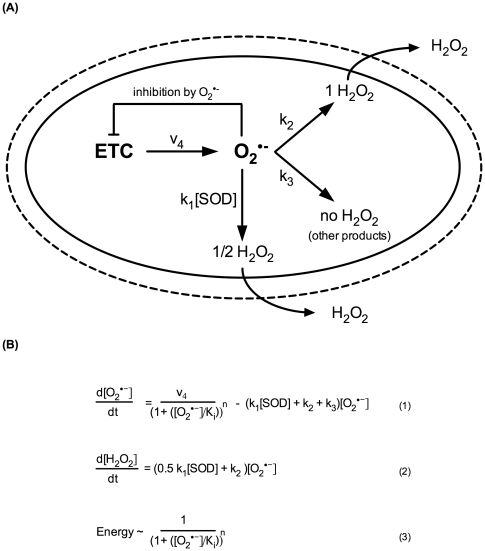
Figure 7. Minimal model for mitochondrial superoxide production and consumption and hydrogen peroxide production.

Model based on that of Gardner et al. [37], [50], modified for the mitochondrial compartment. A) Three classes of superoxide consumption reactions with different hydrogen peroxide yields are considered. Each class represents a large number of actual reactions yielding either 1, 1/2 or no hydrogen peroxide per superoxide consumed. The model includes a simple negative feedback mechanism of superoxide on its own production rate. B) Equations for production and consumption of superoxide and hydrogen peroxide according to the model. Hydrogen peroxide, but not superoxide, can exit the mitochondria, and therefore, only equation (1) is steady state (d[O₂•⁻]/dt = 0). The actual rate of superoxide production is v₄/(1+[O₂•⁻]/K_i)ⁿ, where the denominator models the feedback inhibition of superoxide negatively modulating its own rate of production. The strength of this feedback is controlled by the exponent n, while the threshold at which feedback becomes significant is controlled by the inhibition constant K_i. For n = 0, there is no feedback inhibition at any level of superoxide while, for n = 1, superoxide production is ½ maximal if superoxide levels are equal to K_i.