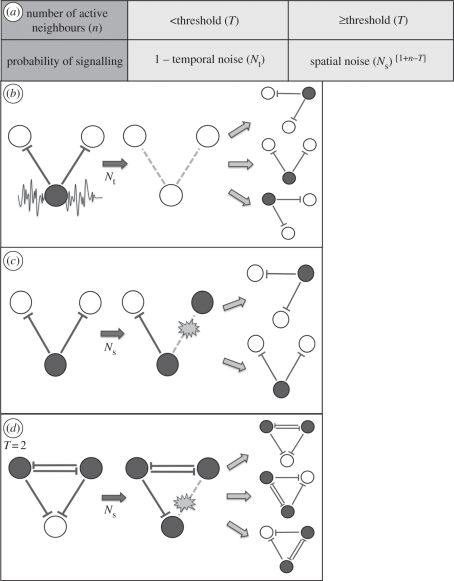
Figure 3.

An enhanced model of lateral inhibition incorporating signalling noise and different inhibitory thresholds. (a) The rule table determines the probability that a selected cell in an asynchronous cellular automaton will actively express an inhibitory signal. The threshold (T) is the minimum number of active signalling cells (n) required to inhibit an inactive cell. Temporal noise (N_t) is the probability that a cell will stop signalling even without an inhibitory signal at the required threshold. Spatial noise(N_s) is the probability that an inactive cell will signal even when it is in contact with the threshold number of active signalling cells. This probability reduces as the number of active neighbours increases over the threshold. (b) A schematic of pattern shifting owing to temporal signalling noise. A cell's inhibitory signal (dark grey) oscillates over time. Its signal effectively ceases such that at subsequent time steps neighbouring cells that were previously inhibited may become active. (c) A schematic of pattern shifting owing to spatial signalling noise. A signal ‘connection’ is broken and an inactive cell (white) no longer receives an inhibitory signal and becomes active. At subsequent time steps when the connection is re-established, the cells compete and a stable configuration is re-established. (d) A representation of spatial noise in which a minimum threshold of two active cells is required to inhibit a third cell. As in (c) the pattern may shift as a result of the signal noise.