Skip to main content
. Author manuscript; available in PMC: 2012 Sep 1.
Published in final edited form as: J Struct Biol. 2011 May 17;175(3):288–299. doi: 10.1016/j.jsb.2011.05.011

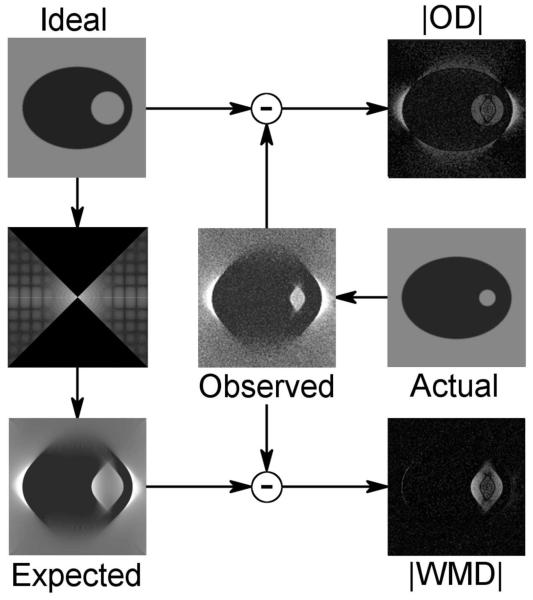
Figure 1.

Figure 1

Comparison of wedge-masked and ordinary differences. Ideal: The ideal or true object. If not known, the ideal may be well approximated by weighted averaging or other techniques. Actual: An individual, sample object containing a smaller hole than the ideal. Individual objects are typically not accessible to direct observation. Instead, we have access only to Observed: a tomographic reconstruction of the actual object suffering from noise and missing data artifacts. |OD|: Magnitude of the ordinary difference between the ideal and observed objects. In addition to the real variation in hole diameter, ODs are heavily influenced by missing data artifacts, resulting in a bright halo. If parameters defining the missing data are known or have been estimated, they can be applied to compute an Expected object from the ideal. In this particular example, we Fourier transform the ideal, zero out a vertically oriented 90° missing wedge, and then invert the masked Fourier transform. |WMD|: Magnitude of the “wedge-masked” difference between the expected and observed objects. WMDs preserves true variation while largely suppressing missing data artifacts.