|
At time
t = 0 |
1. Obtain estimates for model parameters (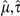 ), initial number of susceptibles, ), initial number of susceptibles, 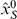 , and a prior distribution for random variable , and a prior distribution for random variable 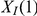 . . |
2. Use Eqs. (7)–(8) to determine the dynamic health policy 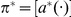 . . |
3. Find the optimal number of susceptibles to immunize, 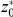 , using Eq. (10). , using Eq. (10). |
4. Increment time to 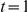 . . |
5. Update the number of susceptibles at time 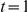 : : 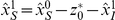 . . |
While
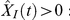
|
1. Given the availability of vaccine, use Eq. (8) or Eq. (12) to find the optimal number of susceptibles to immunize, 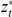 , and the optimal transmission-reducing intervention, , and the optimal transmission-reducing intervention, 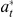 . . |
2. At the end of period  , use the real-time data , use the real-time data 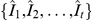 to update the model parameters (using for instance, maximum likelihood estimation) and the dynamic health policy to update the model parameters (using for instance, maximum likelihood estimation) and the dynamic health policy 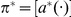 , using Eqs. (7)–(8). , using Eqs. (7)–(8). |
3. Increment time: 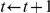 . . |
4. Use Table 1 to update the epidemic state at time  . . |
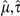 ), initial number of susceptibles,
), initial number of susceptibles, 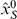 , and a prior distribution for random variable
, and a prior distribution for random variable 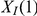 .
.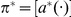 .
.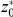 , using Eq. (10).
, using Eq. (10).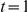 .
.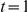 :
: 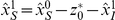 .
.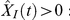
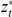 , and the optimal transmission-reducing intervention,
, and the optimal transmission-reducing intervention, 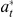 .
. , use the real-time data
, use the real-time data 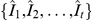 to update the model parameters (using for instance, maximum likelihood estimation) and the dynamic health policy
to update the model parameters (using for instance, maximum likelihood estimation) and the dynamic health policy 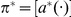 , using Eqs. (7)–(8).
, using Eqs. (7)–(8).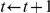 .
. .
.