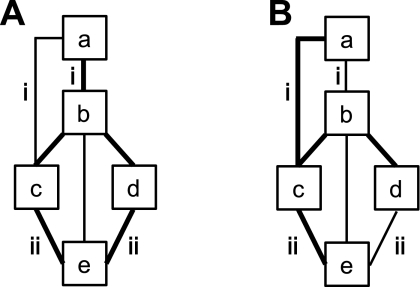
Fig. 28.

Examples of satisfied and violated constraints in estimating the functional hierarchy of cortical regions based on fcMRI. A functional hierarchy is estimated based on the assumption that regions closer in a hierarchy have stronger correlation. A: 5 cortical regions are arranged in a 4-level hierarchy whose functional connectivity strengths satisfy both hierarchical and lateral constraints. B: identical arrangement of 5 cortical regions in a 4-level hierarchy with different functional connectivity strengths that violate both hierarchical and lateral constraints. Thick lines correspond to strong correlations. Thin lines correspond to weak correlations. i: regions a and c are farther apart than regions a and b. In the example in A, correlation of regions a and c is weaker than correlation of regions a and b, so a hierarchical constraint is satisfied. In the example in B, correlation of regions a and c is stronger than correlation of regions a and b, so a hierarchical constraint is violated. ii: regions c and d are on the same hierarchical level. In the example in A, correlation of regions c and e is approximately the same as the correlation of regions d and e, so a lateral constraint is satisfied. In the example in B, correlation of regions c and e is stronger than the correlation of regions d and e, so a lateral constraint is violated. In the context of hierarchy estimation in this article, we consider 2 correlations within 0.2 of each other to be approximately the same when assessing lateral constraints. Given the pairwise correlations of a set of seed regions and a known number of levels in the hierarchy, we can seek the best hierarchical arrangement of the seed regions with the following local optimization procedure: 1) randomly arrange the seed regions into a hierarchy, 2) consider swapping a pair of seed regions or shifting a single seed region to a different hierarchical level without changing the number of levels in the hierarchy such that the proportion of violated constraints is maximally decreased, 3) terminate when no further improvement in the proportion of violated constraints can be achieved, and 4) repeat the preceding steps 20 times, picking the solution with the least proportion of violated constraints. The best solution obtained using this optimization procedure is (in practice) the same as a brute-force search over all possible hierarchical arrangements of the seed regions. We note that we are generally unable to infer the number of levels in the functional hierarchy, since the number of possible constraints can be drastically different for hierarchies with a different number of levels, and so the proportion of violated or satisfied constraints is not comparable across hierarchies with different levels. In practice, however, the solution space is robust; for example, the best solution for a 5-level hierarchy typically differs from the best solution for a 4-level hierarchy by the collapsing of regions from 2 adjacent levels into 1 level. Uncovering the true hierarchical structure in the macaque visual hierarchy based on anatomical connectivity has also proved to be problematic (Hilgetag et al. 1996).