Abstract
The influence of isotopically enriched magnesium on the creatine kinase catalyzed phosphorylation of adenosine diphosphate is examined in two independent series of experiments where adenosine triphosphate (ATP) concentrations were determined by a luciferase-linked luminescence end-point assay or a real-time spectrophotometric assay. No increase was observed between the rates of ATP production with natural Mg, 24Mg, and 25Mg, nor was any significant magnetic field effect observed in magnetic fields from 3 to 1,000 mT. Our results are in conflict with those reported by Buchachenko et al. [J Am Chem Soc 130:12868–12869 (2008)], and they challenge these authors’ general claims that a large (two- to threefold) magnetic isotope effect is “universally observable” for ATP-producing enzymes [Her Russ Acad Sci 80:22–28 (2010)] and that “enzymatic phosphorylation is an ion-radical, electron-spin-selective process” [Proc Natl Acad Sci USA 101:10793–10796 (2005)].
Keywords: radical pair, spin biochemistry
Reports of magnetic effects on biological processes abound in the biochemical literature, but few of them are sufficiently large and well-defined to have received independent confirmation (1). Furthermore, an explanation of the mechanism is often lacking.
One possible reason for an influence of magnetic fields on biochemical reactions is the radical pair mechanism (2–6). In a series of papers by Buchachenko and Kuznetsov (BK) together with various co-workers (7–22), a radical pair mechanism has been proposed for enzymatic phosphorylation of adenosine diphosphate (ADP) to adenosine triphosphate (ATP). This mechanism is influenced by the presence of a magnesium isotope with a nuclear spin. 25Mg (natural abundance 10%) has I = 5/2, whereas the other two natural isotopes, 24Mg and 26Mg (natural abundances 79% and 11%, respectively), have I = 0. BK propose that Mg2+ participates in the phosphorylation reaction of ADP through phosphate-to-Mg2+ electron transfer, forming a radical-ion pair (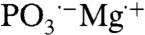 ). The singlet and triplet spin configurations of the pair contribute differently to ATP synthesis, with the triplet state being the more efficient, because recombination from the triplet state is forbidden by Pauli exclusion, whereas this product-depleting step is allowed for the singlet state. 25Mg enhances the product formation by inducing a higher population in the triplet state via hyperfine coupling. Spin rephasing of the unpaired electron in
). The singlet and triplet spin configurations of the pair contribute differently to ATP synthesis, with the triplet state being the more efficient, because recombination from the triplet state is forbidden by Pauli exclusion, whereas this product-depleting step is allowed for the singlet state. 25Mg enhances the product formation by inducing a higher population in the triplet state via hyperfine coupling. Spin rephasing of the unpaired electron in 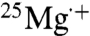 , due to the hyperfine field of the 25Mg nucleus, converts the initial singlet state of the radical pair to the triplet state.
, due to the hyperfine field of the 25Mg nucleus, converts the initial singlet state of the radical pair to the triplet state.
BK show large increases (two- to threefold) in the rate of ATP production from ADP with a variety of enzymes, when they use isotopically enriched 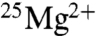 . These enzymes are mitochondrial ATP synthase (8, 11, 16), phosphoglycerate kinase (9, 13), pyruvate kinase (12), and creatine kinase (9, 12, 15, 20). The effects increased linearly with the amount of 25Mg in the isotopic mixture (13). Furthermore, the activity of an enzyme that contains 25Mg was strongly enhanced in the presence of the magnetic field. For instance, with creatine kinase the 2.3-fold increase seen for
. These enzymes are mitochondrial ATP synthase (8, 11, 16), phosphoglycerate kinase (9, 13), pyruvate kinase (12), and creatine kinase (9, 12, 15, 20). The effects increased linearly with the amount of 25Mg in the isotopic mixture (13). Furthermore, the activity of an enzyme that contains 25Mg was strongly enhanced in the presence of the magnetic field. For instance, with creatine kinase the 2.3-fold increase seen for 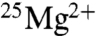 without a field rose to a fourfold increase at 80 mT (12, 15). According to BK (17), the radical-pair-based mechanism and resulting isotope effect operate only at elevated intracellular Mg concentrations (10.5–24 mM), whereas at normal physiological concentrations (< 1 mM) the classical nucleophilic mechanism (23, 24) is at work. No effect was found for the reverse reaction (ATP → ADP) (12).
without a field rose to a fourfold increase at 80 mT (12, 15). According to BK (17), the radical-pair-based mechanism and resulting isotope effect operate only at elevated intracellular Mg concentrations (10.5–24 mM), whereas at normal physiological concentrations (< 1 mM) the classical nucleophilic mechanism (23, 24) is at work. No effect was found for the reverse reaction (ATP → ADP) (12).
The production and hydrolysis of ATP are critical for metabolic processes in living organisms; humans produce and consume quantities comparable to their body mass on a daily basis (25). Creatine kinase, phosphoglycerate kinase, pyruvate kinase, and ATP synthase are key enzymes directly responsible for production and regulation of ATP (26). The ability to modify their reactions via magnetic isotopes or magnetic fields could have significant therapeutic implications. Of particular interest is the treatment of ischemic conditions such as stroke and heart disease, where reduced oxygen supply to the affected tissue leads to a severe reduction in the cell’s ability to produce ATP. Indeed, Buchachenko and co-workers have been involved in developing a delivery system on the basis of a porphyrin adduct of C60 (21), and positive results have been reported in preclinical trials on mammalian heart muscle (22, 27). The 10% natural abundance of 25Mg and the requirement for very high Mg2+ concentrations should limit the influence of the magnetic isotope effect (MIE) and associated magnetic field effects under normal physiological conditions, but BK assert that effects could be significant because of natural local fluctuations in Mg ion concentrations (17). This assertion implies that there could be ramifications for health and safety if ATP production can really be influenced by modest magnetic fields.
Since the publication of their first results in 2004, the only independent evidence of BK’s isotope effect on ATP production is indirect; it was reported in two conference proceedings by Koltover et al. that the proliferation of Escherichia coli cells is increased by around 20% in the presence of 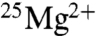 (28, 29). Buchachenko has recently summarized their results in the sentence, “It is universally observable that enzymes with the magnetic-isotopic nucleus of magnesium produce two to three times more ATP than the same enzymes with nonmagnetic nuclei under the same conditions” (7). Here was a large, general effect of potentially wide significance for which there was a clear explanatory mechanism on the basis of a well-established magnetochemical phenomenon (radical pair effect). Thus, we were eager to test the striking assertion. Here we report the results of two series of experiments on creatine kinase (CK; EC 2.7.3.2) that were conducted independently in the authors’ laboratories [Trinity College Dublin (TCD) and University of Essex (UE)].
(28, 29). Buchachenko has recently summarized their results in the sentence, “It is universally observable that enzymes with the magnetic-isotopic nucleus of magnesium produce two to three times more ATP than the same enzymes with nonmagnetic nuclei under the same conditions” (7). Here was a large, general effect of potentially wide significance for which there was a clear explanatory mechanism on the basis of a well-established magnetochemical phenomenon (radical pair effect). Thus, we were eager to test the striking assertion. Here we report the results of two series of experiments on creatine kinase (CK; EC 2.7.3.2) that were conducted independently in the authors’ laboratories [Trinity College Dublin (TCD) and University of Essex (UE)].
Results
Fixed End-Point Assay (TCD).
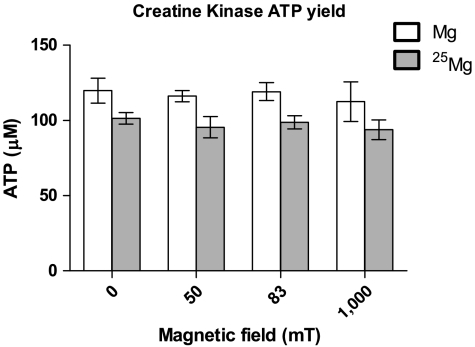
We examined the effect of the 25Mg isotope on the ATP yield of MMI CK (type I of the muscle-muscle dimer) after 30 min in 0- (ambient), 50-, 83-, and 1,000-mT magnetic fields (Fig. 1). Comparing the ATP yields for the natural abundance Mg (∗Mg) and 97.7% enriched 25Mg (25Mg) samples from five independent experiments, we found that the large 25Mg isotope-induced increases reported by BK (> 2-fold at 0 mT, > 3-fold at approximately 50 mT, and > 4-fold at 80 mT) were entirely absent; if there was an isotope effect at work, its result is to decrease ATP production. The overall small decrease (approximately 15%) due to 25Mg was found to be statistically significant (p < 0.001) by a two-way analysis of variance (ANOVA-2), although Bonferroni posttests of the individual isotope-induced changes in each field did not indicate that any were significant on their own.
Fig. 1.
The average ATP yield produced by MM creatine kinase after 30 min in the presence of 20 mM 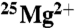 or natural Mg2+ (*Mg) is shown. Bars represent the standard error on the mean.
or natural Mg2+ (*Mg) is shown. Bars represent the standard error on the mean. 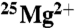 and
and 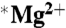 samples were placed in a number of different uniform magnetic fields over this time period. These were the ambient field (0 mT), 50, 83, and 1,000 mT. No increase in ATP production due to the
samples were placed in a number of different uniform magnetic fields over this time period. These were the ambient field (0 mT), 50, 83, and 1,000 mT. No increase in ATP production due to the 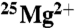 isotope was observed, nor was a magnetic field effect observed. There was a small (15%) but significant (ANOVA-2; p < 0.001; N = 5) decrease in the
isotope was observed, nor was a magnetic field effect observed. There was a small (15%) but significant (ANOVA-2; p < 0.001; N = 5) decrease in the 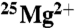 samples versus the
samples versus the 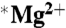 samples.
samples.
Two additional experiments were carried out where no magnetic field was applied, but substrate concentrations and the reaction temperature were identical to those described by BK (0.16 mM ADP, 0.16 mM phosphocreatine, T = 30 °C) (15). We found that the average ATP yield with 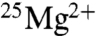 was 0.97 (± 0.09) of the
was 0.97 (± 0.09) of the 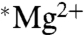 yield, so again the > 2-fold increase reported by BK was not observed.
yield, so again the > 2-fold increase reported by BK was not observed.
Real-Time Assay (UE).
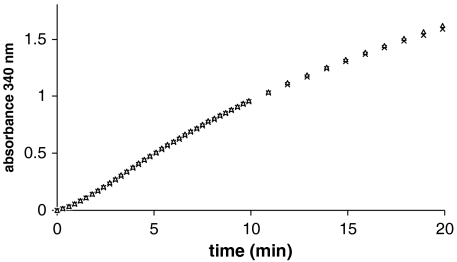
Fig. 2 compares the absorbance time courses obtained in the presence of either 24Mg or 25Mg. On the basis of the reports by BK (12, 15), we would have expected the slope of the curve to double on replacing 24Mg by 25Mg. It is evident there was no discernible isotope effect (N = 5). Any change in reaction rate must be less than 2%. We were concerned that a confounding factor might have been the influence of heavy metals, particularly Pb, which is a known inhibitor of CK, that copurify to different extents in the two Mg isotopes, although both were > 99.9% pure. In order to test whether Pb contamination may have perturbed our results, we added Pb to the assay medium, initially at 120 nM, which was a concentration equivalent to the contaminating Pb in the isotopes, and also at a fiftyfold excess. No effect could be seen at 120 nM, and at 6 μM Pb the slope decreased by only 10%.
Fig. 2.
Illustrative time courses for CK activity obtained in the presence of either pure 24Mg (△) or 25Mg (×) isotopes (20 mM). No increase in ATP production due to the 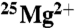 isotope was observed.
isotope was observed.
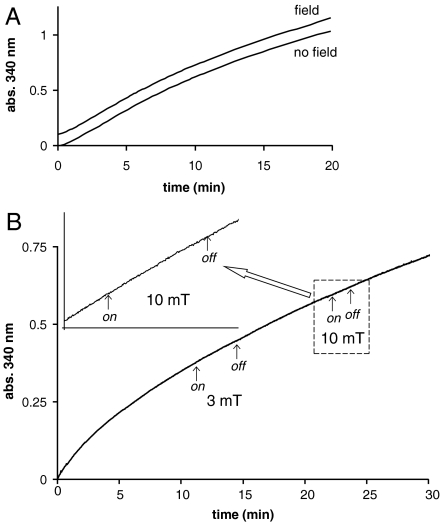
The influence of applying magnetic fields of up to 10 mT was investigated in real time by using a dual coil spectrophotometer system (see Materials and Methods and Figs. S1 and S2). In Fig. 3A, we show the results of setting up identical CK assays in both the sample and the reference cuvettes, with a field of 10 mT applied to the sample. Within the experimental accuracy of our measurements, the traces are completely identical (one is displaced upward by 0.1 for clarity). According to both refs. 12 and 15, we should have expected a 10% increase of slope in the 10-mT magnetic field. To be sure that we were not missing some small effect, we repeated this experiment in another way, illustrated in Fig. 3B. A field was first applied and then switched off during the course of a single assay. The effects of switching on and off a 3-mT and a 10-mT field are shown. The magnified trace in the Inset again demonstrates that there is no change of slope (and hence rate of ATP production). These experiments were repeated more than 10 times, and no significant effect of the field was ever observed. The differences in rates (determined from linear fits to the data points) immediately before and immediately after the application of a 10-mT field were used in a paired t test to assess the statistical significance of any differences observed. In the experiments illustrated in Fig. 3, there was no significant difference with and without the field (p = 0.3, N = 20). In fact, any difference we did observe (although not significant) was to decrease the rate rather than increase it as expected from the work of BK.
Fig. 3.
The effects of magnetic fields on the CK assay containing the 25Mg isotope. (A) The rate of formation of NADPH in the CK assay using 25Mg. Two identical reaction mixtures are shown; one is subject to no applied external magnetic field (S coil), and the other has an applied external magnetic field of 10 mT (F coil). The time course for the sample within the field is displaced upward by 0.1 for clarity. (B) The effects of different magnetic fields (3 and 10 mT) applied in “on” and “off” cycles during the assay. An expanded region of the time course where the system was subjected to a 10-mT field is shown.
Discussion
Our experiments have revealed no magnetic isotope effect on the production of ATP by MM creatine kinase. The results of the fixed end-point study (TCD) show no increase in ATP due to 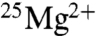 and in fact show a small decrease (15%) in ATP production. This decrease is statistically significant, but it remains unclear whether it could be a real isotope effect or if it is simply due to small differences in the reaction solutions. Inhibitory trace metals like Pb are a possible cause, but Pb was below the detection limits for inductively coupled plasma mass spectrometry in both 25Mg and ∗Mg reaction solutions, implying that concentrations were less than 2.4 μM, which is less than the 6 μM shown by UE to decrease rates by 10%. Further investigation would be required to establish if there is any small but real negative isotope effect. Even if a genuine isotope effect was at work here, it may be a mass isotope effect rather than a magnetic effect. The key point is that the > 2-fold increase reported by BK (12, 15) did not occur for creatine kinase.
and in fact show a small decrease (15%) in ATP production. This decrease is statistically significant, but it remains unclear whether it could be a real isotope effect or if it is simply due to small differences in the reaction solutions. Inhibitory trace metals like Pb are a possible cause, but Pb was below the detection limits for inductively coupled plasma mass spectrometry in both 25Mg and ∗Mg reaction solutions, implying that concentrations were less than 2.4 μM, which is less than the 6 μM shown by UE to decrease rates by 10%. Further investigation would be required to establish if there is any small but real negative isotope effect. Even if a genuine isotope effect was at work here, it may be a mass isotope effect rather than a magnetic effect. The key point is that the > 2-fold increase reported by BK (12, 15) did not occur for creatine kinase.
The extent of the incompatibility of these results with the claims of BK can be assessed by formulating the hypothesis that the effect of using 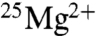 is to double ATP production in ambient magnetic fields. When we calculate the natural log (ln) of the ratio of ATP yields with
is to double ATP production in ambient magnetic fields. When we calculate the natural log (ln) of the ratio of ATP yields with 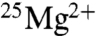 versus those with
versus those with 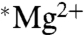 , we get a mean of -0.16 for the five experiments. The standard deviation (SD) was 0.094, and the standard error of the mean (SEM;
, we get a mean of -0.16 for the five experiments. The standard deviation (SD) was 0.094, and the standard error of the mean (SEM; 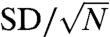 ) was 0.042. No effect corresponds to 0 (ln 1 = 0). Our mean of -0.16 is 3.8 SEMs from 0, which indicates a small but significant decrease for
) was 0.042. No effect corresponds to 0 (ln 1 = 0). Our mean of -0.16 is 3.8 SEMs from 0, which indicates a small but significant decrease for 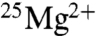 . Doubling the rate of ATP production corresponds to a value of 0.69 (ln 2 = 0.69), which is over 20 SEMs from our mean. The probability of such a difference occurring by chance is vanishingly small (p < 10-7). The probabilities that our data represent the changes reported by BK for applied magnetic fields are similarly miniscule.
. Doubling the rate of ATP production corresponds to a value of 0.69 (ln 2 = 0.69), which is over 20 SEMs from our mean. The probability of such a difference occurring by chance is vanishingly small (p < 10-7). The probabilities that our data represent the changes reported by BK for applied magnetic fields are similarly miniscule.
The real-time analysis (UE) using a direct spectrophotometric assay optimized to monitor the activity of human heart muscle CK is equally conclusive; this enzyme is unaffected by the nature of the Mg isotope that is used as a cofactor. We have carefully assessed the potential confounding effects of Pb and have concluded that the levels of contamination that may be introduced by the Mg isotopes are insignificant, particularly in the presence of millimolar glutathione that is part of the assay system.
Furthermore, we have again failed to find any magnetic field effect as reported by BK. In the real-time assay, we were limited to fields of 10 mT. However, the data of Buchachenko et al. (12, 15) would indicate that with this field the activity of CK in the presence of 25Mg should increase by approximately 10%. Certainly, we would expect to have seen any such increase in the slope of the assay time course. We have paid close attention to the possibility that temperature variations on applying a field (caused by heating effects in the coils) may obscure small isotope-dependent activity changes and have eliminated any such temperature variations as a source of error by careful control of sample and reference cuvettes (< 0.1 °C difference). Any magnetic field effects on the activity of heart muscle CK must be much smaller than reported by Buchachenko. Statistical analysis of our data indicates that a 1% increase in activity would have shown as significant (p < 0.05). In the fixed end-point assays, we can apply much larger fields, up to 1,000 mT, but here too there is no effect, whereas BK claim that we should see approximately two-, three-, and fourfold increases at the fields tested (0, 50, and 83 mT, respectively) (12, 15). One of the predictions made in ref. 18 is for almost no MIE at 1,000 mT, but, because we have found no effect across all fields tested, this agreement with our findings is hardly significant.
The reaction mechanism at the active site of CK is generally regarded to be common to different CK isozymes (MM, MB [muscle-brain], BB [brain-brain], and Mt [mitochondrial]) (30–32). Furthermore, the magnesium isotope effect reported by BK occurs in a number of different kinases including pyruvate kinase, phosphoglycerate kinase, and ATP synthase, so it would be surprising if the effect occurred in one species variant of CK but not in either variant of MM CK that we tested. The CK isozyme used by BK is extracted from the venom of a snake Vipera Xanthia (sic) (15, 20), but details regarding the isozyme and type are not reported. The purified protein is an active monomer (20) not a dimer like CK MM, MB, and BB (30–32). BK also report an isotope effect in mitochondrial creatine kinase (CK Mt) which has an octameric structure (33). It is conceivable that these nondimeric variations of CK operate via a different reaction mechanism to the more commonly studied dimeric forms, but this idea was not envisaged by BK.
It must be noted that, besides the source of our enzyme, our experimental conditions also differ in some respects from those reported in ref. 15 (see Materials and Methods and Table S1). Nevertheless, none of these differences would be expected to significantly alter the reaction mechanism that gives rise to the MIE. There will undoubtedly be differences in overall reaction rates, but these should affect both isotopically enriched and natural magnesium equally so that any MIE remains unaltered. Such is expected to be the case for the differences in temperature across the studies and any differences in enzyme concentration. BK do not specify their enzyme concentration, which cannot be determined from the information they provide, because this information includes only the mass of enzyme added without giving the volume of the solution (15).
The most significant difference between the reaction conditions is probably the substrate concentrations (ADP and phosphocreatine). Different CK species will have different activities and substrate Km values which determine the appropriate substrate concentrations, so it is expected that these would differ when varieties of the enzyme are used. Because the Km values for V. xanthia are not known to us and the rationale behind their choice of concentrations is not explained (15), it is difficult to compare their values with ours. We have used 10× the Km values in order to ensure that the enzyme is close to full occupancy with its substrates (CK follows Michaelis-Menten kinetics) and to minimize the isotope-insensitive reverse reaction (ATP + creatine → ADP + P-creatine). For the TCD study, the final ATP concentrations measured (approximately 120 μM—see Fig. 1) were around 1.5% of the ADP concentrations (8 mM), which indicates that the reaction was still at the stage (< 10% of ADP) where the forward reaction is dominant and the reverse reaction is negligible. In other words, the levels of ATP required to fuel a significant reverse reaction rate had not yet accumulated, so that ATP production greatly exceeded its breakdown throughout the period of the experiment. In the case of UE (see Fig. 2) the rates of ATP production were observed during the early stage when the forward reaction is dominant (linear rise) and during the later stage when both forward and reverse reactions contribute significantly (asymptotic rise toward plateau). Thus, for both studies the lack of an MIE was not due to the occurrence of equilibrium and the negation of the MIE-sensitive forward reaction by the insensitive reverse reaction. Once this effect is ruled out, it seems unlikely that such changes in substrate concentrations would do anything other than alter the overall kinetics.
To verify this conclusion, we carried out two additional fixed end-point experiments comparing ATP yields from CK with either 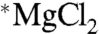 or
or 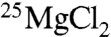 . This time no magnetic fields were applied, but substrate concentrations and the reaction temperature were the same as those described by BK (15); i.e., all conditions but the enzyme type and concentration were the same. Again no twofold increase was observed, and the only change was a small decrease of 3%. Thus, we must conclude that differences in substrate concentrations or temperature were not responsible for the absence of the MIE and that the only plausible cause for this absence appears to be the difference in enzyme source or isozyme, but this explanation is quite unlikely for the reasons discussed above.
. This time no magnetic fields were applied, but substrate concentrations and the reaction temperature were the same as those described by BK (15); i.e., all conditions but the enzyme type and concentration were the same. Again no twofold increase was observed, and the only change was a small decrease of 3%. Thus, we must conclude that differences in substrate concentrations or temperature were not responsible for the absence of the MIE and that the only plausible cause for this absence appears to be the difference in enzyme source or isozyme, but this explanation is quite unlikely for the reasons discussed above.
Finally, we turn our attention to BK’s proposed mechanism. It is well established that the reactivity of a radical pair with certain properties (e.g., required separation distance and lifetime) will be affected by a magnetic field, whether it be externally applied or due to hyperfine coupling of magnetic nuclei like 25Mg (I = 5/2) (2–4). What is more controversial in BK’s mechanism is whether the radical pair forms in the first place, particularly because one of the radicals, Mg·+, is very unstable in an aqueous environment. The other key issue is whether the properties of the radical pair and the conditions of the enzyme active site (EAS) would actually allow the radical pair to exist at the separations and for the periods of time necessary for significant magnetic effects.
Since 2010 (17), BK have accepted that the classical nucleophilic mechanism of ADP phosphorylation operates under normal physiological conditions. Here ADP3- in the EAS is coordinated to an Mg2+ ion forming an MgADP- complex whose oxy-anion can perform an inline nucleophilic attack on the phosphorus of the nearby phosphate-donating substrate (e.g., phosphocreatine or inorganic PO4) leading to formation of ATP (23). BK propose that, when the Mg2+ concentration rises to 50–100 times the normal intracellular concentration, then two additional Mg2+ ions enter the EAS, with one binding the PO4-donating substrate and the other binding n H2O molecules (17). In the EAS where water is “squeezed” out (17), the hydration number n of the free Mg2+ is low (≤ 6), and this low hydration number enables its conversion to an Mg·+ radical ion via electron transfer from the MgADP- complex (17) (Table S2, reaction 3). The result is a radical pair, Mg·+(H2O)n-MgADP·(H2O)m (m is the hydration number of MgADP·/-). From here the ADP· radical can attack the PO4-donating substrate to form ATP or recombine to form Mg2+(H2O)n-MgADP-(H2O)m.
In ref. 17, BK performed a series of density functional theory (DFT) energy calculations to justify the electron transfer from MgADP- to Mg2+ and the formation of the Mg·+ MgADP· radical pair. They calculated the energies for the reaction (ΔE) as a function of the Mg2+ hydration number, n for free Mg2+ and m for pyrophosphate (MgHP2O7 or CH3P2O7), and found that the reaction is energetically favorable for n ≤ 6 with MgHP2O7 and n ≤ 3 with CH3P2O7. m was found to have little effect on ΔE. They used the ADP analogue, pyrophosphate, rather than ADP itself on the grounds that it would not significantly alter the reaction energy calculated, while reducing the computational load. We repeated a few of the calculations using HP2O7 and the full ADP molecule (see Table S2), which were in fairly good agreement with their results, particularly with regard to the stability of the Mg·+ ion versus that of Mg2+, when the hydration number is low. Here the calculated ΔE’s were identical to BK’s. These results were also supported by experimental studies of Mg+/2+ hydration (34) in pure Mg ion-water clusters under ultrahigh vacuum. Low Mg hydration numbers in the EAS are also supported by some experimental evidence (24, 35).
However, these DFT studies are of limited relevance to the actual reaction taking place. The simulated reaction occurs in a vacuum, so the complex network of interactions between reactants and EAS residues is entirely ignored. Furthermore, the calculations provide only energies of the isolated reactants and products at equilibrium, so the kinetics, lifetimes, and separation distances of the radicals remain critical open questions. They also do not deal with the issue of whether two additional Mg2+ ions would actually enter the EAS at high Mg2+ concentrations and whether these ions would take up positions appropriate for electron transfer from MgADP- to occur. We are unaware of any evidence in the literature showing more than one Mg ion in the EAS of creatine kinase. Mg2+ has a strong affinity for phosphate (36). BK suggest that one of the additional Mg2+ ions binds the phosphate of phosphocreatine while the other ion remains free. It seems more likely that this ion would coordinate to MgADP- or to both MgADP- and phosphocreatine, thus stabilizing Mg2+ versus Mg+ and reducing the probability of electron transfer. Indeed in another ADP-phosphorylating enzyme, cAMP-dependent protein kinase, where there are two Mg ions in the EAS, an X-ray crystallographic study reported that both were bound to the ADP phosphates in the 2+ oxidation state (37). With coordinate bonds from ADP phosphates to Mg ions being in the region of 0.2–0.3 nm (24, 35), coordination with MgADP- would also likely force the radical pair separation distance below 1 nm, which is generally considered the distance below which the exchange interaction dominates so that magnetic isotope and field effects on spin become negligible (2, 3).
Conclusion
Working with two common varieties of MM creatine kinase (rabbit muscle and human heart), we have found no evidence for an increase in ATP production when 25Mg is used instead of naturally abundant Mg or 24Mg, nor have we found any magnetic field effect. Although our sources of the enzyme differed from the snake venom for which the CK isotope effect was originally documented, these differences are most likely irrelevant to the general reaction mechanism of the enzyme and, hence, to the presence or absence of a magnetic isotope effect. Although the mechanism proposed by BK is not implausible, there remain major issues which must be addressed before it could be considered convincing. Our experimental data, on the other hand, indicate that BK’s radical pair mechanism does not operate in MMI creatine kinase, and, thus, it can be assumed that only the conventional inline nucleophilic mechanism operates here.
Our data therefore refute the claim made by Buchachenko and Kuznetsov that a large 25Mg magnetic isotope effect is a universally observable property of ATP-producing enzymes. At best, if the MIE and its underlying radical-ion-based mechanism are accepted, then these must exhibit a profound and unusual sensitivity to the chosen isozyme/enzyme source, which has never been reported previously.
Materials and Methods
Preparation of Mg2+ Salts.
For the TCD work, isotopically enriched magnesium oxide (97.7% 25MgO) was purchased from Isoflex, and natural *MgO was used as the reference. 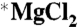 and
and 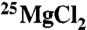 were prepared by reaction of MgO with concentrated molecular biology grade HCl (Sigma-Aldrich). The HCl was evaporated off, and the resulting MgCl2 salt was washed with ultrapure water. The isotopic composition of the
were prepared by reaction of MgO with concentrated molecular biology grade HCl (Sigma-Aldrich). The HCl was evaporated off, and the resulting MgCl2 salt was washed with ultrapure water. The isotopic composition of the 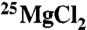 solutions was checked by ICP-mass spectrometry (MS) and found to be (1.5% 24Mg, 97.7% 25Mg, 0.8% 26Mg). After determination of the total magnesium, the concentrations in each sample were equalized by dilution.
solutions was checked by ICP-mass spectrometry (MS) and found to be (1.5% 24Mg, 97.7% 25Mg, 0.8% 26Mg). After determination of the total magnesium, the concentrations in each sample were equalized by dilution.
The oxide forms of the 24Mg and 25Mg isotopes were obtained from CK Gas Products for the UE work. The purity of the Mg isotopes was ascertained by CK Gas Products by ICP-MS and was found to be > 99% for both isotopes in two separately purchased batches. Stock salt solutions of the Mg isotopes were prepared by dissolving their oxides in a minimum of HNO3. This method reduced colloid formation. The solutions were then made up to 0.5 M and filtered through 2-μm filters.
Creatine Kinase Assay.
Creatine kinase (EC 2.7.3.2) MM type I from rabbit muscle was used by TCD. MM type I from human heart muscle was used by UE. All were purchased from Sigma-Aldrich. The luciferase-based ATP determination kit used by TCD (0.5 mM d-luciferin, 1.25 μg/mL firefly luciferase, 25 mM Tricine buffer, pH 7.8, 5 mM MgSO4, 100 μM EDTA, and 1 mM DTT) was purchased from Invitrogen. All other chemicals were purchased from Sigma-Aldrich. For the fixed end-point assay carried out by TCD, the CK reaction occurred at room temperature (22 ± 0.5 °C) in a Tris·HCl and potassium phosphate buffered solution (pH 6.35) containing 280 pM MMI creatine kinase, 20 mM 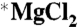 or
or 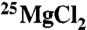 , 8 mM ADP, 50 mM phosphocreatine, and 0.1 mg/mL bovine serum albumin (enzyme stabilizer). All trace metals in our reaction solutions were found to be below 0.5 μg/mL by ICP-MS, except Fe which was present at 14.6 and 9.7 μg/mL in the ∗Mg and 25Mg solutions, respectively. The enzyme was added last to start the reaction, and directly after this step the solutions were placed in a uniform magnetic field. The reaction was terminated after 30 min by heating the solutions simultaneously and rapidly (τ = 16 s) to 90 ± 2 °C in a water bath for 10 min as a means to denature the CK enzyme while leaving the heat stable ATP intact. The samples were then stored at -80 °C for 1–5 d before a luciferase-linked assay kit was used to determine the ATP concentrations.
, 8 mM ADP, 50 mM phosphocreatine, and 0.1 mg/mL bovine serum albumin (enzyme stabilizer). All trace metals in our reaction solutions were found to be below 0.5 μg/mL by ICP-MS, except Fe which was present at 14.6 and 9.7 μg/mL in the ∗Mg and 25Mg solutions, respectively. The enzyme was added last to start the reaction, and directly after this step the solutions were placed in a uniform magnetic field. The reaction was terminated after 30 min by heating the solutions simultaneously and rapidly (τ = 16 s) to 90 ± 2 °C in a water bath for 10 min as a means to denature the CK enzyme while leaving the heat stable ATP intact. The samples were then stored at -80 °C for 1–5 d before a luciferase-linked assay kit was used to determine the ATP concentrations.
The assay operates on the basis that luciferase converts its substrate, luciferin, into oxyluciferin in the presence of ATP and O2 (38). A by-product of this reaction is light, which is produced in proportion to the ATP concentration. Thus, the ATP concentration of each sample was determined by adding 95 μL of the complete luciferase reaction solution (minus ATP) to 5 μL of the sample in triplicate. The resulting luminescence was measured on a Lumoskan Ascent microplate luminometer (Thermo Scientific). Luminescence intensities for a series of known ATP concentrations were also recorded to produce a standard curve, which allows extrapolation of ATP concentrations from the intensities of the samples. Before samples were subjected to the luciferase assay, they were sonicated (10 min) and vortex-mixed (5 s) to undo inhomogeneities caused by heating, freezing, and thawing. All samples were then equally diluted (1∶30 to 1∶50) to keep intensities within range of the ATP standard curve. All reported ATP yields are calculated to represent the original, undiluted concentration.
The real-time ATP measurements made by UE used a creatine kinase assay kit purchased from BioAssay Systems. The assay is based on enzyme-coupled reactions in which creatine phosphate and ADP are converted to creatine and ATP, catalyzed by CK. The ATP generated is used to phosphorylate glucose, catalyzed by hexokinase, to generate glucose-6-phosphate (G6P), which is then oxidized by nicotinamide adenine dinucleotide phosphate (NADP) in the presence of G6P-dehydrogenase (see Scheme 1). The NADPH produced can be monitored optically at 340 nm, and its concentration is equal to the creatine converted in the sample. This method provides a real-time determination of the reaction rate. The assay buffer was MES (20 mM, pH 6.2). The substrate solution comprises AMP, ADP, creatine phosphate, glucose, the reduced form of glutathione (5 mM), and NADP. The substrate solution is usually supplied with magnesium acetate present; however, we requested that this component of the solution be omitted because we wanted to make known additions of the Mg isotopes. The auxiliary enzymes are hexokinase and G6P-dehydrogenase. The presence of glutathione is to activate the CK. Glutathione can also act as a very good chelator of heavy metal atoms by their binding to the sulfhydryl groups.
Scheme 1.
Optimization of the Creatine Kinase Assay.
In the coupled enzyme system used by UE, it is intended that the rate of NADPH production should be a reliable measure of the rate of the reaction catalyzed by the CK present—i.e., that the CK enzyme should limit the overall rate. Once this condition is arranged, then it is possible to use the system to study those factors of interest that influence this rate—namely, the isotope of the Mg2+ used as a cofactor and the applied magnetic field. In order to ensure that the CK is limiting, we undertook a number of experiments adding excess auxiliary enzymes (hexokinase and G6P dehydrogenase) to ensure that conditions had been found where these were not influencing the rate (Fig. S1A). Increasing concentrations of CK were then added to the assay system to ensure that this enzyme was controlling the measured rate of NADPH production. Similarly, Mg2+ was titrated into the assay to achieve the optimum concentration. Increasing [Mg2+] increased the reaction rate up to approximately 20 mM (optimum), but at higher concentrations it inhibited the reaction (Fig. S1B).
Reagent concentrations and other key reaction conditions used for our experiments and those of BK are summarized in Table S1.
Magnetic Fields.
Permanent magnets were used by TCD. These were Halbach cylinders (39), which produced uniform magnetic fields of 50, 83, or 1,000 mT in a bore of at least 3 cm. Two 0.5-mL polystyrene sample tubes containing 100 μL of the complete reaction solution were placed at the center of the magnets. Permanent magnets have the advantage that there is no flow of electric current and, thus, no potential heating of the sample. One sample contained 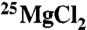 and the other
and the other 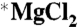 . For the ambient field condition (“0 mT”), the two samples were positioned far away from the magnets where the maximum static field was 60 μT (Earth’s field) and the maximum ac field was 3 μT. All magnetic fields were measured by using a Hall probe gaussmeter (Lakeshore). The temperatures in the vicinity of each sample were measured (TC-08; Pico Technology) before the experiment to ensure that they were equal to within 0.5 °C.
. For the ambient field condition (“0 mT”), the two samples were positioned far away from the magnets where the maximum static field was 60 μT (Earth’s field) and the maximum ac field was 3 μT. All magnetic fields were measured by using a Hall probe gaussmeter (Lakeshore). The temperatures in the vicinity of each sample were measured (TC-08; Pico Technology) before the experiment to ensure that they were equal to within 0.5 °C.
For the UE experiments, a specially designed dual Helmholtz coil system was built by Hirst Magnetic Instruments Ltd. See Fig. S2. The system comprises two separate coils, each made up of a double Helmholtz pair 160 mm in diameter (one is the sham coil S, and the other is the applied field coil F). One Helmholtz pair in each coil serves to cancel Earth’s field. The second pair can generate an additional applied field of up to approximately 10 mT. The two coils, S and F, differ only in the way the second set of windings is arranged. In the F coil both halves of the Helmholtz pair are wound with bifilar wires carrying current in the same sense (parallel fields), whereas in the S coil the halves are wound with a bifilar winding with opposite current in each winding so that no field is generated. The result is that only the F coil generates a net magnetic field at the center in response to an applied current. The arrangement ensures that heating and vibration effects are the same in both coils. Two independent circuits control Earth’s field compensation windings. The field current flows through both coils in series, and it is controlled by Labview software (National Instruments). Each coil has a thermocouple to monitor the temperature. The dual Helmholtz coils S and F were placed approximately 1 m apart to avoid any mutual stray fields. A magnetic field map of the location was prepared, and this map revealed that, with the arrangement described, the field at the S coil amounted to a few microteslas when a field of 10 mT was generated by the F coil. Both coils were isolated from any other field sources, and a field map showed no stray field above a few microteslas.
Dual Spectrophotometer System.
The two spectrophotometers (also denoted S and F in Fig. S2) are identical Ocean Optics USB4000 units. They contain Toshiba 3,648-element linear CCD detector arrays. The source is a deuterium-halogen UV lamp, model DH 2000, also supplied by Ocean Optics. Optical fibers were premium-grade quartz (jacketed) of 600 μm diameter. Collimating lenses were placed on each side of the coils, and the sample at the center was irradiated by a beam a few millimeters in diameter. Data were collected by using Ocean Optics Spectrasuite software. Temperature control of the two cells ensured that they never differed by more than 0.1 °C.
Supplementary Material
Acknowledgments.
We are very grateful to Gavin Davey and Keith Tipton for their helpful advice and to Andrew Bowie for the use of his luminometer. This work was supported by the Electromagnetic Field Biological Research Trust (United Kingdom), Science Foundation Ireland as part of the Magnetic Nanostructures and Spin Electronics project and by the Nanotechnological Toolkits For Multi-modal Disease Diagnostics and Treatment Monitoring Seventh Framework Program project.
Footnotes
The authors declare no conflict of interest.
See Commentary on page 1357.
This article contains supporting information online at www.pnas.org/lookup/suppl/doi:10.1073/pnas.1117840108/-/DCSupplemental.
References
- 1.Lacy-Hulbert A, Metcalfe JC, Hesketh R. Biological responses to electromagnetic fields. FASEB J. 1998;12:395–420. doi: 10.1096/fasebj.12.6.395. [DOI] [PubMed] [Google Scholar]
- 2.Woodward JR, Foster TJ, Jones AR, Salaoru AT, Scrutton NS. Time-resolved studies of radical pairs. Biochem Soc Trans. 2009;37:358–362. doi: 10.1042/BST0370358. [DOI] [PubMed] [Google Scholar]
- 3.Grissom CB. Magnetic field effects in biology: A survey of possible mechanisms with emphasis on radical-pair recombination. Chem Rev. 1995;95:3–24. [Google Scholar]
- 4.Brocklehurst B. Magnetic fields and radical reactions: Recent developments and their role in nature. Chem Soc Rev. 2002;31:301–311. doi: 10.1039/b107250c. [DOI] [PubMed] [Google Scholar]
- 5.Efimova O, Hore PJ. Role of exchange and dipolar interactions in the radical pair model of the avian magnetic compass. Biophys J. 2008;94:1565–1574. doi: 10.1529/biophysj.107.119362. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 6.Rodgers CT, Hore PJ. Chemical magnetoreception in birds: The radical pair mechanism. Proc Natl Acad Sci USA. 2009;106:353–360. doi: 10.1073/pnas.0711968106. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 7.Buchachenko A. Magnetic isotopy: New horizons. Her Russ Acad Sci. 2010;80:22–28. [Google Scholar]
- 8.Buchachenko A, Kouznetsov D. Efficiency of ATP synthase as a molecular machine. Biophys. 2008;53:219–222. [PubMed] [Google Scholar]
- 9.Buchachenko A, Kuznetsov D. Magnesium magnetic isotope effect: A key to the mechanochemistry of phosphorylating enzymes as molecular machines. Mol Biol. 2006;40:9–15. [PubMed] [Google Scholar]
- 10.Buchachenko AL. Magnetic isotope effect: Nuclear spin control of chemical reactions. J Phys Chem A. 2001;105:9995–10011. [Google Scholar]
- 11.Buchachenko AL, Kouznetsov DA, Arkhangelsky SE, Orlova MA, Markarian AA. Spin biochemistry: Intramitochondrial nucleotide phosphorylation is a magnesium nuclear spin controlled process. Mitochondrion. 2005;5:67–69. doi: 10.1016/j.mito.2004.10.001. [DOI] [PubMed] [Google Scholar]
- 12.Buchachenko AL, Kouznetsov DA, Breslavskaya NN, Orlova MA. Magnesium isotope effects in enzymatic phosphorylation. J Phys Chem B. 2008;112:2548–2556. doi: 10.1021/jp710989d. [DOI] [PubMed] [Google Scholar]
- 13.Buchachenko AL, Kouznetsov DA, Orlova MA, Markarian AA. Magnetic isotope effect of magnesium in phosphoglycerate kinase phosphorylation. Proc Natl Acad Sci USA. 2005;102:10793–10796. doi: 10.1073/pnas.0504876102. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 14.Buchachenko AL, Kouznetsov DA, Shishkov AV. Spin biochemistry: Magnetic isotope effect in the reaction of creatine kinase with CH3HgCl. J Phys Chem A. 2004;108:707–710. [Google Scholar]
- 15.Buchachenko AL, Kuznetsov DA. Magnetic field affects enzymatic ATP synthesis. J Am Chem Soc. 2008;130:12868–12869. doi: 10.1021/ja804819k. [DOI] [PubMed] [Google Scholar]
- 16.Buchachenko AL, et al. Dependence of mitochondrial ATP synthesis on the nuclear magnetic moment of magnesium ions. Dokl Biochem Biophys. 2004;396:197–199. doi: 10.1023/b:dobi.0000033528.69032.9b. [DOI] [PubMed] [Google Scholar]
- 17.Buchachenko AL, Kuznetsov DA, Breslavskaya NN. Ion-radical mechanism of enzymatic ATP synthesis: DFT calculations and experimental control. J Phys Chem B. 2010;114:2287–2292. doi: 10.1021/jp909992z. [DOI] [PubMed] [Google Scholar]
- 18.Buchachenko AL, Lukzen NN, Pedersen JB. On the magnetic field and isotope effects in enzymatic phosphorylation. Chem Phys Lett. 2007;434:139–143. [Google Scholar]
- 19.Buchachenko AL, Yasina LL, Belyakov VA. Magnetic and classical oxygen isotope effects in chain oxidation processes: A quantitative study. J Phys Chem. 1995;99:4964–4969. [Google Scholar]
- 20.Kouznetsov DA, Arkhangelsky SE, Berdieva AG, Khasigov PZ, Orlova MA. A novel electrophoretic technique designed to modify the ratio of magnesium isotopes inside the creatine kinase active sites. A preliminary report. Isotopes Environ Health Stud. 2004;40:221–227. doi: 10.1080/10256010410001689916. [DOI] [PubMed] [Google Scholar]
- 21.Amirshahi N, et al. Porphyrin-fullerene nanoparticles for treatment of hypoxic cardiopathies. Nanotechnol Russ. 2008;3:611–621. [Google Scholar]
- 22.Amirshahi N, et al. Fullerene-based low toxic nanocationite particles (porphyrin adducts of cyclohexyl fullerene-C(60)) to treat hypoxia-induced mitochondrial dysfunction in mammalian heart muscle. Arch Med Res. 2008;39:549–559. doi: 10.1016/j.arcmed.2008.05.007. [DOI] [PubMed] [Google Scholar]
- 23.McLeish MJ, Kenyon GL. Relating structure to mechanism in creatine kinase. Crit Rev Biochem Mol Biol. 2005;40:1–20. doi: 10.1080/10409230590918577. [DOI] [PubMed] [Google Scholar]
-
24.Lahiri SD, et al. The 2.1 A structure of Torpedo californica creatine kinase complexed with the
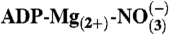 -creatine transition-state analogue complex. Biochemistry. 2002;41:13861–13867. doi: 10.1021/bi026655p. [DOI] [PubMed] [Google Scholar]
-creatine transition-state analogue complex. Biochemistry. 2002;41:13861–13867. doi: 10.1021/bi026655p. [DOI] [PubMed] [Google Scholar] - 25.Tornroth-Horsefield S, Neutze R. Opening and closing the metabolite gate. Proc Natl Acad Sci USA. 2008;105:19565–19566. doi: 10.1073/pnas.0810654106. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 26.Berg JM, Tymoczko JL, Stryer L. Biochemistry. 6th Ed. New York: Freeman; 2007. pp. 223–444. [Google Scholar]
-
27.Rezayat SM, et al. The porphyrin-fullerene nanoparticles to promote the ATP overproduction in myocardium:
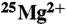 -magnetic isotope effect. Eur J Med Chem. 2009;44:1554–1569. doi: 10.1016/j.ejmech.2008.07.030. [DOI] [PubMed] [Google Scholar]
-magnetic isotope effect. Eur J Med Chem. 2009;44:1554–1569. doi: 10.1016/j.ejmech.2008.07.030. [DOI] [PubMed] [Google Scholar] - 28.Koltover VK, et al. Magnetic isotope of magnesium-25 stimulates growth of Escherichia coli cells: Preventive maintenance antioxidant effect by the nuclear spin. Free Radic Biol Med. 2009;47:S126. [Google Scholar]
- 29.Koltover VK. Nanotechnology 2010: Bio Sensors, Instruments, Medical, Environment and Energy—Technical Proceedings of the 2010 NSTI Nanotechnology Conference and Expo, NSTI-Nanotech 2010; Anaheim, CA: Nano Science and Technology Inst; 2010. pp. 475–477. [Google Scholar]
- 30.Eder M, Stolz M, Wallimann T, Schlattner U. A conserved negatively charged cluster in the active site of creatine kinase is critical for enzymatic activity. J Biol Chem. 2000;275:27094–27099. doi: 10.1074/jbc.M004071200. [DOI] [PubMed] [Google Scholar]
- 31.Muhlebach SM, et al. Sequence homology and structure predictions of the creatine kinase isoenzymes. Mol Cell Biochem. 1994;133–134:245–262. doi: 10.1007/BF01267958. [DOI] [PubMed] [Google Scholar]
- 32.Pickering L, Pang H, Biemann K, Munro H, Schimmel P. Two tissue-specific isozymes of creatine kinase have closely matched amino acid sequences. Proc Natl Acad Sci USA. 1985;82:2310–2314. doi: 10.1073/pnas.82.8.2310. [DOI] [PMC free article] [PubMed] [Google Scholar]
- 33.Schnyder T, Engel A, Lustig A, Wallimann T. Native mitochondrial creatine kinase forms octameric structures. II. Characterization of dimers and octamers by ultracentrifugation, direct mass measurements by scanning transmission electron microscopy, and image analysis of single mitochondrial creatine kinase octamers. J Biol Chem. 1988;263:16954–16962. [PubMed] [Google Scholar]
- 34.Berg C, et al. Stability and reactivity of hydrated magnesium cations. Chem Phys. 1998;239:379–392. [Google Scholar]
- 35.Cohn M. Magnetic resonance studies of specificity in binding and catalysis of phosphotransferases. Ciba Found Symp. 1975;31:87–104. doi: 10.1002/9780470720134.ch6. [DOI] [PubMed] [Google Scholar]
- 36.Lawson JW, Veech RL. Effects of pH and free Mg2+ on the Keq of the creatine kinase reaction and other phosphate hydrolyses and phosphate transfer reactions. J Biol Chem. 1979;254:6528–6537. [PubMed] [Google Scholar]
- 37.Madhusudan, Akamine P, Xuong N-H, Taylor SS. Crystal structure of a transition state mimic of the catalytic subunit of cAMP-dependent protein kinase. Nat Struct Biol. 2002;9:273–277. doi: 10.1038/nsb780. [DOI] [PubMed] [Google Scholar]
- 38.Ronner P, Friel E, Czerniawski K, Fraenkle S. Luminometric assays of ATP, phosphocreatine and creatine for estimation of free ADP and free AMP. Anal Biochem. 1999;275:208–216. doi: 10.1006/abio.1999.4317. [DOI] [PubMed] [Google Scholar]
- 39.Coey J. Magnetism and Magnetic Materials. Cambridge, UK: Cambridge Univ Press; 2010. pp. 32–475. [Google Scholar]
Associated Data
This section collects any data citations, data availability statements, or supplementary materials included in this article.