Factors Influencing the Development of School Bonding Among Middle School Students

Abstract

The goal of this research is to examine the trajectory of school bonding over the middle school period and how factors such as gender, substance use, antisocial peers, delinquent behavior, and academic achievement affect this developmental process. Data from 4 waves of measurement of 2,902 adolescents are analyzed using hierarchical growth curve modeling. Results suggest that school bonding decreases in a non-linear fashion from Grade 6–8. However, school bonding development varies based on inter-individual differences. Boys have lower initial levels and greater decreases in school bonding than girls. Student deviant behavior, having antisocial peers, and low academic achievement are associated with lower levels of school bonding at Grade 6. Low grades and an increase in substance use are associated with a steeper decrease of school bonding over time. Increases in substance use and being male are also associated with a curvilinear pattern of school bonding. Implications for interventions are discussed.

Youth have a strong psychological need to belong and form attachments (Baumeister & Leary, 1995; Osterman, 2000). Schools are one important source of such attachments for adolescents. In fact, Catalano and Hawkins (1996) identify schools as “important agents of socialization” (p. 172). Youth that are strongly bonded to their school are more likely to be academically motivated and successful (Goodenow, 1993; Osterman, 2000; Roeser & Eccles, 1998; Wentzel, 1997, 1998), and are less likely to engage in youth problem behavior, such as delinquency, violence, and substance use (Battistich & Hom, 1997; Hawkins, Guo, Hill, Battin-Pearson, & Abbott, 2001; Lonczak, Abbott, Hawkins, Kosterman, & Catalano, 2002; McBride et al., 1995). Despite its importance as a developmental construct and potential as an intervention target, little is known about the development of school bonding over time. This study uses multilevel growth modeling to investigate the normative development of school bonding over the middle school period and how factors, such as gender, problem behavior, antisocial peers, and grades affect this developmental process.

Defining school bonding

The concept of school bonding captures the connections students experience at their school; the extent to which they feel cared for and respected by their teachers and attached to their school, their level of participation and involvement in their school, and their commitment to the values and beliefs of the school (Battistich, Schaps, & Wilson, 2004; Catalano & Hawkins, 1996; Maddox & Prinz, 2003). However, the measurement of school bonding has varied widely between studies (Libbey, 2004; Maddox & Prinz, 2003). For example, studies of the Social Development Model define three distinct elements of school bonding: attachment to the school, commitment to the school, and belief in the school values (Catalano & Hawkins, 1996). Other studies, such as Roeser and Eccles (1998) and Murray and Greenberg (2000), focus on perceptions of the student-teacher relationship and/or the extent to which students feel cared for by their teachers. Goodenow (1993) defines a subjective sense of school belonging as “students sense of being accepted, valued, included, and encouraged by others in the academic classroom setting and feeling of oneself to be an important part of the life and activity of the class” (p. 25).

School bonding as a protective factor

School bonding may be a salient intervention target for school based interventions and an important pathway to reduce youth problem behavior. Regardless of how school bonding is conceptualized, high levels of school bonding have been consistently associated with positive youth outcomes, including academic outcomes, such as increased academic motivation, self-efficacy, and higher grade point averages (Goodenow, 1993; Osterman, 2000; Roeser, Eccles, & Sameroff, 2000; Wentzel, 1997; Wentzel, 1998) and low levels of problem behavior (e.g. substance use, risky sexual activity, truancy, bullying, fighting, stealing, and vandalism; Battistich & Hom, 1997; Hawkins et al., 2001; Lonczak et al., 2002; McBride et al., 1995; Roeser & Eccles, 1998; Simon-Morton et al., 1999). The Social Control Theory (Hirschi, 1969) and the Social Development Model (Catalano & Hawkins, 1996) suggest that youth bonds to their schools may influence the extent to which they are bonded to other deleterious influences, such as devious peers. Strong bonds to prosocial institutions, such as schools, may lead to the internalization of positive values and act as informal controls to behavior (Catalano & Hawkins, 1996). By targeting school bonding in school based interventions, we may help youth internalize positive values and prevent the emergence of problem behavior. Understanding the developmental process of school bonding may help us understand mechanisms to strengthen school bonding and may help the field refine our school based interventions.

Despite the importance of school bonding to prevention, we know little about how school bonding changes over middle school and what individual factors influence its development. A few studies suggest that there are normative changes in school bonding over the middle school period and that the development of school bonding may vary depending on individual factors such as problem behavior, peers, grades, and gender (Blankmeyer, Flannery, & Vazsonyi, 2002; Catalano & Hawkins, 1996; Maddox & Prinz, 2003).

Normative Changes in School Bonding

A few studies have found that on average, levels of school bonding may decline over adolescence, particularly during the middle school transition, as peer and school contexts often change. Characteristics of middle schools, such as a greater number of teachers and an increased focus in student competition, may lead to less intimacy with teachers and subsequent declines in bonding (Eccles, 2004, Eccles & Midgley, 1989). Simons-Morton, Crump, Haynie, and Saylor (1999) found student attitudes towards school became increasingly negative between sixth and eighth grade. Similarly, O' Donnell, Hawkins, Catalano, Abbott, and Day (1995) found decreasing levels of school bonding from age 13 – 18. Roeser and Eccles (1998) found mean decreases in the value that students place on school between seventh and the end of eighth grade.

Factors Associated with School Bonding

Although the mentioned studies suggest that school bonding declines over time, they do not specifically explore which characteristics of youth influence changes in school bonding. Research has found consistent correlations of school bonding and a host of individual factors such as youth academic achievement, problem behavior, and gender (Blankmeyer, Flannery, & Vazsonyi, 2002; Catalano & Hawkins, 1996; Maddox & Prinz, 2003). A number of studies have found that engagement in problem behavior may be related to subsequent reductions in school bonding (Bryant et al., 2000; Maddox & Prinz, 2003). Aggressive and antisocial children may be less likely to form positive student-teacher relationships (Blankmeyer, Flannery, & Vazsonyi, 2002). Roeser and Eccles (1998) found that positive teacher regard was associated with increases in the value a student places on school between seventh and eighth grade. Further, developmental patterns of school bonding may differ between girls and boys (Brookmeyer, Fanti, & Henrich, 2006). Simons-Morton et al. (1999) found that girls reported higher levels of school bonding than boys in the middle school years. Goodenow (1993) found a stronger association between middle school student perceptions of belonging and academic motivation for girls than boys. Blankemeyer, Flannery, and Vazsonyi (2002) found that girls with poor school adjustment had more positive perceptions of their relationship with teachers than poorly adjusted boys.

This Study

Our knowledge on the development of school bonding is limited, as many of the studies on school bonding conceptualize it as a predictor for specific student outcomes, not as an outcome itself. No explicit analysis has explored the development of school bonding and how factors such as problem behavior, antisocial peers, grades, and gender affect the growth of school bonding over the middle school period. Understanding the developmental process of school bonding is important for designing interventions to strengthen it. In this paper, we examine the developmental process of school bonding. First, we examine the construct of school bonding itself, determining the most appropriate measurement model for our data. Second, we estimate the developmental trajectory of school bonding from Grade 6 through Grade 8 among a sample of rural adolescents. Finally, we examine how factors including gender, academic achievement, substance use, deviant behavior, and peer relations affect the growth of school bonding during the middle school years. We have three hypotheses. First, we hypothesize that school bonding will decline between sixth and eighth grade as part of normative development. Second, we expect that the pattern of school bonding over time will be different for girls than boys; boys will show a greater decline across the middle school years. Third, associations with deviant peers, prior delinquent behavior, substance use, and poor academic achievement will be associated with a lower initial level of school bonding in sixth grade and a faster decline in school bonding over the middle school period.

Method

Sample

The data for this study are drawn from the PROSPER project, a large scale effectiveness trial of preventive interventions aimed to reduce substance use among middle school students (Spoth, Greenberg, Biermann, & Redmond, 2004). Data were collected from in-school surveys of two successive cohorts of youth from 28 project communities in two states (Iowa and Pennsylvania). Seven small towns and rural communities in each state were randomly assigned to the PROSPER intervention condition, while the remaining 14 were assigned to a wait-list control group. Because the goal of this paper is to identify the development of school bonding and its antecedents, not intervention effects, we only used data from the cohort one control condition. Informed consent procedures, approved by the Institutional Review Board, were followed during data collection. For data collected in-school, parents were sent a letter prior to the administration of the survey giving them the option to decline study participation. All youth who participated in the in-school survey signed an assent form before taking the survey.

Four waves of measurement were used: the Fall of Grade 6 (Fall 2002), Spring of Grade 6 (2003), Spring of Grade 7 (2004), and Spring of Grade 8 (2005). To handle missing values of the study variables, a multiple imputation with LISREL (Jöreskog & Sörbom, 1989) was performed. The final sample includes data from 2,902 youth. The mean age of the study participants is 11.83 years and 51.10 % were female. A summary of the demographic variables can be found in Table 1.

Table 1.

Demographics of the Final Sample at the First Point of Measurement (valid cases only)

	State
	Pennsylvania (n=1390)	Iowa (n=1501)	Total
Mean Age (SD)	11.81 (0.43)	11.85 (0.39)	11.83 (0.41)
Gender (%)
Female	714 (51.40)	762 (50.90)	1,476 (51.10)
Male	675 (48.50)	736 (49.10)	1,411 (48.90)
Race (%)
Non-White	222 (16.70)	257 (18.70)	480 (17.70)a
White	1,104 (83.30)	1,120 (81.30)	2,233 (82.30)
Biological Parents (%)
Both biological parents	849 (63.60)	836 (59.90)	1,689 (61.60)
Other	486 (36.40)	559 (40.10)	1,051 (38.40)
Marital Status (%)
Single parent	291 (21.80)	340 (24.40)	634 (23.10)
Dual parent	1,044 (78.20)	1,055 (75.60)	2,106 (76.90)
School Transition (%)
no transition	172 (12.40)	0 (0.00)	172 (6.00)
before 6th Grade	555 (40.20)	1,250 (83.80)	1,805 (62.80)
before 7th Grade	655 (47.40)	242 (16.20)	897 (31.20)

Note. States differ significantly in mean age of students and school transition time point.

^aThe category “Non-White” consists of 164 Latino/Hispanic people, 100 Black/African American, 50 Native American/American Indian, 37 Asian and 129 other people.

Measures

For this analysis, we used measures of school bonding as well as measures of predictors of school bonding; gender (0 = girls, 1 = boys), experience with alcohol and drugs, deviant behavior, contact with antisocial peers, and grades. Correlations of these measures are found in Table 2. All measures were youth-report and were gathered at each of the four measurement occasions. In this analysis, we used values of all the predictor variables at sixth grade. Additionally, weighted change scores for the five predictor variables were calculated by taking the average change in a variable and multiplying it by the interval length between measurement occasions:

$${\mathrm{p}}^{\ast }=\sum _{x=1}^3({\mathrm{p}}_{x+1}-{\mathrm{p}}_x)\times {\mathrm{I}}_x\begin{array}{c}{\mathrm{p}}^{\ast }=\text{weighted change variable of predictor p}\hfill \\ {\mathrm{p}}_x=\text{predictor variable at time point x}\hfill \\ {\mathrm{I}}_x=\text{Interval}\left(inyears\right)\text{between time point x and}(x+1)\hfill \end{array}$$

A positive value of a weighted change indicates an average increase over time (and thus more problematic behavior), whereas a negative value suggests a decrease over time. In addition, we included the variable state (0 = Pennsylvania and 1 = Iowa) and a variable on the timing of school transitions. Descriptive statistics on these predictors can be found in Table 2.

Table 2.

Descriptive Statistics and Correlations of the Predictors at sixth grade and Descriptive Statistics of the weighted Change Variables

Descriptive Statistics	n	min	max	Mean	sd
Substance Use	2710	0	7	0.30	0.75
Deviant Behavior	2740	1	5	1.11	0.31
Antisocial Peers	2741	1	5	1.51	0.82
Grades	2607	1	5	1.82	0.82
Weighted Change Substance Use	1632	0	2.33	0.32	0.44
Weighted Change Deviant Behavior	1816	−1.33	1.33	0.05	0.16
Weighted Change Antisocial Peers	1795	−1.17	1.33	0.18	0.32
Weighted Change Grades	1539	−0.67	1.33	0.05	0.24

Correlations
	Substance Use	Deviant Behavior	Antisocial Peers	Grades
Substance Use	1
Deviant Behavior	0.55**	1
Antisocial Peers	0.32**	0.43**	1
Grades	0.14**	0.17**	0.20**	1

Note. n = Number of valid cases, min = Minimum, max = Maximum, SD = Standard Deviation

^*p < 0.05,

^**p < 0.01

School bonding

School bonding was measured with eight items (e.g., “I like school a lot.”, “I try hard at school.”, “Grades are very important to me.”, “I feel close to at least one of my teachers.”, “I get along well with my teachers.”) which constitute one distinct factor. The answers were coded on a five point Likert-Scale with the values 1 (never true), 2 (seldom true), 3 (sometimes true), 4 (usually true), and 5 (always true). The school bonding scale was adapted from a scale developed by Simons, Whitbeck, Conger, & Conger (1991) and is similar to other items used in the literature to measure school bonding. For a review see Libbey (2004). Higher values indicate higher levels of school bonding. The internal consistency of the scale is .76.

Substance use

The poly-substance intoxication index asks youth seven questions about their alcohol and drug use (e.g. “Have you ever smoked a cigarette?”, “Have you ever drunk more than just a few sips of alcohol?”) which are answered with 0 (no) or 1 (yes). The questions ask if the youth has ever drunk alcohol, smoke cigarettes, marijuana or hashish, sniffed glue or paint, used ecstasy, used prescription hard drugs or medication, and if the youth has ever used Vicodin, Percocet, or Oxycontin. The index ranges from 0 to 7, with 7 indicating use of all substances. The internal consistency of the measure is .60.

Deviant behaviors

A student's deviant behavior was assessed with a 12 item scale that asks about theft and burglary, fighting, vandalism, breaking into homes, running away, contact with police and carrying a weapon (e.g. “During the past 12 years, how many times have you taken something worth $25 or more that didn't belong to you?”). The answers are coded on a Likert-Scale with 1 (Never), 2 (Once), 3 (Twice), 4 (Three or four times), 5 (Five or more times). Internal consistency of the scale is .83.

Antisocial Peer Behavior

The antisocial behavior of the student's peer group was assessed with three questions measuring level of agreement with 1 (strongly disagree), 2 (disagree), 3 (neutral), 4 (agree), and 5 (strongly agree) to statements about their closest friends; if their friends had trouble with the police, if they broke the law, and if they get along with their parents (“These friends sometimes get into trouble with police.”). Internal consistency of the scale is .80.

Grades

Grades were measured by the question “What grades do you generally get in school?” using a five point scale: 1 (Mostly A's), 2 (Mostly B's), 3 (Mostly C's), 4 (Mostly D's), and 5 (Mostly lower than D's).

Timing of School Transition

A variable indicating if children transitioned to a new school before Grade 6 with the values 0 (no transition in sixth grade) and 1 (transition in sixth grade) was created to account for school transition effects.

Data Analysis

Data analysis consists of four steps. We first computed and compared measurement models for the school bonding construct. Next, we used latent growth curve models to identify the trajectory of school bonding over four measurement occasions by a common group growth curve (e.g., similarities in development among the youth in our sample) as well as inter-individual differences via variability around this group curve (Duncan, Duncan, Strycker, Li, & Alpert, 1999; McArdle, 2006; McArdle & Epstein, 1987; McArdle & Nesselroade, 2003; Rovine & Molenaar, 1998, 2000). A quadratic growth curve model with a level (π₀), linear (π₁) and quadratic growth component (π₂) was assumed as a starting point for a series of successive model tests to assess the best fitting model (no growth, linear growth or quadratic growth model). The general equations for the models used are

$${\mathrm{Y}}_m={\pi }_0+{\pi }_1\times {\mathrm{t}}_m+{\pi }_2\times {\mathrm{t}}_m^2+{\epsilon }_m\text{and}$$

$${\pi }_k={\beta }_k+{\mathrm{r}}_k$$

The Y_m is the observed value of school bonding at measurement occasion m, ε_m is a residual term for measurement occasion m. The equation for the Y_m represents the first level; the level of measurement occasions. The parameters π_k represent growth curve components; π₀ is the level component, π₁ represents the linear component (linear slope) and π₂ is the quadratic component. The components consist of a mean β_k (representing the mean over all persons) and a deviation from that mean r_k (McArdle & Nesselroade, 2003). The means are “fixed effects” and as such, they do not vary between persons. However, deviations from that mean are different from person to person (“random effects”). Thus, the equations for the latent variables π represents a second level; the person level. Finally, the parameter t represents the time coding which is crucial for interpretation of the parameters (Biesanz, Deeb-Sossa, Papadakis, Bollen, & Curran, 2004; Duncan et al., 1999; Hancock & Lawrence, 2006; McArdle, 2006; McArdle & Nesselroad, 2003; Stoolmiller, 1995). The time vector was chosen as t = [0, 0.5, 1.5 and 2.5] making it possible to interpret the first growth component π₀ (level component) as the extent of school bonding at the first point of measurement and thus at sixth grade (Y₁ = π₀).

After determining the best fitting growth curve model, we expand it to a three level model (third step of analysis) by adding the school level equation, allowing us to account for possible systematic variation in the development of school bonding between schools. A mean value β_kij of a person i within a school j will be assumed to consist of the grand mean of all persons γ and a (school specific) deviation from that mean called u:

$${\beta }_{\mathrm{kij}}={\gamma }_{\mathrm{kij}}+u_{\mathrm{kij}}$$

HLM 6.06 (Raudenbush, Bryk, & Congdon, 2008) was used to analyze this hierarchical model.

In the last step of analysis, predictor variables p_i were added to the model. Gender, substance use, antisocial peers, deviant behavior, grades, and the weighted change variables were introduced into the hierarchical model at the second level to explain the variation in the growth curve of school bonding and to account for between-person variance.

$${\pi }_k={\beta }_{\mathrm{k}0}+{\beta }_{\mathrm{k}1}\times {\mathrm{p}}_1+\dots +{\beta }_{\mathrm{ki}}\times {\mathrm{p}}_{\mathrm{i}}+{\mathrm{r}}_{\mathrm{k}}$$

With the exception of gender, all variables were grand mean centered by subtracting each student's value on the predictor variable from the sample mean. Thus, in these models, the intercept is the predicted score of an individual whose value for that predictor variable is equal to the grand mean. Values of the predictor variables at Grade 6 were treated as antecedences of school bonding development and therefore used as predictors for all three growth curve components (the level of school bonding at sixth grade, the linear growth rate, and the quadratic growth rate). The weighted change variables of substance use, antisocial peers, deviant behavior, and grades also were used to predict between-person differences in the growth of school bonding over time (the linear and quadratic growth curve component). The predictor variables state and the school transition time variable (uncentered) were added to explain variance at the school level (third level):

$${\beta }_{\mathrm{kij}}={\gamma }_{\mathrm{ki}0}+{\gamma }_{\mathrm{ki}1}\times \mathrm{p}+\dots +{\gamma }_{\mathrm{ki}1}\times {\mathrm{p}}_{\mathrm{j}}+{\mathrm{u}}_{\mathrm{ki}}$$

with p_j representing third-level predictors.

Results

Measurement Model for School Bonding

LISREL 8.3 (Jöreskog & Sörbom, 1989) was used to analyze the factor analytic structure of the scale by estimating measurement models and analyzing the model fit. Results favor a model with longitudinal varying, congeneric variables. A model with congeneric variables, where items were allowed to load differently within each time point, showed a better fit than a tau-equivalent model where all items loaded equally with a value of one (χ²-difference = 1200.02 with df = 7, p < 0.00). Further, a model where loadings of each item were allowed to vary across time had a significantly better Chi-Square (χ²-deviation = 284.41, df-deviation = 21, p < 0.00) compared to a model assuming longitudinal invariance. The values of the Root Mean Square Error of Approximation (RMSEA=0.06) and the Standardized Root Mean Residual (SRMR=0.05), the Comparative Fit Index (CFI = 0.97) and the Non-normed Fit Index (TLI/NNFI = 0.96) indicate a good model fit (Hu & Bentler, 1999). Thus, the model with unequal loadings on the school bonding factor across time points was used for further analysis. Finally, the means of the school bonding variables at each occasion were estimated freely (which changed the model fit slightly). The parameter estimates of the final measurement model including means can be found in Table 3. Loadings on the school bonding scale increased over time except for item 4 (“School bores me”) indicating a change in the relative importance of items over time. The latent variable scores (Jöreskog, 2000) of the four factor variables of the measurement model are used as scale scores of school bonding for further analysis. Thus, each youth in our sample has one value of school bonding at each time point, representing total school bonding.

Table 3.

Parameter Estimates of the Final Measurement Model for the School Bonding Construct

Loadings for every measurement time point
	t1 (T-value/SE)	t2 (T-value/SE)	t3 (T-value/SE)	t4 (T-value/SE)
λ 1	1.00	1.00	1.00	1.00
λ 2	1.22 (190.65/0.01)	1.25 (190.21/0.01)	1.28 (179.54/0.01)	1.28 (179.36/0.01)
λ 3	1.25 (189.52/0.01)	1.28 (186.08/0.01)	1.31 (176.20/0.01)	1.32 (175.31/0.01)
λ 4	0.96 (141.29/0.01)	0.94 (137.92/0.01)	0.92 (131.97/0.01)	0.89 (134.10/0.01)
λ 5	1.20 (163.40/0.01)	1.22 (156.85//0.01)	1.29 (154.88/0.01)	1.30 (158.40/0.01)
λ 6	0.97 (120.28/0.01)	0.96 (117.040.01)	0.99 (115.990.01)	1.02 (119.19/0.01)
λ 7	1.17 (173.83/0.01)	1.18 (171.33/0.01)	1.22 (167.70/0.01)	1.23 (168.04/0.01)
λ 8	1.22 (172.34/0.01)	1.25 (166.26/0.01)	1.32 (160.21/0.01)	1.36 (162.14/0.01)
Error Variance of the observed School Bonding variables for every time point
ε 1	0.81 (35.91/0.02)	0.74 (35.61/0.02)	0.68 (35.42/0.02)	0.63 (35.47/0.02)
ε 2	0.41 (31.42/0.01)	0.38 (30.31/0.01)	0.42 (30.79/0.01)	0.38 (30.80/0.01)
ε 3	0.45 (31.76/0.01)	0.47 (31.55/0.01)	0.50 (31.74/0.02)	0.48 (31.91/0.01)
ε 4	1.07 (36.57/0.03)	1.01 (36.46/0.03)	0.89 (36.36/0.02)	0.73 (36.28/0.02)
ε 5	0.96 (35.41/0.03)	1.06 (35.36/0.03)	0.96 (34.92/0.03)	0.81 (34.59/0.02)
ε 6	1.79 (37.17/0.05)	1.72 (37.10/0.05)	1.53 (36.93/0.04)	1.39 (36.84/0.04)
ε 7	0.67 (34.46/0.02)	0.66 (34.14/0.02)	0.58 (33.39/0.02)	0.54 (33.33/0.02)
ε 8	0.76 (34.64/0.02)	0.85 (34.70/0.02)	0.87 (34.40/0.03)	0.79 (34.18/0.02)
Means of the latent School Bonding variables
α	3.65 (194.07/0.02)	3.46 (184.34/0.02)	3.17 (170.73/0.02)	3.05 (170.26/0.02)
Variance-Co variance-Matrix of the latent School Bonding variables
t1	0.23 (28.63/0.01)
t2	0.20 (26.66/0.01)	0.30 (30.25/0.01)
t3	0.17 (22.62/0.01)	0.22 (25.87/0.01)	0.34 (31.09/0.02)
t4	0.14 (20.99/0.01)	0.19 (23.85/0.01)	0.25 (28.09/0.01)	0.32 (31.21/0.02)

Note. SE = Standard Error; all parameter estimates are significant.

Growth curve model (Two-Level Model)

To determine the best fitting model of the growth of school bonding, we proceeded through a series of successive model tests to assess level (π₀), linear (π₁), and quadratic components (π₂). The model including a quadratic component was the best fitting model with both the RMSEA and the SRMR being smaller than 0.06 and the CFI and NNFI being close to one (Hu & Bentler, 1999). The χ²-deviation between the model with and without the quadratic component was significant (χ²-deviation = 630.36, df-deviation = 4, p < 0.00). The high values of the squared multiple correlations for the different time points (0.96, 0.72, 0.80, and 0.89) indicate the latent scores of school bonding have adequate reliability (Jöreskog & Sörbom, 1989).

Model estimations can be found in Table 4. The mean value of school bonding at sixth grade is π₀ = 3.66. The total average mean difference between the first measurement point and the last one is 0.60, indicating that school bonding decreases by 1.25 standard deviations between Grade 6 and 8. The decrease in school bonding across middle school is non-linear, but has a quadratic shape, indicated by a negative linear growth component of 0.44 and a small but significant positive quadratic component of 0.08. An illustration of the shape of the common growth curve is seen in Figure 1. Random-variance estimates of all three growth curve components (r₀ to r₂) are significant, suggesting that the level of school bonding in sixth grade and the shape of the growth curve over time vary considerably between children. In addition, the growth curve model does not account for all of the individual differences in school bonding growth for students in the sample indicated by the significant estimates of the residual variances for the second and third measurement occasion (ε₂ and ε₃).

Table 4.

Parameter Estimates of the Quadratic Growth Curve Model (Two-Level-Model)

	Value	T (SE)
Fixed Effects
Means of the Growth Curve Components
β0 (Level)	3.66*	410.64 (0.01)
β1 (Linear)	−0.44*	−38.72 (0.01)
β2 (Quadratic)	0.08*	19.25 (<0.01)
Random Effects
Residual Variance
Var (ε1)	0.01	1.66 (0.01)
Var (ε2)	0.08*	21.90 (<0.01)
Var (ε3)	0.07*	11.74 (0.01)
Var (ε4)	0.03	1.95 (0.02)
Variance of the Growth Curve Components
Var (π0) = r0	0.22*	27.13 (0.01)
Var (π1) = r1	0.21*	11.92 (0.02)
Var (π2) = r2	0.02*	10.22 (<0.00)
Covariance between Growth Curve Components
Cov(π0, π1)	−0.05*	−4.79 (0.01)
Cov(π0, π2)	0.01*	2.06 (<0.00)
Cov(π1, π2)	−0.06*	−11.29 (0.01)

Note. SE = Standard Error, T = T-value

^*p < .05

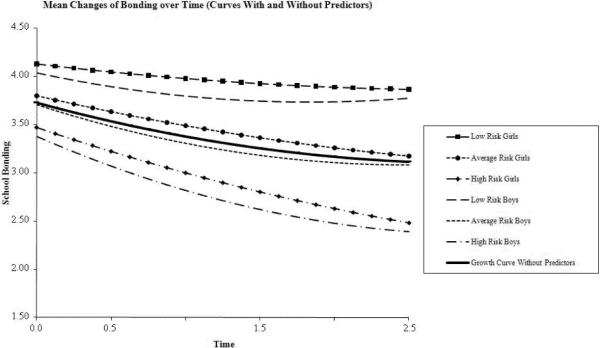
Figure 1.

Mean Changes of Bonding over Time (Curves With and Without Predictors)

Three-Level Model

The quadratic growth curve model was extended to a three-level model to assess the influence of schools on school bonding growth (between schools is level 3). Parameter estimates of the three-level model are almost identical to those in the two-level model. The mean level of school bonding in Grade 6 is estimated as π₀ = 3.73, the mean linear growth rate as π₁ = −0.42, and the quadratic growth curve component with a value of π₂ = 0.07. The mean value of school bonding at sixth grade and the linear growth rate of school bonding differ between schools (u₀₀ and u₁₀). The random variance estimates on the third level, while significant, are relatively small (u₀₀ and u₁₀ are both < 0.01) compared to the variance estimates on the second level (r₀ = 0.14, r₁=0.12, and r₂=0.01). Therefore, the development of school bonding varies between schools, but the inter-individual variation is substantially higher.

Predictor Variables for School Bonding Development (Conditional Model)

The variables antisocial peer behavior, grades, substance use, and deviant behavior at sixth grade, the weighted change of these variables, and gender were added to the unconditional three-level model as predictor variables at the second level (person-level). The third-level predictors state and transition time were added for relevant significant third-level random effects only. The significant random effects at the third-level were determined by a first run of the model: the random variance of the mean level of school bonding at sixth grade (u₀₀ < 0.01) and the random variance estimated of the effect of the predictor antisocial peer behavior on the mean level of school bonding at sixth grade (u₀₄ = 0.01) were significant. Results of the final analysis with all predictors are presented in Table 5.

Table 5.

Parameter Estimates of the Three-Level Model with Predictors

	Value	T (SE)
Fixed: Main effects and Interactions
Level component π0 (γ000)	3.75***	140.03 (0.03)
γ001 = State	0.02	0.51 (0.03)
γ002 = Transition in sixth grade	0.03	0.85 (0.03)
γ010 = Gender (Male)	−0.09***	−4.44 (0.02)
γ020 = Substance Use	−0.06*	−2.66 (0.02)
γ030 = Deviant Behavior	−0.39***	−4.04 (0.03)
γ040 = Antisocial Peers	−0.12**	−4.04 (0.03)
γ041 = State	< −0.01	−0.08 (0.03)
γ042 = Transition in sixth grade	0.06	1.94 (0.03)
γ050 = Grades	−0.14***	−9.86 (0.02)
Linear component π1	−0.35***	−13.40 (0.03)
γ110 Gender (Male)	−0.15***	−4.32 (0.03)
γ120 Substance Use	−0.02	−0.49 (0.04)
γ130 Deviant Behavior	0.20	1.79 (0.11)
γ140 Antisocial Peers	0.07	1.56 (0.04)
γ150 Grades	<0.01	<0.01 (0.02)
γ160 Weighted Change Substance Use	−0.37***	−7.44 (0.05)
γ170 Weighted Change Deviant Behavior	−0.17	−1.15 (0.15)
γ180 Weighted Change Antisocial Peers	−0.03	−0.45 (0.07)
γ190 Weighted Change Grades	−0.31***	−4.39 (0.07)
Quadratic component π2	0.04**	4.43 (0.01)
γ210 Gender (Male)	0.06***	4.41 (0.01)
γ220 Substance Use	0.01	0.64 (0.01)
γ230 Deviant Behavior	−0.05	−1.12 (0.04)
γ240 Antisocial Peers	−0.02	−1.52 (0.02)
γ250 Grades	−0.01	−0.88 (0.01)
γ260 Weighted Change Substance Use	0.12***	5.01 (0.02)
γ270 Weighted Change Deviant Behavior	−0.01	−0.16 (0.06)
γ280 Weighted Change Antisocial Peers	−0.04	−1.46 (0.03)
γ290 Weighted Change Grades	0.01	0.18 (0.03)

	Value	χ2 (df)
Random: Variance components
Level-1 (ε)	0.07	--
Level-2
Level (r0)	0.08***	3250.67 (1261)
Linear (r1)	0.07***	1608.36 (1218)
Quadratic (r2)	0.01***	1514.59 (1205)
Level-3 (only relevant significant random effects are displayed)
u00 (variance of π0 between schools)	< 0.01**	34.08 (11)
u04 (effect of antisocial peers on π0)	< 0.01*	24.25 (11)

Note. SE = Standard Error, T = T-value, χ² = Chi-Square, df = Degrees of Freedom

^*p < .05,

^**p < .01,

^***p < .001

Level of school bonding in Grade 6

The overall average initial level of school bonding γ₀₀₀ has a value of 3.75. This value represents the average level of school bonding at the first occasion for girls who display average values on the other second-level predictor variables. A significant fixed effect of gender on the intercept of the growth curve (γ₀₁₀ = −0.09) was found, indicating that boys have a lower initial level of school bonding at the sixth grade than girls. Further, youth with higher rates of substance use (γ₀₂₀ = −0.06), deviant behavior (γ₀₃₀ = −0.39), more antisocial peer groups (γ₀₄₀ = −0.12), and lower grades (γ₀₅₀ = −0.14) had lower levels of school bonding in sixth grade as indicated by the significant fixed effects of these predictor variables for the intercept in our models.

The overall initial level of school bonding (parameter γ₀₀₀) varies significantly between different schools (indicated by significant random variance at third-level). However, the variance accounted for is very small. The effect that antisocial peer groups have on the level of school bonding in sixth grade also varies significantly between schools, as seen by a significant random effect for this variable at the third level. However, neither the variable state nor the variable school transition can explain the random variance on the third-level.

The growth of school bonding between Grade 6 and 8

The overall linear growth rate of school bonding is significant and negative; indicating that on average, school bonding declines over middle school. However, a significant effect of gender (γ₁₁₀ = −0.15) indicates a steeper linear decline in school bonding for boys than for girls. The value of the linear growth curve component for a girl with average values on the predictors is −0.35, for a boy it is −0.50. In addition, the weighted average change from sixth to eighth grade for substance use (γ₂₂₀ = −0.37) and grades (γ₂₉₀ = −0.31) were also significant, suggesting that higher increases in substance use and greater reductions in grades over middle school were associated with a steeper decline in school bonding over middle school.

The overall quadratic development of school bonding is positive (γ₂₀₀ = 0.04), indicating a slowing-down effect of the decrease in school bonding over time. The quadratic component varies by gender and is higher for boys, suggesting that the growth curve of school bonding is more curvilinear for boys than for girls. Students with an increase in substance use among middle school have a more curvilinear pattern of the development of school bonding than students who don't show increases in substance use as indicated by a significant fixed effect of the weighted change in substance use (γ₂₆₀ = 0.12).

Further, there are no relevant random effects on the third-level for the linear growth rate and the quadratic growth curve component; indicating no variation in the growth of school bonding between schools. Thus, while the level of school bonding in sixth grade does appear to depend on school context, the growth of school bonding over time is less dependent on school context, indicating declines in school bonding may be a part of normative development. In contrast, the random effects for all three growth curve components on the second level (person level) are still significant, indicating inter-individual differences in the growth of school bonding between children cannot be explained fully by the model.

A visual illustration of the variation in growth curves by predictor variables can be found in Figure 1. The prototypical curves for boys and girls with average values on the second-level predictors are presented as well as the curves for boys and girls with values one standard deviation above the mean values of all predictors (high-risk) and below the mean value of all predictors (low-risk). All graphics represent youth in Pennsylvania who made a school transition in sixth grade. As illustrated, boys have a lower level of school bonding at Grade 6 than girls. High-risk youth also have lower levels of bonding at Grade 6 than low-risk youth. However, all students show a decrease in school bonding over time. The decrease is generally stronger for boys and the growth curves for boys are more curvilinear than those for girls. At-risk youth also have a steeper decrease in school bonding over time and a more curvilinear trajectory than low risk youth. The greatest decreases in school bonding are for prototypical boys and girls with values one standard deviation above the average values of the second-level predictors.

Discussion

Results of this study lend support for previous research findings indicating that school bonding decreases during the middle school years. However, the study extends existing research by suggesting that the development of school bonding may be non-linear and more complex than a simple decrease would indicate. In fact a significant quadratic component was found, suggesting a curvilinear shape of the trajectory of school bonding over the middle school period. Complex growth needs to be considered in future research on school bonding.

Second, the results suggest that the decline of school bonding over the middle school period may be a part of normative adolescent development (Simons-Morton, Crum, Haynie, & Saylor, 1999). The common growth components (linear and quadratic growth curve components) did not vary significantly between schools in our final model with all predictors included. This result is somewhat contrary to the results of the third-level model without predictors, in which the linear growth rate did vary between schools. Models of school bonding that include person level characteristics such as gender and problem behavior may be necessary to account for systematic differences in student populations at different schools.

However, our findings suggest that schools in general might not be suitable to satisfy developmental needs of students. Eccles and colleagues argue that the structure of middle school may not match the developmental needs of early adolescents, making the transition to middle school a critical period of development (Eccles, 2004; Eccles & Midgley, 1989). As part of normative adolescent development, students may become less oriented towards their school and thus experience a reduction in school bonding. Although the growth of school bonding over time does not vary between schools, levels of school bonding in the sixth grade do vary at the school level. However, none of our third-level predictors could explain the varying levels of school bonding in sixth grade. Further research is needed to explore the reasons for different school bonding levels in sixth grade in different school in order to strengthen school bonding in all schools.

The growth of school bonding over time also varied between persons. Gender appears to significantly impact the trajectory of school bonding and was associated with differences in all three growth curve components. Boys have lower values of school bonding at the entrance to Grade 6 than girls, a steeper decrease in school bonding over the course of middle school, and a higher quadratic component, indicating a more curvilinear shape to the trajectory of bonding for boys than girls. This indicates a general developmental difference in school bonding growth between boys and girls and as hypothesized, a more unfavorable trajectory of school bonding for boys. Gender differences, such as those found for other developmental processes (e.g., self-esteem development; Kling, Hyde, Showers, & Buswell, 1999) seem to exist for school bonding as well, suggesting that exploration of gender differences may be important for research on this construct.

In addition to gender differences, the results suggest that individual risk factors have a strong impact on the level of school bonding in the sixth grade and that these differences may persist over time. This importance of problem behavior in our models lends support for the Social Development Model (Catalano & Hawkins, 1996). Problem behavior may influence school bonding by increasing bonds to deviant peers. In our study, students engaging in substance use or deviant behavior as well as students with antisocial peers and low grades, have a lower level of school bonding at Grade 6 than youth without these risk factors. Because all children experience a decrease in school bonding over time, children with a lower level of school bonding in sixth grade continue to have a lower level of school bonding in eighth grade. Therefore, it may be critical to target school bonding early in development, prior to sixth grade. Strengthening school bonding for youth with deviant behavior may be particularly important, as deviant behavior was the strongest predictor of sixth grade levels of school bonding in our model.

Risk factors not only affected the level of school bonding in sixth grade; they also affected the trajectory of school bonding over the middle school period. Whereas deviant behavior is relatively important for the value of school bonding at sixth grade, increases in substance use and lower grades over time were associated with a steeper decline in school bonding over the middle school period. Therefore, attempts to strengthen school bonding may need to address youth with different problem behaviors at different time points. After sixth grade, it may be important to strengthen school bonding for students who are engaging in substance use and for those with academic problems.

Furthermore, the importance of grades to the trajectory of school bonding suggests it may be important to foster non-academic avenues to strengthen a sense of belonging at school for low achieving students. Frustration among students with poor grades may hamper their ability to form positive bonds with their schools. Within-school activities that are independent of grades such as arts and music activities, athletic activities, and school improvement projects that involve both children and teachers may be important to strengthen teacher-student relationship and school bonding, especially for students who struggle academically. It might be useful to consider interventions that target youth at the highest risk of becoming disconnected from school. Eggert and colleagues found evidence that a preventive intervention program that takes a social network support approach can lead to increases in school bonding and help reducing bonding to deviant peers, substance use, and related problems (e.g. Eggert, Thomas, Herting, Nicholas, & Dicker, 1994; Eggert, Thompson, Herting, & Randell, 2001). From our data, students who already engage problem behavior and have poorer grades are at the highest risk of having poor bonds to their schools during early adolescence. It is possible that programs targeting problem behaviors and/or academic achievement already exist in some schools in our sample, and these differences may explain the school-level differences in levels of school bonding in Grade 6.

In conclusion, to strengthen school bonding as a protective factor for youth (Catalano & Hawkins, 1996), two strategies might be used. First, it may be important to focus on reducing problem behavior, especially deviant behavior and substance use. Second, it may be important to increase within-school activities that do not focus solely on academics. However, it should be noted that even when modeling school bonding using multiple predictors, we did not fully explain the variance in the growth of school bonding. Thus, future research is needed to explore other influences on school bonding.

Limitations

This analysis used a carefully modeled measurement instrument of school bonding. Still, our findings should be considered in light of different measurement strategies between studies. Possible discrepancies between our results and other studies may be influenced by the use of different measurement instruments. Although our scale of school bonding has a high reliability and the measurement model fits the data very well, the validity of the construct is still uncertain. We used a model with varying loadings over different time points, which might make it difficult to separate changes in measurement from real developmental processes and changes. However, the lack of invariance in factor loadings may also indicate a real change in the meaning of school bonding conceptually. Our finding that some items of school bonding became relatively more important over time than others might indicate a changing definition of school bonding over time. Further research on this topic is needed.

Our model was limited to four occasions of measurement and analyzed school bonding from 6^th–8^th grade only. Additional time points may have resulted in a different growth curve. We used a quadratic growth curve model to approximate the true trajectory of school bonding. However, even with these limitations, our analysis suggests that the true trajectory of school bonding development is more complex than a simple decrease.

Further, it is likely that the relationship between school bonding and youth behavior is reciprocal (Maddox & Prinz, 2003; Roeser, Eccles, & Sameroff, 2000). As earlier research suggests, problem behavior predicts school bonding but school bonding also predicts problematic youth outcomes (Battistich & Hom, 1997; Hawkins, Guo, Hill, Battin-Pearson, & Abbott, 2001; Lonczak, Abbott, Hawkins, Kosterman, & Catalano, 2002; McBride et al., 1995). Youth bonds to their schools may influence the extent to which they feel bonded to antisocial peers; bonds to antisocial peers might in turn influence the extent to which children feel bonded to their school (Catalano & Hawkins, 1996). However, reciprocal influences are beyond the scope of this paper and we cannot identify the specific causal causal pathway between school bonding and behavior. Further research on reciprocal relationships is needed.

Furthermore, all measures are youth report, and may reflect a self-report bias. The model was based on a sample of youth in 28 rural and small town communities in the U.S. These results may not be generalizable to urban youth or those in other countries.

Conclusion

School bonding decreases over the middle school period as part of normative development. However, the trajectory of school bonding is more complex than a simple linear decrease. Results suggest that inter-individual differences between youth have a meaningful impact on intra-individual development. The initial level of school bonding at Grade 6 varies between schools and is influenced by student characteristics such as gender, problem behavior, and academic achievement. The growth of school bonding over time varies by gender in addition to changes in substance use and academic achievement. Thus, interventions to strengthen school bonding may need to focus on inter-individual differences between children, taking into account the powerful role of negative peer groups, child engagement in deviance and substance use, and academic achievement and targeting children most at risk for low levels of school bonding over the middle school period.

Biography

Jenny Oelsner is a research assistant in the Institute for Criminology at the University of Cologne, Germany. Her interests include the development of problematic and criminal behavior in early adolescence and the implementation and evaluation of preventive interventions.

Melissa Lippold is a Ph.D. Candidate in the Department of Human Development and Family Studies at the Pennsylvania State University. Her research interests include the development of problem behavior in early adolescence and the design and implementation of interventions for high risk youth and their families.

Mark T. Greenberg holds the Bennett Chair in Prevention Science in Human Development and Family Studies at Pennsylvania State University. He is also Director of the Prevention Research Center. His interests focus on risk and protective factors as well as interventions related to maladjustment and successful functioning in children and youth.