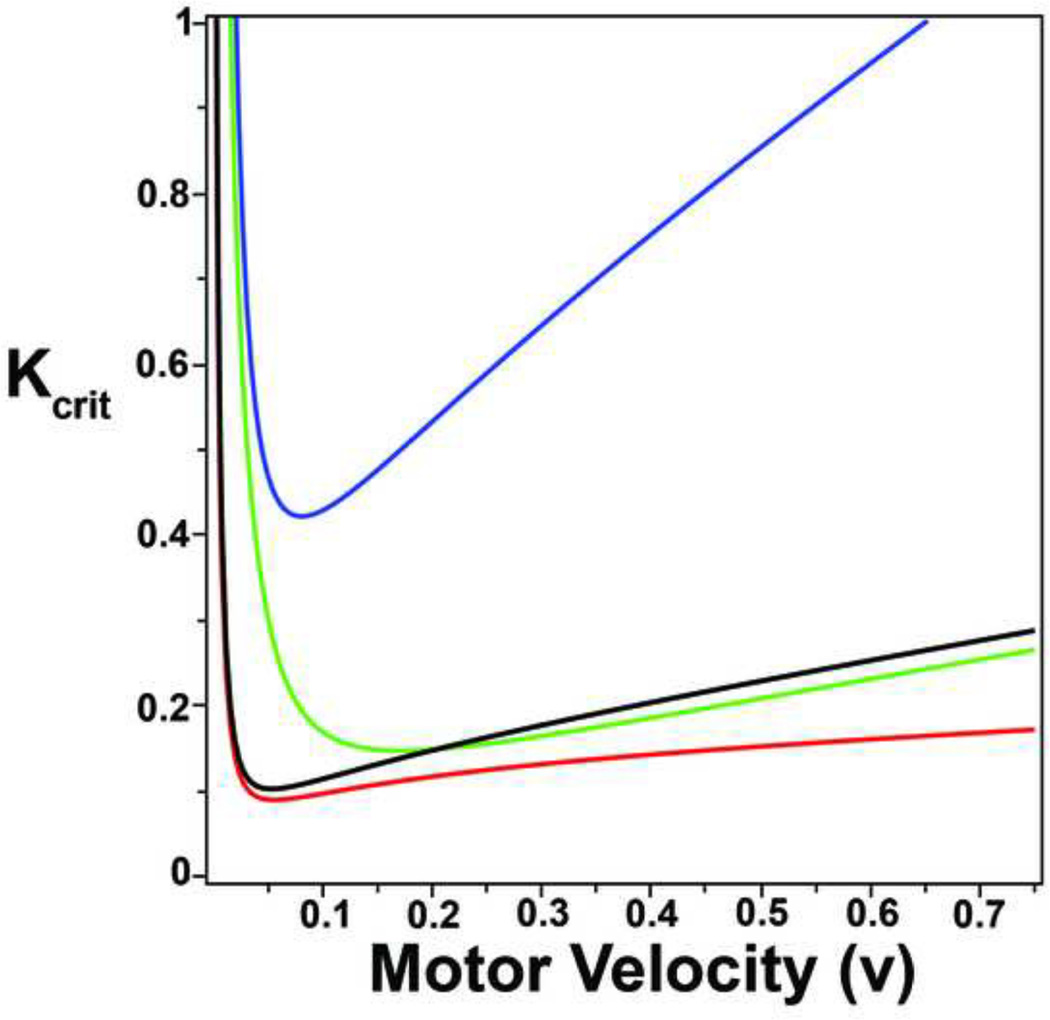
Figure D.1. Determining how K_crit is a function of motor velocity.

K_crit, shown in Equation 41 depends on all the variables of the model: motor velocity, and rates of attachment, detachment, and polymerization. The curves specifically show how K_crit depends on motor velocity, but also how it depends on the three variable rates. The blue curve shows a low rate of attachment (r₀=0.7), a low rate of detachment (r₁=0.1), and a high rate of polymerization (r₂=0.1). The rest of the curves are as follows: green (r₀=0.7 (low), r₁=0.4 (high), r₂=0.025 (low)), black (r₀=0.7 (low), r₁=0.1 (low), r₂=0.025 (low)), and red (r₀=2.8 (high), r₁=0.1 (low), r₂=0.025(low)). Notice that the minimum value of K_crit depends most on the sum of the rate of detachment and polymerization. The asymptotic approximation yields the range of motor velocities for which maximal alignment is expected.