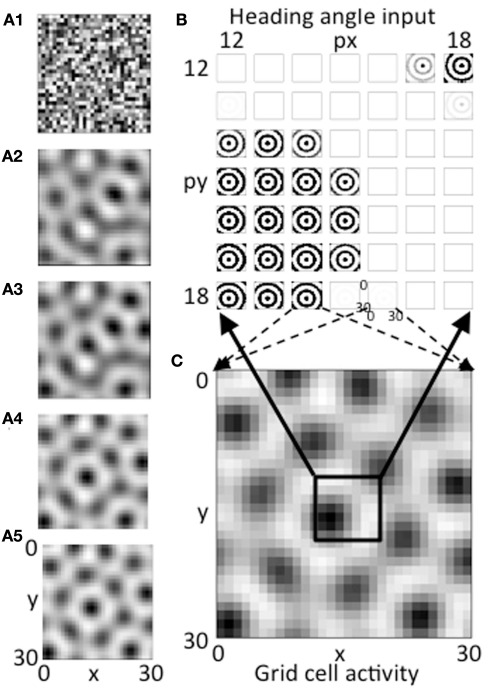
Figure 3.

(A1–A5) The evolution of activity in the grid cell plane at different stages during the iterative interaction. The network starts with random activity that then evolves into a hexagonal array of firing fields in a manner similar to continuous attractor dynamics models of grid cells. (B) Example of the magnitude of synaptic input at a single time step at the end of the simulation for a 7 by 7 set of heading angle cell arrays. The full simulation contains 30 spatial phases in the px dimension and 30 in the py dimension, but only a 7 by 7 array of spatial phases from the center is shown (phases 12–18). The input from heading angle cells at a specific spatial phase is determined by feedback from the grid cell plane. (C) The pattern of activity in the full grid cell plane with 30 by 30 spatial phases showing how feedback regulation of the pattern of summed activity from the heading angle plane produces a grid cell firing pattern that is stable. The pattern is stable because the pattern created by summing the 30 by 30 pattern of synaptic input from the 30 by 30 array of spatial phases of heading angles (B) matches the pattern of feedback activation of the heading angle array from active cells in the 30 by 30 array of grid cells (C).